1. Introduction

^{Rees (1978)} suggested that a time-variability in the ejection of an extragalactic jet would produce shock waves travelling down the jet beam. This idea was pursued by ^{Wilson (1984)}, who presented gasdynamical simulations of ejections with a periodical variability) and ^{Roberts (1986)}, who presented a momentum conserving model of a stochastic ejection). ^{Raga et al. (1990)} applied variable ejection jet models to outflows from young stars.

In the context of Herbig-Haro (HH) objects, variable jet models have proved to be quite successful, because images obtained with the Hubble Space Telescope (HST) in some cases show that knot chains along HH jets show structures similar to the “internal working surfaces” that result from ejection velocity variability. Comparisons between predictions from numerical simulations of variable jets and observations have proved to be quite successful at least for some HH objects (e.g., HH 211: ^{Völker et al. 1999}; HH 111: ^{Masciadri et al. 2002}; HH 444: ^{Raga et al. 2010}; HH 34: ^{Raga et al. 2012} HH 1: ^{Hansen et al. 2016}).

Theoretical work on variable HH jets includes both analytical models (e.g., ^{Raga & Kofman 1992}; ^{Smith et al. 1997}; ^{Cantó et al. 2000}) and numerical simulations (e.g., ^{Stone & Norman 1993}; ^{Raga & Biro 1993}; ^{Cerqueira & de Gouveia Dal Pino 2001}; ^{Hansen et al. 2015}); single- and multi-mode periodical ejections (e. g., ^{Raga & Noriega-Crespo 1998}; ^{Raga et al. 2015}), as well as stochastic ejections (^{Raga 1992}; ^{Yirak et al. 2009}, ²⁰¹²; ^{Bonito et al. 2010a}, ^b; ^{Raga & Noriega-Crespo 2013}; ^{Hansen et al. 2015}, ²⁰¹⁶).

Multi-mode, periodical ejection variabilities and stochastic ejections produce two-shock “internal working surfaces” which travel at different velocities along the jet beam. This results in “knot merging” events, in which a faster working surface catches up with a slower one, and both merge into a single working surface (travelling at an intermediate velocity).

A single-mode sinusoidal ejection velocity variability produces one working surface per ejection period. These successive working surfaces have almost identical time evolutions, so that catching-up events (between successive knots) do not occur. However, it is possible to construct single-mode ejection velocity variabilities which lead to the formation of two or more internal working surfaces per ejection period. These working surfaces do not necessarily have identical time evolutions, so that catching-up events can be produced.

In the present paper, we describe ejection velocity variabilities that lead to the formation of two working surfaces per ejection period. In § 2, we describe the formation of internal working surfaces by a periodic ejection velocity variability, derive criteria for the production of multiple working surfaces per ejection period, and describe possible ways to derive ejection variabilities leading to multiple working surfaces. In § 3, we explore the formation of pairs of internal working surfaces through an ejection velocity variability of the form u0∝sinnωτ, where u0 is the time-dependentent ejection velocity, n is an odd integer, τ is the ejection time, and ω is a constant. Finally, we summarize our results and discuss the implications for HH jets in § 4.

2. The formation of multiple working surfaces by a periodic variability

Let us consider a hypersonic, free-streaming jet with a time-dependent ejection velocity u0(τ) (given as a function of the ejection time τ). As shown by ^{Raga et al. (1990)}, if the ejection velocity increases with τ (i.e. u̇0=du0/dτ>0), the fluid parcels ejected at a time τ catch up with each other at a time

(see equation 4 of ^{Raga et al. 2015}). Therefore, the parts of the u0(τ) variability with u̇0>0 can in principle give rise to discontinuities (which correspond to two-shock “internal working surfaces”, see, e.g., ^{Kofman & Raga 1992}). However, the fluid parcels ejected at a time τ can hit an already existing internal working surface at a time t<tcol. Because of this, ^{Raga & Kofman (1992)} argued that the working surfaces corresponding to the successive ejection variability periods would initially be formed by the fluid parcels ejected at times τc that correspond to minima of the tcol(τ) function (see equation [tcol]). Therefore, the fluid parcels which lead to the initial formation of a working surface are ejected at times τc which are given by the roots of the equation

where care must be taken to see that the roots actually correspond to minima (rather than maxima) of tcol(τ).

For the well studied case of a sinusoidal ejection velocity variability, each period has a single root (see equation [tauc]), and a single working surface is formed per ejection variability period (this problem having a full analytic solution, see ^{Cantó et al. 2000}). The successive working surfaces (one per ejection period) are formed at times:

(obtained setting τ=τc in equation [tauc]) and at a distance

from the outflow source (see equations 7-8 of ^{Raga et al. 2015}).

It is possible to construct functional forms for u0(τ) that produce two or more roots for equation ([tauc]) per ejection period. Let us assume that we have two roots τc1<τc2. The fluid parcels ejected around τc,1 will produce a working surface at a time tc,1 and position xc,1 (obtained from equations [timec]-[xc]). The parcels ejected around τc,2 ( >τc,1, see above) will catch up with each other at a later time tc,2, at a distance xc,2 from the source (see equations [timec]-[xc]).

Clearly, if

the second working surface will indeed be formed before the fluid parcels ejected at time τ2 hit the first working surface (produced by the parcels ejected at time τ1). Therefore, if we have a periodic ejection velocity variability u0(τ) with two τc roots of equation ([tauc]) per ejection period satisfying condition ([xc2]), two internal working surfaces (per period) will be formed. These internal working surfaces will in principle have different equations of motion (resulting in different velocities along the jet flow), so that catching up processes between successive working surfaces will occur farther along the jet beam.

Of course, we could have variabilities which do not satisfy condition ([xc2]) but still lead to the formation of working surface pairs. This is due to the fact that at the time t=tc,2 (in which the second working surface is formed) the first working surface will have moved down the jet flow from the position xc,1 at which it was formed, to a position x1(tc,2). Therefore, the second working surface would still be formed provided that

In order to apply this condition, however, one needs to solve the equation of motion for the first working surface (in order to calculate its position at time tc,2). In the present paper, we will use the simpler criterion given by equation ([xc2]) (which is a sufficient but not necessary condition for the formation of working surface pairs), and apply it to study a family of periodic functional forms for u0(τ) (see § 3).

We end this discussion by noting that it is possible to construct forms for u0(τ) which lead to the formation of two or more internal working surfaces per ejection period in the following way. In Figure 1, we show an ejection velocity variability with two steps (at ejection times τ1 and τ2) of increasing velocities per ejection period p. This variability gives two roots of equation ([tauc]) which are τ1 and τ2. Each of these velocity jumps produces a working surface at the ejection point (i.e., xc,2=xc,1, so that condition [xc2] is satisfied). Therefore, two working surfaces are produced per ejection period for the velocity variability shown in Figure 1. At later times, the faster, second working surface eventually catches up with the first working surface produced by each ejection variability period.

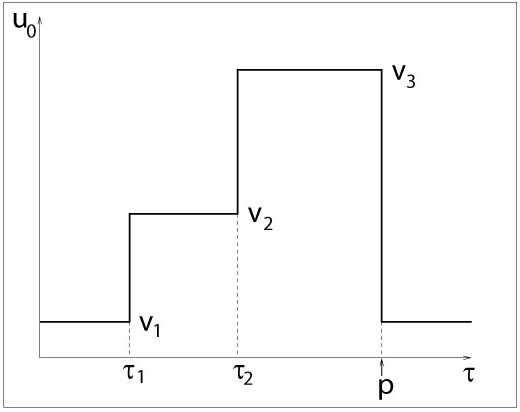
Fig. 1 Schematic diagram of a “double box-car” ejection velocity variability. Within the ejection period p, the velocity has an initial value v1, then jumps to v2, to v3 and back to v1. The properties of the resulting flow are described in § 2. 

The ejection variability shown in Figure 1 can be used as a model for obtaining continuous forms of u0(τ) which produce two or more working surfaces per ejection period. An example of this is explored in the following section.

It is of course possible to construct a periodic ejection variability that produces two working surfaces, with the second working surface being slower than the first one. In this case, one would obtain interactions between working surfaces produced in contiguous ejection variability periods. However, by appropriately shifting the starting times of the ejection variability periods, the problem can be converted into the “slow first working surface/fast second working surface” case described above.

3. Odd powers of a sine wave

3.1 General considerations

We consider an ejection velocity variability of the form:

u0(τ)=v0+v1sinnωτ , (7)

where u0 is the ejection velocity (as a function of the ejection time τ), n is an odd integer, and v0, v1 and ω are constants.

In Figure 2, we show the first period of sinnωτ for n=1, 3 and 5. One sees that starting from n=3, this function has two steep rises (as a function of increasing ωτ) per period p=2π/ω. In the following subsections, we show that these two rises result in the production of a pair of internal working surfaces per ejection period.

Fig. 2 This graph shows a single period of the functions sinωτ (solid line), sin3ωτ (dashed line) and sin5ωτ (dotted line). 

We calculate the ejection times τc which give rise to the fluid parcels which eventually form internal working surfaces as the roots of equation (2). Combining equations (7) and (2) we obtain that the ejection times τc giving rise to working surfaces for a sinnωτ variability are given by the roots of:

λsin2ωτc-2-sinωτc=0 , (8)

for n=1, and

λnsinn+2ωτc-(n+1)sinnωτc

-nsin2ωτc+n-1=0 , (9)

for n=3, 5, …, where λ≡v1/v0. The roots for the n=1 case (equation [tc1]) are described in § 3.2, and approximate solutions for the n≥3 case are presented in § 3.3.

4. Numerical solutions for the n= 3 case

In order to illustrate the characteristics of a jet with “double working surfaces”, we have obtained a numerical solution of Burgers’ equation:

subject to an inflow condition

at x=0 (the outer boundary of the simulation being a free outflow). This variability corresponds to equation (7) with n=3, v0=1, v1=0.1 and ω=1.

Burgers’ equation corresponds to a pressure-less flow, and has discontinuities that correspond to two-shock working surfaces in the Euler equations. The equation of motion of the discontinuities of Burgers’ equation corresponds to a ram-pressure balance working surface in a constant density flow (see, e.g., ^{Raga et al. 1990} and ^{Raga & Kofman 1992}), and therefore generally does not agree with the motion of a working surface modeled with the gas-dynamic (Euler) equations. However, solutions of Burgers’ equation do illustrate the general properties of the corresponding gasdynamic problems (in the case of hypersonic flows).

We first use equations (16) and (17) to obtain estimates for the distances from the source at which the working surfaces are formed. With the parameters of the chosen ejection velocity variability (see equation [infl]), we obtain a position x1=7.86 and x2=9.72. We can compare the results of the numerical simulation with these predictions.

The velocity structure resulting from the numerical simulations is shown in Figure 3 for different integration times. It is clear that two discontinuities (corresponding to internal working surfaces) are formed per ejection period. In the t=36 velocity structure (first frame of Figure 3), we see that the first discontinuity (from one of the ejection periods) has been formed at a position x≈7.5, and in the t=40 structure (bottom frame of Figure 3), we see the second discontinuity forming at x≈9. These values are in approximate agreement with the predictions from the analytic model (see above).

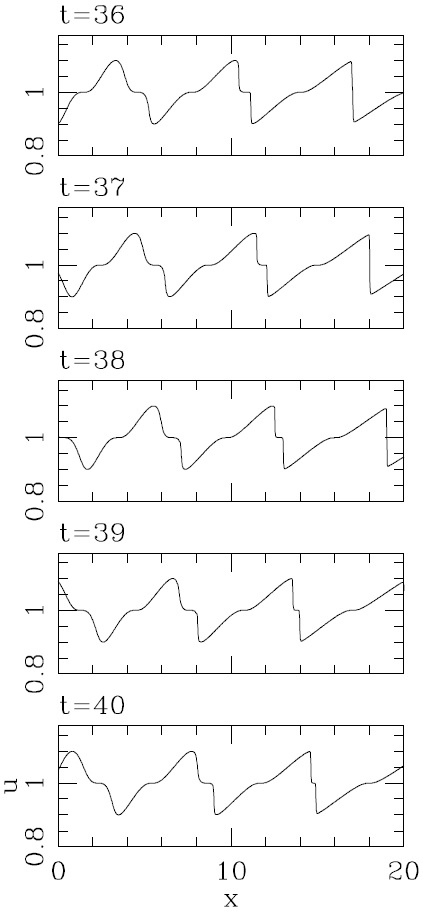
Fig. 3 This graph shows the velocity vs. position obtained at different times from a numerical solution of Burgers’s equation with an ejection velocity (at x=0) of the form u0=1+0.1sin3t. The development of double working surfaces, and the subsequent catching-ups between the working surface pairs are clearly seen. 

In the t=40 velocity structure (bottom frame of Figure 3), we also see that the two working surfaces produced by one of the ejection periods (at a position x≈14) are about to merge. At larger distances from the source, we see discontinuities that correspond to merged pairs of working surfaces (see the last discontinuity of the t=36, 37 and 38 velocity structures in Figure 3).

5. Summary

We have explored the possible formation of multiple working surfaces in jets per ejection period of a periodic ejection velocity variability. Given the functional form of a proposed ejection velocity variability, it is clear that it produces double working surfaces if it has two roots of equation (2) which satisfy the criterion of equation (5). The ejection variability will also produce double working surfaces (per ejection period) if the roots satisfy the less stringent criterion of equation (6), but this criterion is of less straightforward application because it requires solving the equation of motion for the faster working surface (see § 2).

We have then studied a variability of the form u0(τ)=v0+v1sinnωτ (with odd n, see § 3), and found that for n≥3 one obtains two roots of equation (2), and that these two roots asymptotically approach the criterion of equation (5) for small values of λ=υ1/ν0. We derived approximate analytic expressions for these roots and for the distances from the source at which the resulting working surfaces are formed.

This “small λ” approximation is in principle valid for some of the knot structures observed along HH jets. Examples of this are the chains of aligned knots observed at distances of ≈1017cm from the source in HH 34 and HH 111, which for an interpretation in terms of a variable jet model require amplitudes of ≈10 % of the mean velocity of the ejection (see Raga et al. 2002). As shown in § 4, for λ=0.1 the analytic, small λ solution (described in § 3.2 and 3.3) already gives good results.

We finally presented a numerical solution of Burgers’s equation for a one-dimensional flow with an ejection velocity variability of the form u0τ=1+0.1 sin3ωτ. In this flow, we saw that two working surfaces are formed per ejection period, at the distances from the source predicted from the analytic model.

In this numerical example, the two working surfaces that were produced have different velocities, and therefore the faster working surface catches up with the slower working surface as they travel away from the source. The position at which this catching up process occurred was approximately twice the distance from the source at which the working surfaces were formed. At larger distances from the source, one has single working surfaces (produced through the merger of the double working surfaces resulting from the ejection) per ejection velocity variability period.

This characteristic is a general feature of double working surfaces produced by periodic ejection variabilities: they only remain double working surfaces for a certain time, and eventually merge into one, as the faster working surfaces catch up with the slower ones. Therefore, one would expect to see a qualitative difference between the knot structures at larger distances from the source (where we see merged knots) and close to the point of working surface formation (where we see the double working surfaces). Such an effect has been described by ^{Raga & Noriega-Crespo (2013)} for the HH 34 jet, but no comparable studies exist for other HH objects.

Because of this lack of clear application, our present work should be regarded as a mathematical curiosity relating to the formation of shock structures in periodically variable jets. The method which we have described for evaluating the possible formation of double working surfaces (per ejection period) might be useful for choosing appropriate forms for an ejection velocity when trying to simulate specific HH jets.

An interesting question is whether or not the analytic approach used in this paper is applicable to the case of magnetized jets. The criterion for working surface formation (see § 2) is valid for a ballistic flow. In the case of a magnetized jet, a ballistic regime is obtained if the flow is hypersonic and also “hyperalfvénic” (i.e., with a jet velocity much larger than the Alfvén velocity). Therefore, under these conditions the analysis presented above should also be valid. However, implicit in the discussion of the subsequent evolution of the working surfaces (involving “catching up” events) is the assumption that they have a small spatial extent along the outflow axis (i.e., that the two shocks in the working surfaces have relatively small separations). This is not necessarily the case for magnetized jets, which (depending on the radial configuration of the magnetic field) can have internal working surfaces with substantial axial extents (see, e.g., ^{De Colle et al. 2008} and Hansen et al. 2015). If this axial stretching is large enough, it can also lead to mergings between successive working surfaces. This effect (which is nicely illustrated in Figure 2 of Hansen et al. 2015 for the case of axisymmetric, magnetized jets) was studied (for the case of 1D, non-magnetized flows, which also develop axially extended working surfaces) by ^{Smith et al. (1997)}. Clearly, variable jets with axially extended, double working surfaces (which could be obtained with one of the ejection variabilities discussed above, together with an appropriate magnetic field configuration) will have a richness of behaviour not appropriately described by the simple, “catching up” description which we have used in the present paper.