1. INTRODUCTION
Tony Thirlwall considers Roy Harrod (1900-1978) one of the greatest economists of the 20th century and second, in originality, to Keynes among British economists, surpassing Hicks, Meade, Kaldor and Joan Robinson2. Harrod’s main innovations which include the foreign trade multiplier, his approach to economic dynamics, and the fundamental growth equation are a major point of reference in Thirlwall’s writings and a source of influence underlying his most important contributions to economics, found in the fields of international economics, growth theory, regional and development economics3.
Along with Kaldor, Thirlwall recognized the importance and superior analytical power of Harrod’s foreign trade multiplier over the Keynesian investment multiplier (and certainly Neoclassical growth theory) and as the principle explaining the differences in the growth performance of countries. It also acts as the mechanism working through income rather than substitution effects, to equilibrate countries trade balances maintaining the consistency with Keynes’s principle of effective demand.
The analysis of the impact of the balance of payments on countries’ economic performance led Thirlwall to formulate the dynamic analogue to Harrod’s foreign trade multiplier known as Thirlwall’s law. Its empirical validation across a variety of developed and developing countries historical experiences exemplifies the durability of Harrod’s foreign trade multiplier analysis.
Thirlwall has underscored the generality of Harrod’s dynamic foreign trade multiplier by extending its use to explain interregional growth rate differences and address the problem of regional convergence/divergence. Thirlwall has also provided greater consistency to Harrod’s dynamics by integrating the balance-of-payments equilibrium growth rate with Harrod’s warranted and natural growth rates. Harrod’s equations were derived within the context of a closed economy. The different scenarios considered provide a useful parallel to those identified by Harrod to illustrate the policy conflicts that can arise when using expansionary policies to equilibrate the warranted, natural, and actual growth rates4.
Abstracting from external sector considerations, Thirlwall has shown that the relation between the natural and warranted rates of growth is a ‘useful pedagogic device’5 for understanding the policy choices available to less developed countries characterized by a growth of the labor force and productivity exceeding the rate of capital accumulation. In Harrodian terms in less developed economies the natural rate of growth exceeds the warranted rate of growth.
The deep concern that both Harrod and Thirlwall share regarding the social and economic problems faced by developing economies is also visible, within an open economy setting, in identifying the balance-of-payments constraint as a major obstacle to the improvement in their living standards. Both authors trace the external imbalance to the differences between countries’ income elasticity of demand for their imports and for their exports. The diversification of exports or structural change, using Thirlwall’s expression, is the solution to overcome this predicament6.
2. THE ANALOGY BETWEEN HARROD’S FOREIGN TRADE MULTIPLIER AND THIRLWALL’S LAW
A central theme that has guided Thirlwall’s research and approach to economics is the analysis of how the balance of payments impinges on the growth performance of countries. The inclusion of the open economy in the analysis of growth and more precisely the recognition that countries must achieve, in the long-run, a trade/current account balance led Thirlwall to develop his balance-of-payments constrained growth model and, under simplifying conditions, to formulate Thirlwall’s law.
Thirlwall’s law states that the long-run rate of growth of an economy compatible with balance-of-payments equilibrium (ybpc ) can be approximated by the product of the rate of growth of the rest of the world (yrw ) and the ratio of the income elasticity of the demand for exports (ε) to the income elasticity of the demand for imports (π), or by the simple rule of the rate of growth of exports (x) divided by the income elasticity of demand for imports (equation [1]):
Where ε, π > o and x = εyrw .
Thirlwall’s law is the dynamic analogue of Harrod’s foreign trade multiplier:
Where Y, X and m are income/output, exports, and the average propensity to import, which is a static concept. Starting with equation [2] the dynamic Harrod multiplier can be obtained as follows7. Equation [2] gives rise to the expression:
Multiplying the left-hand side of equation [5] by Y/X and the right-hand side by Y/M:
This analogy between both means that the Harrod trade multiplier works in a growth context. According to both equations [1] and [2] growth is driven through a multiplier process driven by autonomous demand. Within an open economy context, exports are the only true component of autonomous demand in the sense of demand emanating from outside the system and also the only component of demand that can pay for the import requirements for growth (Kaldor, 1989 [1981]).
In the static and dynamic versions of the foreign trade multiplier income effects predominate over substitution effects. Variations in income provide the mechanism that brings about the adjustment between internal and external economic conditions. For a given ratio of income elasticities, an increase in the rate of growth of the rest of the world translates into a rise in exports over imports and thus generates the space for the expansion of aggregate demand. The consequent increase in income and thus imports restore the balance of payments equilibrium.
Relative prices play no role in the adjustment process8. Thirlwall’s law assumes the near constancy of real exchange rates as variations in internal and prices adjust to those of nominal exchange rates. Adjustment is also prevented by the existence of price setting behavior and wage bargaining clauses.
3. THE ORIGINS, DEVELOPMENT, ECLIPSE AND REVIVAL OF HARROD’S FOREIGN TRADE MULTIPLIER9
Harrod first formulated the foreign trade multiplier in the first edition of International Economics (Volume VIII of the Cambridge Handbook series). Keynes as editor of the Cambridge Economic Handbooks commissioned the book to Harrod. The derivation of the foreign trade multiplier was prompted by a critique of the law of comparative costs which did not take into account the relationship between trade and employment and, more to the point, the conditions under which an open economy is able to maintain full employment. In the second edition of International Economics, published in 1939, Harrod provided a full-fledged statement of the foreign trade multiplier process and its incorporation into Keynes’ theory of effective demand.
As he put it: “In the 1939 edition I set out (in Chapter 6) what I believe to have been the first statement of the doctrine of the ‘foreign trade multiplier’, which is now commonly accepted.” (Harrod, 1957 [1933], p. ix). In Towards a Dynamic Economics (1948) he made a similar statement: “When it fell to me to produce a revised edition (which appeared in 1939) of my handbook on International Economics, I resolved to endeavor to grapple manfully with the problem; I think I succeeded in producing something which gave a systematic account of the relation between the Keynesian theory of unemployment and balance-of-trade theory.” (Harrod, 1948, p. 101).
In fact, in later editions of the book he stated that the purpose of the book was to provide an explanation of the traditional theory of international trade modified to take into account Keynes’s and Keynes’s followers contribution to economics (Harrod, 1957 [1933], p. 158). While Harrod was convinced that he filled a gap in Keynes’s thought, Keynes himself was not completely convinced about the foreign trade multiplier but did not raise ‘fundamental objections’ to its formulation.
Following Harrod (1941 [1933]) , pp. 119-122), the foreign multiplier can be formally stated as follows. Assume an initial situation where all payments are made only with respect to tradeable goods and services, no foreign or domestic investment, and no savings (individuals spend all their income). Further assume that total income (Y) is derived from the sale of goods by residents (Cd ) and non-residents (exports, X) and that expenditures are equal to total consumption (C) which is divided into the consumption of domestic goods (cY) and imported goods (mY). Formally,
Where c, m are the proportion of income spent on domestic goods and foreign goods.
Given income equals expenditure, and that the income derived from the goods purchased by domestic residents is equal to their income, then exports are equal to imports.
Exports depend on two factors: 1) domestic production costs; 2) world prices and external demand. Assuming a gold standard regime in place, that the costs of production and world prices and that the proportion of income spent on foreign goods (m) is known, the value of income (Y) can be determined as follows:
Treatments of the multiplier preceding Harrod’s 1939 full-fledged treatment of the multiplier referenced in the literature include Giblin (1930), Warming (1932), Kalecki (1934 [1971]), Clark (1938), and Paish (1936)10. Prior to all of these, Thirlwall (1982, p. 3, note 1) includes the Danish parliamentarian Julius Wulff (1896)11.
By 1941, the foreign trade multiplier was a well-established concept in the literature (Haberler, 1952 [1937])12. As explained by Harrod (1969, p. 221): “The essentials of the income (‘multiplier’) theory of the foreign balance of trade were fairly well understood by the time of the Second World War.”13
Still, according to Thirlwall, echoing Kaldor’s views (who is largely responsible for reviving the idea and significance of Harrod’s foreign trade multiplier)14, Harrod’s foreign trade multiplier “was eclipsed by Keynes’s investment multiplier.”15 This, despite the fact, that Harrod’s foreign trade multiplier provided a more adequate mechanism to explain growth and the different rates of growth among countries as well as of the fluctuations of their business cycle16. As explained by Kaldor (1975, p. 354):
In some ways I think it may have been very unfortunate that the very success of Keynes’s ideas in explaining unemployment in a depression -essentially for short period analysis- diverted attention from the foreign trade multiplier which over longer periods is a far more important principle for explaining the growth and rhythm of industrial development. For over longer periods, Ricardo’s presumption that manufacturers and traders only save in order to invest, so that the amount and/or the proportion of savings would adapt to change in the opportunities for, or profitability of, investment, seems to me more relevant than the Keynesian assumption for explaining the true constraints on the growth of production and employment in the ‘capitalist’ industrial sector.
Kaldor became convinced of the validity of the Harrod foreign trade multiplier and of the dominance of income elasticities over price elasticities of demand in the 1970s when he became aware that, within a floating exchange rate regime, nominal exchange rate adjustment would not bring sufficiently large changes in real exchange rates or that prices elasticities were not large enough in the short run to bring about a balance of payment equilibrium17. In his paper ‘The Effect of Devaluations on Trade in Manufactures’ (1977), Kaldor found a positive relationship between an increase (decrease) in relative unit costs, appreciating (depreciating) exchange rates and higher (lower) export shares for selected developed countries.
In the same year, he published an article in The Times criticizing the views of two British economists that argued that, at the time, the British economy did not have a balance of payments problem as the autonomous increase in the share of exports in income had kept pace with the rise in the of imports in income. Following the logic of the foreign trade multiplier, Kaldor argued that a rise in imports (and thus of the import share in income) reduced income and as a result the share of exports in income increased18.
Thirlwall began corresponding with Kaldor, albeit irregularly in 1975 when, with Robert Dixon he formalized Kaldor’s theory of growth and regional growth differences as a cumulative disequilibrium process found in ‘A Model of Regional Growth Rate Differences on Kaldorian Lines’19. Later on, as Thirlwall explains their “correspondence blossomed when quite independently they both became interested in Harrod’s foreign trade multiplier.”20
Thirlwall’s initial analyses of Harrod’s foreign trade multiplier were not related to the problem of growth in an open economy21. And when Thirlwall presented for the first time the balance-of-payments constraint (and what became known as Thirlwall’s law) as an explanation of the differences in the growth rates of countries, he made no reference to Harrod’s foreign trade multiplier22. As he explains (Thirlwall, 1987b, p. xvi) the end result of the article “turned out to be the dynamic analogue of the static Harrod trade multiplier, although I did not realize at the time. It was only when Kaldor wrote a letter to the Times on the subject that my colleague Charles Kennedy referred me to Harrod’s International Economics (1933) and I realized that I had reinvented the wheel.” Thirlwall’s seminal 1979 article included an empirical application of Thirlwall’s law only for developed economies. Later the empirical estimations were also expanded with success to the case of developing economies (Thirlwall, 1982; McCombie and Thirlwall, 2004; Soukiazis and Cerqueira, 2012; Pérez Caldentey and Vernengo, 2019).
4. EXTENDING HARROD’S FOREIGN TRADE MULTIPLIER TO THE REGIONAL CONTEXT
Thirlwall extended the applicability of the foreign trade multiplier to encompass the analysis of regional growth and development and of interregional growth rate differences. In his paper ‘Regional Problems are “Balance-of-Payment” Problems’ (Thirlwall, 1980), he argued that within a regional context slow growth and unemployment could be explained by balance-of-payments problems. As with the case of a national economy, regional growth is determined by the propensity to export relative to that of imports23.
The view that regional growth is demand constrained contrasts sharply with the traditional supply side based Neoclassical theory which determines the steady state rate of growth of regional output by the rate of growth of the labor force and productivity which are given exogenously and independent of demand. The Neoclassical theory attributes deviations from a supply-demand determined equilibrium to market imperfections. Unemployment is due to real wages that are too high to clear the labor market. Also, balance-of-payments difficulties are explained as real exchange being too high relative to its equilibrium level. Similarly, differences in regional productivity growth are explained by the misallocation of resources. The free flow of goods and factors of production between regions equilibrate the disparities in unemployment, productivity, growth, as well as balance-of-payments positions through changes in relative prices which lead to a Pareto optimal allocation of resources24.
Thirlwall replaces this approach with a ‘model in the Keynesian spirit,’ based on the interaction of two Harrodian notions, the foreign trade multiplier and the natural rate of growth combined with Verdoorn’s law. In the model long-term growth is demand driven by exports under a balance-of-payments constraint. As explained by Thirlwall (1980): “Regional growth is demand determined for the obvious reason that no region’s growth rate can be constrained by supply when factors of production are freely mobile.” Supply is endogenous adapting to demand, and income effects predominate over substitution effects, income providing the main adjustment mechanism between exports and imports.
The model determines first output growth via the dynamic foreign trade multiplier (Thirlwall’s law). The actual rate of growth (yt) is determined by the balance-of-payments equilibrium growth rate. That is:
Where ybpg, xt, π are the balance-of-payments equilibrium growth rate, the rate of growth of real exports and the income elasticity of the demand for imports. If the balance-of-payments equilibrium rate of growth equals the Harrodian natural rate of growth (ynt = lt + pt, where ynt, lt, pt are the natural rate of growth, the rate of growth of employment and productivity respectively), the rate of growth of employment can be expressed as a function of Thirlwall’s law and the rate of growth of productivity. This assumes that the direction of causation runs from the balance-of-payments equilibrium growth rate to the natural rate (Thirlwall, 2018, 2019). Formally:
Following Verdoorn’s law the rate of productivity growth is a function of output growth:
Where pat is autonomous productivity growth and λ is the Verdoorn coefficient (0 < λ < 1)25.
By substituting equation [13] in equation [12], the rate of growth of employment is expressed as a function of Thirlwall’s law, the Verdoorn coefficient and autonomous productivity:
The behavior of unemployment then depends on the difference between the rate of growth of labor supply and the rate of growth of employment (nt). Thirlwall (1980) specifies the rate of growth of labor supply as a function of the indigenous labor supply (nat), the elasticity of the labor supply from outside the region with respect to demand (ρ), the difference between the regional rate of unemployment and the rate outside the region (urt - ut), and the sensitivity of migration to unemployment rate differences (μ):
The change in the unemployment rate can be approximated by the difference between equations [14] and [13]:
For a given rate of growth of output determined by the balance-of-payments constraint, unemployment will depend on the indigenous rate of growth of the labor supply (nat), on autonomous productivity (pat), the elasticity of the labor supply from outside the region with respect to demand (ρ), and the sensitivity of migration to unemployment rate differences (μ).
Thirlwall argues that under conditions of growing unemployment policy has little space to modify these variables and parameters. As a result, policy must focus on expanding the balance-of-payments equilibrium growth rate through the increase in x and/or the reduction in π. It is in this sense the Harrod’s foreign trade multiplier in its dynamic version is applicable to the explanation of regional growth and unemployment rates.
5. HARROD’S DYNAMIC THEORY AND OPEN ECONOMY: FROM THE TRADE CYCLE (1936) TO TOWARDS A DYNAMIC ECONOMICS (1948)
Harrod dedicated an important part of his work and effort to the analysis of the problems of foreign trade and the open economy. However, these were never central, but tangential, to his most renowned contribution to economics, dynamic theory which was developed within a closed economy setting. In spite of having invented the foreign trade multiplier he never recognized its dynamic version and its implications for growth theory26. This is the main critique levelled by Thirlwall against Harrod27. The following two sections expand on the relation between Harrod’s dynamic theory and the open economy.
The first articulated version of Harrod’s dynamics, The Trade Cycle (Harrod, 1936), is presented mainly within a closed economy context. Considerations relating to the open economy are spelled out in one section (The foreign balance) found before the last chapter of the book28. Harrod defends the closed economy assumption based on the observed synchronicity in the trade cycle across different countries. At the same time, it is that very same synchronicity that requires the inclusion of different countries’ specificities in the analysis of the trade cycle. As he explained (Ibid., p. 145):
The argument so far has been concerned with a ‘closed system, not subject to the extraneous influence of a balance of payments with an outside world. It applies therefore to the world as a whole. Manifestations of the cycle have recently been world-wide; the depressions of 1920-2 and 1929-32 were so; recovery was proceeding at varying rates in all countries in the years 1925-9, and, despite the great variety of circumstances and policies in different countries, there was a remarkable synchronization, with but a few exceptions, in the recovery of 1933. Thus, it appears by no means out of accord with the facts to regard the world as a single unit for these purposes. None the less there are national differences. If each nation was entirely isolated from the rest, it is improbable that the observed synchronization would occur. It is important, therefore, to consider how the world phase of the cycle transmits its influence to each particular country.
The section on the foreign balance follows the line of thought of his book International Economics (1933). Harrod argues that in an open economy there is only one multiplier which depends on the proportion of income spent on foreign goods and services:
It is necessary to examine the relation of the savings multiplier to the foreign trade multiplier. At first sight it might appear that there is a conflict of principle; but this is not really so. Both the active current items in the foreign balance (exports and net income from foreign investment) and the volume of net investment at home serve as bases which if appropriately multiplied determine the level of total activity. And there are not two multipliers, but one. The multiplicand is the value of the active current items and the value of net investment at home taken together; and the multiplier depends on the proportion of income which people choose to spend on home-made goods. It is constituted by the ratio of their total income to their total income less what they spend on home-made goods29.
Harrod relates the foreign trade multiplier to dynamic analysis the proper question of which is to determine the conditions under which a society can maintain what Harrod would call later in his “An Essay on Dynamic Theory” (1939b), the warranted rate of growth. That is, “what is the rate of growth which, if maintained, will leave the parties content to continue behaving in a way consistent with it?”30
For Harrod, the foreign balance is determined by dynamic forces through the operation of the foreign trade multiplier. In an open economy, the way in which an increase in expenditure is divided between home-made and foreign-made goods, and, also, the increase in the current active items of the balance-of-payments (income derived from exports of goods and services and from foreign investment) are determinants of the steady advance which requires full employment of capital goods31. As explained by Thirlwall (2001, p. 83), Harrod does not relate the foreign trade multiplier to the balance-of-payments equilibrium growth rate. Rather he argues that current account balance is not necessary to maintain the steady advance. As long as financial inflows are positive “there is no obstacle on the monetary side to a given steady state.”32
As in the case of The Trade Cycle, the foreign sector is not central to the argument found in the “An Essay on Dynamic Theory” (1938, 1939b) and in the analysis of the foundation of Harrod’s dynamics, the instability principle which is derived for a closed economy. The introduction of the external sector is an add-on, a pure extension to his fundamental equation. The foreign sector enters into the warranted rate growth (GW ) through the introduction of foreign savings (the difference between imports and exports):
Where s is the propensity to save,
The foreign sector has a role to play in Harrod’s dynamics and the ensuing trade cycle only under disequilibrium conditions. The warranted rate of growth (GW) varies positively with a trade deficit (inflow of foreign savings) and negatively with a trade surplus (export of savings) and this sets the stage for the divergence between the warranted and the actual rate of growth. Due to the instability principle, the same factors that affect the warranted rate of growth in one direction (including changes in the parameters) have the opposite effect on the actual rate of growth. And disequilibrium conditions in the external sector will have an equilibrating role between the warranted and the actual growth rate only if the actual rate is above the warranted rate. It is worth to quote Harrod at length on this central point of his dynamics:
It is essential… to grasp the point that a change in the fundamental conditions tends to have the opposite effect on the actual rate to that which it has on the warranted rate. An increasing propensity to save, a decreasing capital coefficient… and a declining active balance or growing passive balance on the international current account, all tend to increase the warranted rate; but they all tend to have a depressing effect on the actual rate. If the actual rate is equal to the warranted rate, by raising the warranted rate above it, they precipitate a downward movement. If the actual rate is above the warranted rate, by raising the warranted rate, they reduce the gap and so diminish the drive to expansion. If the actual rate is below the warranted rate they increase the gap and so increase the forces of depression. These propositions are all connected with the instability of the moving equilibrium. In the ‘field’ on either side of the warranted line of growth, there are cenrifugal forces, which increase with the distance of the actual output from the warranted output. (Harrod, 1938, p. 1203).
The warranted rate of growth and thus its divergence from the actual is independent of considerations relating to the open economy if the balance of trade is in equilibrium (m = X/Y). Harrod’s depiction of dynamics provides no space for the inclusion of a balance-of-payments (trade) equilibrium growth rate.
Nonetheless, from the above citation a parallel can be drawn between Harrod’s foreign trade multiplier in its static and dynamic (Thirlwall’s law) versions and the dynamics in the upward phase of the trade cycle resulting from the interaction of the actual, warranted, and natural (“the maximum rate of growth allowed by the increase in population, accumulation of capital, technological improvement and the work/leisure preferences schedule, supposing that there is full employment in some sense”33) rates of growth. An increase in the import ratio is accompanied by a lower level of output in the static version of the foreign trade multiplier) and by a lower rate of growth of output in the dynamic version of the foreign trade multiplier. The latter can also be shown to be one of the results of the workings of the fundamental equations with the foreign balance.
Harrod used this same logic to argue in Towards a Dynamic Economics (1948, pp. 105-106) that when the warranted rate of growth exceeds the natural rate of growth (GW > Gn) a positive trade balance (X/Y > m) is beneficial if there is a ‘runaway of actual growth downwards from the warranted rate of growth’34. In Towards a Dynamic Economics (1948) Harrod made explicit the divorce between the balance-of-payments position and the volume of exports: “… It should be the absolute volume of exports, and not the balance of trade, that is important… In my system the increase in the volume of exports would be rightly found to have a stimulating effect on employment, even although, through consequential reactions in home investment, no addition to the balance of trade ensued.”35
6. ECONOMIC DYNAMICS (1973) AND THE INTRODUCTION OF THE BALANCE-OF-PAYMENTS EQUILIBRIUM GROWTH RATES INTO HARROD’S THEORY OF DYNAMICS
In his last full-fledged treatment of economic dynamics (Economic Dynamics, 1973), which is a rewrite of Towards a Dynamic Economics (Harrod, 1973, p. vii) Harrod raises two questions related to the adjustment required to equilibrate the external balance. The first is the Keynesian criticism, namely the detrimental effects on output and employment as a product of a restrictive monetary policy. The second relates to whether price elasticities of demand are sufficiently high to fulfill the Marshall-Lerner conditions (“… that the sum of the price elasticity of foreign demand for home goods and of the price elasticity of the home demand for foreign goods is greater than one”)36.
It is in connection with the first question that Harrod brings to the fore the impact of the external balance on the problems faced by monetary management and the conflicts that can arise in relation to the various positions of the three growth rates (actual, warranted, and natural growth rates, G, GW, Gn) which he had discussed earlier in Economic Dynamics but without reference to the external sector37. Harrod distinguished seven different scenarios relating (G, GW, Gn) which give rise to policy conflict or harmony between growth and inflation/deflation and long-term growth objectives (see Table 1 for a summary)38. Scenarios 1 and 3 portray a situation where the warranted rate of growth exceeds and has a steeper slope than the actual rate of growth (GW > G). The tendency towards stagnation or recession could be mitigated by reducing (GW) by an export boom or by a shift to more capital-intensive methods than the average in the production of goods.
Table 1 Taxonomy of different types of the impact of expansionist policies on the relationships between the actual, warranted and natural growth rates (G, GW, Gn) and their implications on inflation, employment and long-run growth
| Cases depicting the relations between the actual, warranted and natural growth rates at a point in time (G, GW, Gn) | Warranted and natural rates of growth | Warranted and actual rates of growth | Natural and actual growth rates | Conflict or harmony | |
|---|---|---|---|---|---|
| First case GW > Gn > G This inequality occurs after a position of full employment |
GW > Gn Savings exceeds that which is required to sustain a growing labor force and technological progress. Dynamised version of “stagnation thesis.” The policy recommendation is to regulate savings by reducing budget surpluses or increasing deficits. |
GW > G The downward runway movement of G from GW means that unemployment will develop unless expansionary policies are undertaken. Expansionary monetary policy would check the downward runa way movement, would also reduce profits and hence savings (given a higher propensity to save out of profits than wages). |
Gn > G No inflationary pressures |
Expansionary policies need not cause a conflict. Positive effects on employment and growth. | |
| Second case G > GW > Gn This inequality occurs before a position of full employment |
GW > Gn Savings exceeds that which is required to sustain a growing labor force and techno logical progress. Dynamised version of “stagnation thesis.” The policy recommendation is to regulate savings by reducing budget surpluses or increasing deficits. |
G > GW The upward runway movement of G from GW means that unemployment will decline but that inflation would result if contractionary policies were not undertaken. |
G > Gn Inflationary pressures |
Expansionary policies increase employment but also as full employment is approached will create inflationary pressures. Positive effect on longterm growth. |
|
| Third case GW > G > Gn This inequality occurs before a position of full employment |
GW > Gn Savings exceeds that which is required to sustain a growing labor force and technological progress. Dynamised version of “stagnation thesis.” The policy recommendation is to regulate savings by reducing budget surpluses or increasing deficits. |
GW > G The downward runway movement of G from GW means that unemployment will develop unless expansionary policies are undertaken. |
G > Gn Inflationary pressures. However, these will be checked by the downward runaway movement of G from GW |
Expansionary policies need not cause a conflict. Positive effects on employment and growth. | |
| Fourth case Gn > G > GW This inequality occurs after a position of full employment |
Gn > GW Savings falls short of that which is required to sustain a growing labor force and technological progress. |
G > GW The upward runway movement of G from GW means that unemployment will decline but that inflation would result if contractionary policies were not undertaken. |
Gn > G No inflationary pressures |
Expansionary policies increase employment but also cause inflation. Negative effects on growth. | |
| Fifth case Gn > GW > G This inequality occurs at a position of full employment |
Gn > GW Savings falls short of that which is required to sustain a growing labor force and technological progress. |
GW > G The downward runway movement of G from GW means that unemployment will develop unless expansionary policies are undertaken. |
Gn > G No inflationary pressures |
Expansionary policies increase employment and need not increase inflation. | |
| Sixth case G > Gn > GW This inequality occurs before a position of full employment |
Gn > GW Savings falls short of that which is required to sustain a growing labor force and technological progress. |
G > GW The upward runway movement of G from GW means that unemployment will decline but that inflation would result if contractionary policies were not undertaken. |
G > Gn Inflationary pressures |
Expansionary policies will have positive effects on employment but create inflationary pressures. Negative effects on growth. | |
| Seventh case Gn > GW > G This inequality occurs before a position of full employment |
Gn > GW Savings falls short of that which is required to sustain a growing labor force and technological progress. |
GW > G The downward runway movement of G from GW means that unemployment will develop unless expansionary policies are undertaken. |
Gn > G No inflationary pressures |
Expansionary policies will have positive effects on employment without inflationary pressures. Negative effects on growth. |
Source: On the basis of Pérez Caldentey (2019) and Harrod (1973, Chapters 7 and 9).
Scenario 2 describes a situation where the natural rate exceeds the natural rate which in turn is above the warranted rate of growth (Gn > G > GW). However, the slope of the natural rate of growth is shallower than that of the actual and warranted rate of growth. The position of the different rates sets the stage for a recession. The actual rate will diverge away from the warranted rate and hit the natural growth rate ceiling. For its part the warranted rate of growth may continue to increase above the natural rate leading to a decline in the actual rate of growth. The recession cannot be avoided but could be mitigated if the natural rate of growth is increased and the warranted rate of growth reduced via a rise in exports in those sectors in which technological progress was more rapid than on average in the economy and with capital intensity greater than the average in the economy39.
In scenarios 4 to 7, a rise in exports and the technical conditions for increasing the natural rate of growth while decreasing the warranted rate of growth increases the difficulty of achieving full employment and utilization of resources and exacerbates the conflicts. In scenarios 4 to 7, the slope of the natural rate of growth is steeper than that of the warranted rate of growth.
According to Thirlwall, in an open economy the ultimate constraint to growth is the balance-of-payments equilibrium growth rate and not the Harrodian natural rate of growth (Thirlwall, 2001, p. 87). It is also the main determinant of the actual rate of growth. As a result, an appropriate analysis of growth based on Harrod’s approach to dynamics must combine the natural, warranted and balance-of-payments constrained rate of growth. Using a similar approach to that of Harrod (1973, Chapter 7), Thirlwall identifies six scenarios of the three growth rates. These are summarized along with the policy implications in Table 2. Scenarios three, four and six are defined by a natural rate of growth above the warranted rate of growth (GW < Gn) since it characterizes the predicament of less developed countries (the coexistence of unemployment and underemployment and inflation) which is the theme of the next section of this paper. Thirlwall addresses this issue in more detail in three articles on Keynes and economic development40.
Table 2 Constellations of the warranted rate of growth, the natural rate and the balance-of-payments equilibrium growth rates (GW, Gn, Gbp)
| Cases depicting the relations between the balance-of-payments equilibrium growth rate, warranted and natural growth rates at a point in time (Gbp, GW, Gn) | Natural rate of growth and balance-of-payments equilibrium growth rate | Warranted rate of growth and natural rate of growth | |
|---|---|---|---|
| First case GW > Gn > Gbp Combination of excess ex-ante savings with a balance of pay- ments deficits. |
Gn > Gbp Depicts the case of a demand constrained econ- omy. The balance-of-payments equilibrium growth rate is below the natural growth rate. As a result, capacity is not being fully utilized and there is employment. |
GW > Gn Further departure of the warranted rate of growth from the natural rate of growth. Since Gn > Gbp the economy has a tendency to a balance of payments >deficits financed in the short-run with financial inflows. This increases the warranted rate. |
|
| Second case GW > Gn < Gbp Fifth case GW > Gbp > Gn |
Gn < Gbp The economy accumulates balance-of-payment surpluses and exports capital. |
GW > Gn Reduction in the warranted rate of growth. |
|
| Third case GW < Gn > Gbp Sixth case GW < Gbp < Gn |
Gn > Gbp This gives rise to conflicting effects. The bal- ance-of-payments equilibrium is below the war- ranted rate of growth implying an inflationary situation unless the natural rate of growth be- comes endogenous to the balance-of-payments equilibrium growth rate. The balance-of-pay- ments deficits must be offset with capital flows which have a positive effect on the warranted rate of growth. This is characteristic of (non-oil producing) developing countries. |
GW < Gn Planned investment exceeds planned savings. This will result in inflationary pressures. Since at the same time the growth of the labor force exceeds the rate of capital accumulation, inflation will coexist with unemployment. This characterizes the development process of developing economies. This is developed more fully in the next section. |
|
| Fourth case G W < Gn < Gbp |
Gn < Gbp There are balance-of-payments surpluses. These reduce the warranted rate of growth aggravating the disparity between the warranted and natural rates of growth. This situation is characteristic of oil-producing developing countries. |
GW < Gn Planned investment exceeds planned savings. This will result in inflationary pressures. Since at the same time the growth of the labor force exceeds the rate of capital accumulation, inflation will coexist with unemployment. This characterizes the development process of developing economies. This is developed more fully in the next section. |
Source: On the basis of Thirlwall (2001, pp. 85-86).
7. HARROD’S GROWTH THEORY AND ECONOMIC DEVELOPMENT
Both Harrod and Thirlwall’s writings attest to their interest and concern in the social and economic problems of developing countries. An important part of Thirlwall’s research on this topic has consisted in the successful application of approaches designed originally to analyze and/or explain the performance of developed economies. This includes the balance-of-payments constraint approach to growth, Kaldor’s growth laws and, also, Harrod’s fundamental equations.
According to Thirlwall the process of economic development is a generalized process of capital accumulation and developing countries face a scarcity of capital. Thirlwall refers to the “strong association between the level of capital deepening and income per head and between the ratio of investment to GDP [Gross Domestic Product] and the rate of growth of output.”41
At the same time less developed countries and poorer countries are characterized by a surplus of labor. Surplus labor is explained on the one hand by disguised unemployment (underemployment in the land) due to the imbalance between the demand and supply of land-based products. This is explained in turn by an income elasticity of demand for agricultural products that is less than unitary (Engel’s law). As a result, the excess labor either depresses the average product and the agricultural wage or migrates to urban areas which leads to an increase in unproductive activities and/or a rising informal sector thus also putting downward pressure on the average product and the corresponding wages42.
According to Thirlwall the imbalance between capital accumulation and the growth of the labor force lends itself to be analyzed through the lens of the relationship between Harrod’s natural rate of growth and the warranted rate of growth43. This framework also provides an avenue to identify different policy options to redress this imbalance.
In less developed economies and poorer economies the natural rate of growth exceeds the warranted rate of growth (Gn > GW).44 Abstracting from the actual rate of growth, in Harrod’s taxonomy (Harrod, 1973, Chapter 7) this situation corresponds to cases 4-7 which are characterized by under savings. In the case of less developing and poorer countries a natural rate of growth which exceeds the warranted rate of growth leads to the coexistence of unemployment (since labor supply exceeds labor demand) and inflation (since ex ante savings are below ex ante investment).
Thirlwall identifies four policy options to redress the imbalance between Gn and GW. This is explained with the pedagogical device of figures 1 and 2. In figure 1 the warranted rate of growth is determined by the intersection of planned savings and investment (S/Y = I/Y ⇔ S = I)45. When the natural rate of growth exceeds the warranted rate of growth (Gn = n + l > GW = so/c) where l, τ are the rate of the labor force and the rate of growth of labor productivity) planned investment exceeds savings (S/Y < I/Y ⇔ S < I). This is the case characterizing developing economies. The opposite case occurs with developed economies.

Source: Thirlwall (2015, p. 141 and 181).
Figure 1 The relation between the natural and warranted rates of growth for developing and developed economies
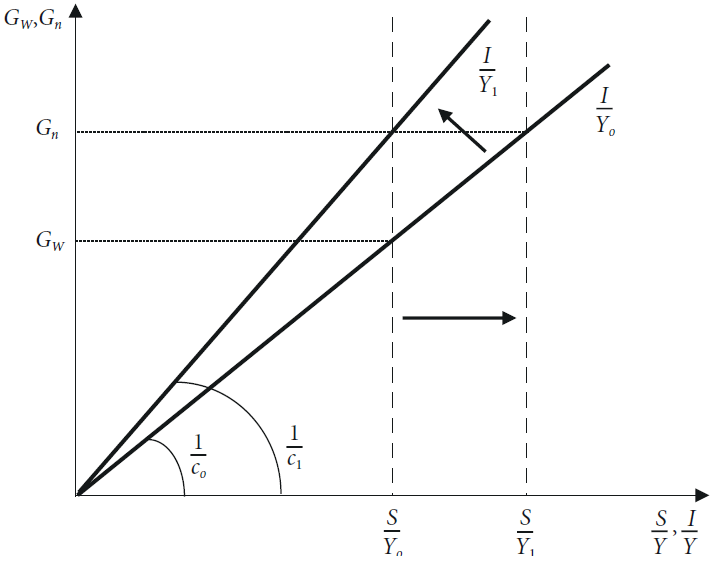
Source: Thirlwall (2015, p. 181).
Figure 2 Policy options to increase the warranted rate of growth (GW)
In the case of developing economies redressing the imbalance between Gn and GW, and thus between S and I requires reducing Gn and/or increasing GW. The policy options for reducing Gn include reducing the rate of growth of the labor force (l) through population control measures and/or reducing the rate of labor productivity growth (τ). The former policy option could not be implemented in the short-run and the latter would have detrimental effects on the economic and social conditions of workers.
An alternative is to increase the warranted rate of growth by raising ‘the amount of saving that people currently want to make’ (Δsd) and/or by reducing ‘the amount of capital goods on hand, fixed and circulating, that corresponds exactly to that which people find convenient.’ (c). An increased desire to save necessarily reduces the value of the multiplier and thus aggregate demand with detrimental effect on working conditions and living standards unless it is accompanied by an increase in autonomous demand that more than offsets the impact on output of an increased propensity of save. Thirlwall considers that the use of more labor-intensive production techniques is a feasible to reduce the capital-output coefficient to move the warranted rate of growth (ΔGW) towards the natural rate of growth (Gn) without reducing output and the level of savings. The use of more labor-intensive techniques increases employment and consumption and income. The consequent reduction in unemployment (and probably in informality) can reduce the level of social precariousness and improve the human capital base of an economy.
8. CONCLUSION
Thirlwall’s contributions to economics complement and extend in an essential and necessary way the work and insights of Harrod on the open economy and growth theory. They also address its limitations and contradictions.
Harrod was aware that his foreign trade multiplier belonged to the realm of static analysis. However, he never got around to developing a dynamic theory for the open economy. In the fourth edition of International Economics (Harrod, 1957 [1933]), Harrod introduced a section on growth and the balance of payments. He focusses on the potential imbalances caused by an increase in exports working through the accelerator and on the remedies to correct for these imbalances. But as Harrod explains the analysis is limited: “A complete theory of dynamics… is unhappily still in a rudimentary condition.”46
Replacing the static version of the foreign trade multiplier by its dynamic analogue has profound analytical and policy implications for growth theory. Internally driven demand expansions are not sustainable unless structural change-oriented policies are implemented to change the ratio of income elasticities. This applies to both the national and regional context [see also Thirlwall, 1985). Even, within the case contemplated by Harrod (1957 [1933]) the impact of an export led boom may be short-lived depending on the strength of the accelerator mechanism. The dynamic foreign trade multiplier also provides a more convincing explanation of growth within a commercial and financially integrated world than the traditional approaches especially for those economies that do not issue an internationally accepted reserve currency.
The introduction of the balance-of-payments equilibrium growth rate changes Harrod’s dynamic framework. The actual rate of growth is replaced by the balance-of-payments constrained growth rate and the natural rate becomes endogenous to this under full employment equilibrium growth rate (León-Ledesma and Thirlwall, 2000, 2002; Perrotini and Tlatelpa, 2003; Perrotini-Hernández and Vázquez-Muñoz, 2019). The relevant comparisons are between the balance-of-payments equilibrium, natural and warranted rates of growth. The consequent scenarios provide useful guidelines for the understanding of the available policy alternatives for sustained growth over time. One of the scenarios most fully developed by Thirlwall relate to the natural rate of growth being above the warranted rate of growth implying the coexistence of inflation and unemployment which is characteristic of the less developed economies.
Thirlwall’s efforts to complement Harrod’s views on the open economy, growth, and dynamics, to identify and remedy their shortcomings set a precedent to re-examine other parts of Harrod’s thinking that have not captured the attention they deserve. This includes, among others, his writings on methodology and, most importantly, on the reform of the international financial architecture which is such a currently pressing issue.











 nueva página del texto (beta)
nueva página del texto (beta)


