1. Introduction^{1 3 4 5 6 7} Fig. 1 ^{8 13 14 18 19 20 21 22 23 24}

FIGURE 1 Orthorhombic perovskite structure of ReTmO₃. 

2. Experiment

where (Re = La, Ce, Nd, Gd, Dy, Y) and (Tm = Fe, Cr). Nucleation is responsible for the formation of grains. In our case transition metal ions act as seed crystal and responsible of nucleation during the sol-gel process. The stoichiometric ratio of rare earth and transition metal ions is equal, so more nucleation was observed in synthesis process that is responsible to nanocrystals formation. The phase identification was carried out using Bruker D8 advance X-ray diffractometer equipped with Cu-K α source of X-rays of wavelength 1.54056 Å. Average grain size was estimated by Scherrer’s formula considering the position and broadening of the most intense diffraction peak in XRD spectra and by Williamson Hall analysis. Morphology and elemental composition of the samples were observed using FEI NOVA 450 scanning electron microscope (SEM) equipped with Oxford energy dispersive X-rays spectroscopy (EDS) detector. The UV-visible measurements were taken by using Perkin Elmer Lambda 950 UV/VIS/NIR spectrophotometer.

3. Results and discussion

(5)3.1. Structural and morphological Properties

Figure 2 shows the X-ray diffraction pattern of a representative sample LaFeO₃ among the prepared samples analyzed by using Rietveld refinement technique considering orthorhombic structure with Pbnm space group. The pseudo-Voigt function was used to perform fitting of diffraction peaks by using the JANA2006 software. A good fitting has been clearly seen between observed and refined XRD data, as all the peaks are well overlapped with fitted data, which confirm single phase has been successfully formed and no impurity peak has been observed. All samples of ReTmO₃ (Re = La, Nd, Gd, Dy, Y and Tm = Fe, Cr) were fitted by JANA2006 (not shown here). Figure 3(a) shows the XRD spectra of polycrystalline ReTmO₃. The diffraction peaks are narrow and sharp, which reflects the high crystalline nature of the prepared samples. The diffraction pattern of ReTmO₃ (Re = La, Nd, Gd, Dy, Y and Tm = Fe, Cr), suggest orthorhombic perovskite structure with space group pbnm (No. 62). The XRD pattern of polycrystalline Ce(Fe,Cr)O₃, where a couple of secondary phases are present as shown in Fig. 3(b). Thus single phase Ce(Fe,Cr)O₃ could not be obtained by sol-gel combustion method. The calculated lattice parameters (a, b, c), unit cell volume (V), and data collected about miller indices (hkl) of lattice planes of as prepared ReTmO₃ (Re = La, Nd, Gd, Dy, Y and Tm = Fe, Cr), are tabulated in Table I. It is clearly shown that, in all cases, c/2 lies between a and b(b>c/2>a) (see Table I), this is the characteristic of the O-type orthorhombicaly distorted perovskite oxides²⁵, where the distortion occurs due to the steric effect and Jahn Teller effect⁹.

FIGURE 2 (color online) Rietveld refinement of the XRD pattern by using the JANA2006 program of representative sample LaFeO3. Inset shows zoom region of the fit of calculated curve on observed curve. 

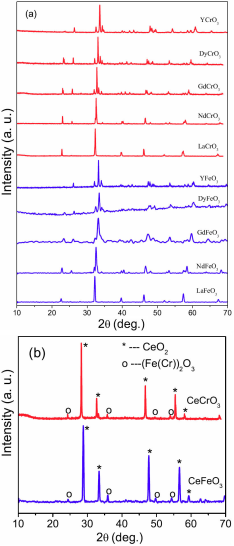
FIGURE 3 XRD pattern of (a) ReTmO₃ (Re = La, Nd, Gd, Dy, Y and Tm = Fe, Cr) and (b) Ce(Fe,Cr)O₃. The asterisks on the peaks show the cerium dioxide (CeO2) phase and circles on the peaks show. Iron(III) oxide or ferric oxide (Fe₂O₃) and Chromium(III) oxide (Cr₂O₃). 

The values of average grain size of ReTmO₃, which was calculated by using the well known Scherrer’s formula [ D=0.89λ/βcosθ, where λ is the wavelength of X-ray radiation (1.54056 Å), θ is the diffraction angle and β is the full width at half maximum (FWHM) of diffracted peaks], are shown in Table II and Table III. It is obvious to identify that the grain size decreases as ionic radius of Re and Tm decreases.

In nanomaterials lattice strain and grain size both have their self contribution to peak broadening of X-ray diffraction and lattice strain is to be contributed in peak broadening due to large volume of grain boundaries^26,27. In order to measure the grain size precisely, the lattice strain calculations are very important²⁸. Hence, the Williamson-Hall (W-H) method was used for estimating the lattice strain and grain size^29,30. In addition, lattice strain and grain size independently contribute to the total peak broadening. The peak broadening induced by strain (βS) is given by the relation βS = 4 εtanθhkl. Assuming that the strain present in the material is uniform, the W-H equation for the total peak broadening is given by³¹,

Rearranging Eq. (3) gives:

where k is the shape factor and D is the grain size. A graph is plotted by taking 4sinθhkl along X-axis and βcosθhkl along Y-axis as shown in Fig. 4. In W-H analysis, the strain present in the material is extracted from the slope and the grain size is estimated from the Y-intercept of the linear fit made to the plot. The estimated values of grain size and lattice strain are (60.52 nm) and (1.32×10-3) for NdFeO₃ and (67.60 nm) and (2.57×10-3) for NdCrO₃ respectively. The small values of lattice strain indicate that the volume of grain boundries should be small for prepared samples. The values of grain size estimated by W-H analysis for ReFeO₃ and ReCrO₃ are shown in Table II and Table III, respectively. The SEM micrographs of all samples of the polycrystalline ReTmO₃ (Re = La, Nd, Gd, Dy, Y and Tm = Fe, Cr) which were taken at accelerating voltage of 10 KV and magnification of 80 K are shown in Fig. 5(a) to (l). Large grains with less grain boundaries can easily be seen from micrographs. However, grains show no perfect alignment, which is a typical characteristic of polycrystalline sample. The individual grains shown in the Fig. 5(a) to (l), are of the round shape with an average grain size 25-75 nm, which is consistent with XRD results. Moreover, from Fig. 5(f) and 5(l), which show the SEM images of CeCrO₃ and CeFeO₃ respectively, it is clear there is no formation of grains were observed as in other SEM images. These results also consistant with XRD analysis, the two phase formation in CeCeO₃ and CeFeO₃. In Fig. 6(a) to (l), EDX spectra show the chemical composition of the synthesized samples of ReTmO₃ (Re = La, Nd, Gd, Dy, Y and Tm = Fe, Cr). It can be seen that there are clear peaks of rare earth elements (Re = La, Nd, Gd, Dy, Y), Iron (Fe), Chromium (Cr), and oxygen (O) elements present with the molar ratio of about 1:1:3 (Re:Fe(Cr):O), giving a stoichiometric formula for ReFe(Cr)O₃, confirmed with analysis of atomic % of all elements present in a sample shown in Fig. 6(a) to (l). There is no peak of any impurity elements which confirms the single phase formation of the samples. However, single peak of carbon appeared which is due to the carbon tape on which samples were mounted with holder. The value of average grain size calculated from XRD pattern is consistent with the one obtained from SEM analysis.

FIGURE 4 Williamson Hall analysis of (a) NdFeO₃ and (b) NdCrO₃. 

FIGURE 5 SEM images of perovskite (a) LaCrO₃ (b) NdCrO₃ (c) GdCrO₃ (d) DyCrO₃ (e) YCrO₃ (f) CeCrO₃ (g) LaFeO₃ (h) NdFeO₃ (i) GdFeO₃ (j) DyFeO₃ (k) YFeO₃ and (l) CeFeO₃. 

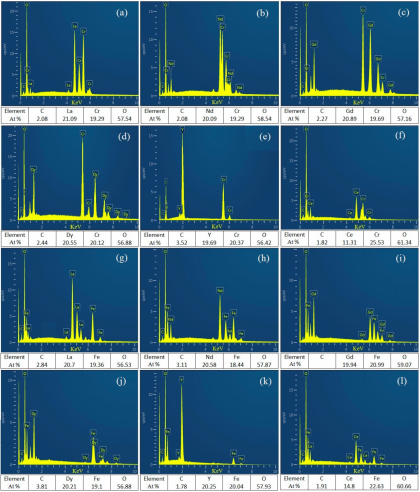
FIGURE 6 EDX images of perovskite (a) LaCrO₃ (b) NdCrO₃ (c) GdCrO₃ (d) DyCrO₃ (e) YCrO₃ (f) CeCrO₃ (g) LaFeO₃ (h) NdFeO₃ (i) GdFeO₃ (j) DyFeO₃ (k) YFeO₃ and (l) CeFeO₃. 

The variation in lattice parameters (a,b,c/2) and cell volume (V) with ionic radii of rare earth cations on Re site in the prepared ReFeO₃ (Re = La, Nd, Gd, Dy, Y) is shown in Fig. 7(a). It is obvious from the graph that a, c/2 and V increases with the increase in the ionic radius of Re site ion i.e. [from Y³⁺ (1.04 Å) to La³⁺ (1.172 Å)], but lattice parameter b remains almost unaltered. The similar variations were observed in lattice parameters and cell volume for ReCrO₃ (Re = La, Nd, Gd, Dy, Y), not shown in the graph, but values for this system are given in Table I.

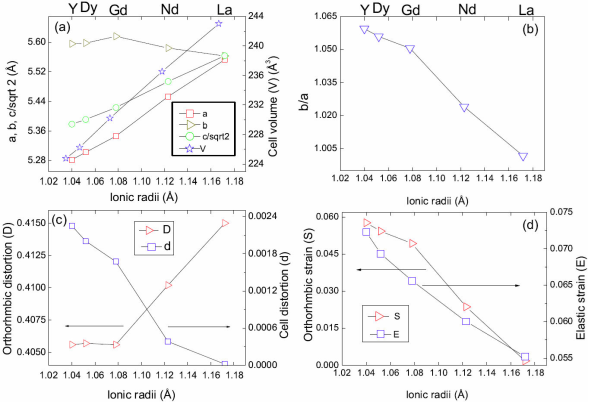
FIGURE 7 Variation of (a) lattice parameters (a; b; c= √2) and cell volume V. (b) Orthorhombicity factor (b/a). (c) Orthorhombic distortion (D) and cell distortion (d). (d) Orthorhombic strain (S) and Elastic strain (E) with ionic radius of Re-site. 

The orthorhombic factor (b/a) sharply increases, with decreasing ionic radius of Re site in ReFeO₃, as shown in the Fig. 7(b). Similar trend is also obtained for ReCrO₃, not shown here. To study the structural distortion, cell distortion (d) [32] was calculated as,

where ap=(a/2+b/2+c/2)/3. The value of cell distortion increases for both ReFe(Cr)O₃ by the replacement of large ionic radius La³⁺ to small ionic radius Y³⁺ are given in Table II and Table III. It is worth mentioning that the decrease in cell distortion has been observed when we replace bigger Re3+ cation in ReFeO₃ as indicated in Fig.7(c), similar results were obtained for ReCrO₃. According to the analysis mentioned above, the variance of lattice parameters with coupled substitution on Re site and on Tm site is the result of distortion in TmO₆ octahedra for matching Re sizes. That is why distortion at octahedral site increases with replacement of the small ionic radius on Re site, because the cations rearrange themselves in such a way that they fit in the unit cell and results in decreasing the unit cell volume.

Orthorhombic distortion (D), which is defined as the ratio of standard deviation to average of the lattice parameters is calculated as³³,

where ai = a, b and c/2 and a¯ is the average of ai. The orthorhombic distortion remains almost constant in region of ionic radii of Re3+ ≤ 1.078 Å, in this region lattice parameter b increases slightly. After further increase in ionic radio of Re3+ a linear increase has been observed in orthorhombic distortion, in this region lattice parameter b slightly decreases. The variation in orthorhombic distortion with Re3+ ionic radii is shown in Fig. 7(c). The elastic strain of the prepared compounds can be calculated by using the formula E = β/2cotθ. The value of elastic strain increases with the decrease in the grain size both for ReFeO₃ and ReCrO₃ as obvious from Table II and Table III. The observed variation in the calculated elastic strain in ReFe(Cr)O₃ elaborate the broadening of the XRD pattern. The elastic strain decreases with the increase in Re site ionic radii for ReFeO₃ as shown in Fig. 7(d). The spontaneous orthorhombic strain, defined as S = 2(a - b)/(a + b), is tabulated in Table II. The value of S decreases from 0.0577 to 0.0118 for YFO to LFO. The variation in orthorhombic strain with ionic radius of rare earth cations is shown in Fig. 7(d). The reason for decrease of this parameter may be the substitution on Re site of smaller ionic radius cation Y³⁺ to bigger La³⁺. The similar results also obtained for YCO to LCO i.e. 0.0493 to 0.0062. It is obvious from the results that replacement of small radius transition metal cation Fe³⁺ to big radius Cr³⁺ on Tm-site of ReTmO₃ also gives the similar trend i.e. decrease in the orthorhombic strain. The X-ray density, bulk density and porosity for all samples were determined using the following relations

FIGURE 7 Variation of (a) lattice parameters (a; b; c=√2) and cell volume V. (b) Orthorhombicity factor (b/a). (c) Orthorhombic distortion (D) and cell distortion (d). (d) Orthorhombic strain (S) and Elastic strain (E) with ionic radius of Re-site. 

where M the molar mass, Z is the number of molecules per formula unit (4 for the orthorhombic structure), N _A Avogadro s number (6.02×1023 /mole) and V cell is the unit cell volume. The bulk density was calculated by the following relation,

where 𝑚 is the mass and 𝑉 = πr2h (where r is the radius and h is the height/thickness of pellet) is the volume of the pellet. The porosity (P) of all the samples was calculated using the equation,

where db is the bulk density and dx is the X-ray density. The measured values of dx, db, and P of ReFeO₃ for various Re (Re = La, Nd, Gd, Dy, Y) site replacement are tabulated in Table II. Figure 8 shows that the dx and the db increase with increase in the formula weight of the prepared ReFeO₃ for various Re (Re = La, Nd, Gd, Dy, Y) compounds. The increase in the dx is considered to be due to the fact that the atomic mass of various Re [Re= Y (89 amu), La (139 amu), Nd (144 amu), Gd (157 amu), Dy (162.5 amu)] increases due to which formula weight or mass of the compound increases . While the increase in the db is due to the fact that increase in value of densities of Re [Re = Y (4.472 g/cm³), La (6.162 g/cm³), Nd (7.400 g/cm³), Gd (7.900 g/cm³), Dy (8.536 g/cm³)]. The magnitude of the db is smaller than that of the dx as can be seen from the graph. These results indicate that the experimental db is less than the theoretical dx due to the presence of pores created during preparation or sintering process of the samples . Figure 8 shows the 𝑃 decreases with the increase of formula weight of the sample from YFO to LFO, but remains almost constant for further increase in the formula weight of prepared compounds. The increase in the db confirms that samples become denser with the increase in density of individual Re site element, due to which the porosity of the samples decreases.

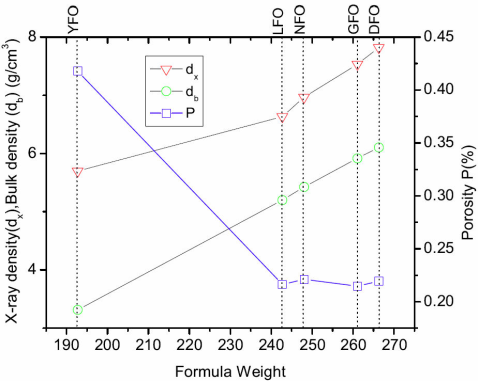
FIGURE 8 Variation in x-ray density (dx), bulk density (db) and porosity (P) with variation of the formula weight of ReFeO₃ (Re = Y, La, Nd, Gd, Dy). 

The Brunauer-Emmett-Teller (BET) specific surface areas of ReTmO₃ (Re = La, Nd, Gd, Dy, Y and Tm = Fe, Cr) nanoparticles are measured by the BET relation of the form,

where D is the orthorhombic distortion and dx-ray is the X-ray density. The values of the specific surface area are given in Table II and III for ReFeO₃ and ReCrO₃, respectively.

3.2. Optical Properties

The obtained UV-visible diffuse reflectance spectra (DRS) of 𝑅𝑒FeO 3 and 𝑅𝑒CrO 3 (Re = La, Ce, Nd, Gd, Dy, Y) were measured by using UV/VIS/NIR spectrophotometer and are shown in Fig. 9(a) and (b), respectively. The absorbance spectrum of ReFeO₃ and ReCrO₃ is obtained from their reflectance spectrum according to the Kubelka-Munk theory³⁸. Moreover, optical energy band gap of ReFeO₃ and ReCrO₃ (Re = La, Ce, Nd, Gd, Dy, Y) is estimated by using the Tauc relation³⁹.

FIGURE 9 UV-visible reflectance spectra of (a) ReFeO₃ and (b) ReCrO₃. 

αhν=A(hν-Eg)n, (11)

where hv is the energy of the incident photon, α is the absorption coefficient, A is a characteristic parameter, Eg is the band gap. Exponent n specify the type of transition and it may be 1/2 or 2 for the allowed direct or allowed indirect transition, respectively. Here, we assume a direct band gap system and from the plot of (αhν)1/2 versus hv by extrapolating the linear portion to the hv (i.e. α = 0) determine theE _g . The obtained value of E _g for ReFeO₃ and ReCrO₃ is found to be in the range 1.77 - 1.87 eV and 2.77 - 3.14 eV, respectively as shown in Fig. 10(a) and (b). It is noted that in ReFeO₃ only single band gap is observed due to the transition from O 2p valence band to Fe 3d conduction band. This constitues a charge transfer energy gap. Interestingly, three edges are observed for ReCrO₃ at 1.58 eV, 2.20 eV and 3.14 eV values for YCrO₃. Khuong P Ong et al. have also observed three types of energy gap in LaCrO₃ theoretically . Interestingly similar trend of energy gaps were also observed experimentally in our prepared compounds of family of orthochromites. The energy gap at 1.58 eV (value for YCrO₃), which is the energy band gap among the occupied t_2g (Cr 𝑑 𝑥 2 − 𝑦 2 , 𝑑 𝑥𝑧 , and 𝑑 𝑦𝑧 ) and unoccupied e_g (Cr dz2 and dxy) states, has been observed. This type of energy gap is Mott-type insulating gap. The second edge at 2.20 eV (value for YCrO₃) reveals the optical transitions between the Cr t_2g and Cr e _g bands which are, of course, partly hybridized with O 𝑝. These transitions are in visible range and indicate the color of various orthocromites ReCrO₃. The theoretical calculation reveals that the green color of these prepared compounds have its origin from the transition between Cr t2g bands centered at (-0.23 eV) and Cr e _g bands at (2.15 eV)⁴⁰. Another important transition at 3.14 ev (value for YCrO₃), shows the transition between the top of O p bands and the bottom of the Cr e _g conduction bands, this constitues a charge transfer gap. T. Arima et al. estimated E _g for LaCrO₃ and YCrO₃ experimentally ^41,42, which is consistent with the observed values of optical band gap of ReCrO₃. So far, the experimental optical gap at higher energy edge for ReCrO₃ was considered as the energy band gap between the transition to the top of the valance band and the bottom of the conduction band by previous theoretical works . The minimum E _g for Fe-based system (i.e. 1.77 eV) than that of Cr-based system (i.e. 2.77 eV) may be attributed to lower energy of 3d orbital of Fe than that of Cr.

FIGURE 10 Tauc plot of (a) ReFeO₃ and (b) ReCrO₃. 

4.Conclusion

The sol-gel auto combustion citrate method is successfully used for the synthesis of polycrystalline ReTmO₃ (Re = La, Nd, Gd, Dy, Y and Tm = Fe, Cr) and single phase character has been confirmed by XRD patterns. A systematic increase in grain size with increasing r Re and r Tm has been observed and found to vary from 25-75 nm for both ReFeO₃ and ReCrO₃, which is consistant with SEM results. The various crystallographic parameters have been calculated and found to be affected by changing Re and Tm sites as described earlier. The allowed direct E _g estimated to be 1.77 - 1.87 eV and 2.77 - 3.14 eV for ReFeO₃ and ReCrO₃, respectively. Three edges are observed for ReCrO₃ at 1.58 eV which is a Mott-type insulating gap, at 2.20 eV are in visible range corresponds to light green color of orthocromates and at 3.14 eV this is due to charge transfer gap (E _g ) between O 2p valance and Cr 3d conduction band. Moreover, the E _g has larger value (∼ 1 eV) in ReCrO₃ as compared to ReFeO₃ which may be attributed to the Jahn Teller distortion.