Introduction
Pyrimidines comprise a relatively large, growing and most interesting group of antibacterial drugs. In the recent years, it has made a major impact on the field of antibacterial chemotherapy. Sulfamethazine (SMZ) and sulfamerazine (SMR) are classified as chemotherapeutic agents. They contain pyrimidine nucleus and have clinical use such as an antibacterial and antibiotics. Antibiotics are neutral compounds which are synthesized by living organisms to inhibit the growth of harmful microorganisms [1]. During recent decades, the use of antibiotics in animal husbandry has increased considerably. Sulfonamides are a group of synthetic antimicrobial agents with a broad spectrum of antibacterial activity. They therapeutically are used to treatment infections and prophylactically to improve feed efficiency, and to promote growth [2]. Sulfadiazine, sulfamerazine and sulfamethazine are three sulfa drugs and are commonly called triple sulfa drugs. They introduced in medical therapy because of their antibacterial activities [3]. Sulfamethazine (SMT) is widely used as an antimicrobial agent in the feed of meat-producing animals to treat infections [4]. Pyrimidines and their derivatives have a wide spectrum of biological activities such as antimicrobial, anti-inflammatory, analgesic, antiviral and anticancer activities [5]. Sulfamerazine (Fig. 1) is used as effective antimicrobial agents for the prevention and cure of several types of bacterial infections in human and veterinary therapies. Although SMR and SMT have been widely used in therapeutics, the solubility data of these drugs in co-solvent mixtures is still scarce in the literatures [6]. SMR, the mono-metyl derivative of sulfadiazine, is in fairly wide clinical use at the present time and certain advantages have been claimed for it over the other commonly employed sulfonamide drugs, particularly sulfadiazine [7].
Various experimental procedures are frequently used for the determination of acidity constants. However, there is an increasing interest for theoretical prediction of pKa values, employing various quantum theoretical techniques during the last two decades.
A compound is defined as Bronsted acid, in aqueous solution, if it dissociates as follows:
pKa equals to ∆G/2.303RT, where ∆G is a free energy change of the dissociation reaction either in a gas or solution. As it can be seen, the acidity of a compound can be determined by ∆G value as the follow [8]:
On the basis of solvation free energies, the pKa values were obtained for the compounds by using thermodynamic equations, involving the combined experimental and calculated data [9].
The pKa is an important property of drug molecules. It is the key parameter for drug development because it governs solubility, absorption, distribution, metabolism and elimination. Moreover, the transport of drugs into cells and across other membranes is a function of this physicochemical property.
On the other hand, most drugs are either weak acids or weak bases, therefore, pKa can be an important parameter in drug absorption.
A sulfonamide contains one amine group (-NH2) and one amide group. Amine group is able to take a proton, while the amide group is able to release proton under specific pH conditions (Fig. 1) [10].
The drug-protein complex can serve therefore as a reservoir in the vascular space for sustained drug release to extra vascular tissues, but only for drugs that exhibit a high degree of binding. Thus, the protein-binding characteristics of a drug can play a significant role in its therapeutic effect regardless of the dosage form [11].
In addition, precise measurements of the acid-ionization constants (pKa) of sulfamethazine and sulfamerazine can be hampered by their low solubility in water. In this case, a theoretical investigation would be an alternative way to determine the pKa values [12]. Quantum mechanical calculations in both ab initio and DFT levels of theory have been extensively employed to the study of acidities in the solution phase and the results have been compared with the experimental values. It is well known that the calculated gas-phase acidities using a high level theory such as GAUSSIAN-2 (G2) are reliable. Some recent models of solvation such as polarizable continuum model (PCM) ignore some part of solute- solvent interactions [13]. Quantum chemical calculation has been widely used in the determination of the pKa values in water or organic and inorganic solvents [14]. We used Tomasiʼs method [15] of the B3LYP/6-31+G(d) level of theory for the anion, cation, and neutral species at 298.15 K and compared them to experimental pKa values.
Computational Methods
Two compounds were studied in this research work. These components are sulfamerazine and sulfamethazine as shown in Fig.1. The solvation was included in the calculations using the polarized continuum model (PCM) [16]. The optimization process in the solution phase was performed at the B3LYP/6-31+G(d) level on the structures obtained from solution phase [14].
To analyze the solvent effects on all species continuum model (PCM) of Tomasi et al, was used. Furthermore, to shed light on the experimental pKa values of sulfamerazine and sulfamethazine in water, several conformers were tested by program and some of them were not further considered because the estimated error in its acidic dissociation constants was unacceptable [9]. In the other word, if the conformers aren't energetically stable, they don´t contribute to the study of sulfamerazine and sulfamethazine.
The model calculates short-range interaction energies between solvent and solute using a modified solvent accessible surface area which incorporates parameters for atomic and molecular surface tensions and hydrogen-bound acidity and basicity. It has been proven to be an effective tool to investigate on a variety of solution phase physicochemical properties. Solvation of selected species was finally considered in terms of the intermolecular hydrogen bonds (IHBS) (see Table 1 and Fig. 2) [17].
Table 1 Values of pKa for protonation of sulfamerazine and sulfamethazine that were obtained using the Tomasi method at the B3LYP/6-31+G(d) level of theory, at 298.15 K.

Results and Discussion
The molecular structures of sulfamerazine and sulfamethazine are shown in Fig. 1. As it can be seen in this figure, there are two sites for protonation. First site, -NH2, which is attached to benzene ring, and second site, -NH, which is attached to -SO2 and N2C4H3 ring. It means that the protonated sulfamerazine and sulfamethazine can lose two acidic hydrogens. This first proton can be lost from NH3 + group and the second one from NH group (Fig. 1) [17].
The total free energies (Hartree and kJ.mol-1) of the neutral, cation and anion species of sulfamerazine and sulfamethazine are listed in Tables 2 and 3, respectively. These values were calculated for single and solvated, in water, species at the B3LYP/6-31+G(d) level of the theory using Tomasi’s method. Tables 2 and 3 show that the total free energies (Hartree) for different species of sulfamerazine and sulfamethazine increase when the number of water molecules increases in the solvation. Also, these tables (2 and 3) and Fig. 3 show that the total free energies per water molecule (kJ.mol-1) decrease by increasing of the number of water molecules. All of these changes can be evidence on the endothermic nature of the reaction.
Table 2 Calculated total energies using the Tomasi method at the B3LYP/6-31+G(d) level of theory for cationic, neutral, and anionic species of sulfamerazine, at 298.15 K.
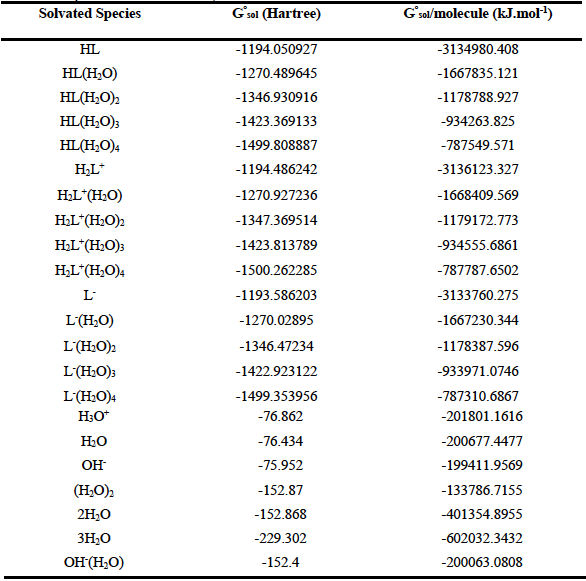
Table 3 Calculated total energies using the Tomasi method at the B3LYP/6-31+G(d) level of theory for cationic, neutral, and anionic species of sulfamethazine, at 298.15 K.
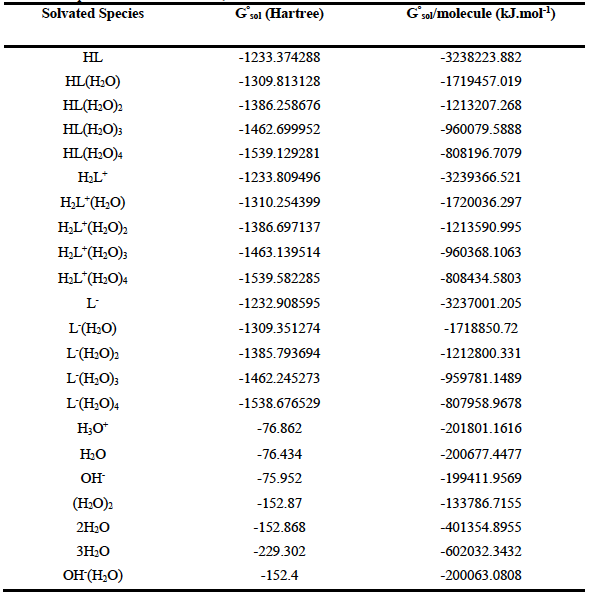
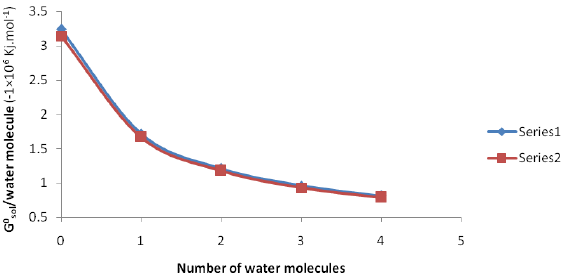
Fig. 3 The total energy (kJ · mol-1) of solvated sulfamethazine (series 1) and sulfamerazine (series 2) cations per water molecule against the number of solvation water molecules.
The structural properties of solvated species of sulfamerazine and sulfamethazine were shown in Tables 4 and 5, respectively. These properties are including of the distance between the indicated atoms (A°), D: dihedral angle between the indicated atoms (°), a◦: bohr radius (A°), q: total atomic charges (Mulliken) (au), d: distance of the IHB between the indicated atoms (A°), and A: H-bond angle (°).
Table 4 Calculated structural magnitudes using Tomasi’s method at the B3LYP/6-31+G(d) level of theory for the cations, anion, neutral molecule of sulfamerazine at 298.15 K.

Table 5 Calculated structural magnitudes using Tomasi’s method at the B3LYP/6-31+G(d) level of theory for the cations, anion, neutral molecule of sulfamethazine at 298.15 K.

Several factors are effective in structural properties of single and solvated species. These factors can be number and size of atoms, nature of agents (electron donor or acceptor effect), and the number of solvent molecules.
First ionization constant of sulfamerazine and sulfamethazine
In alkaline solution, the sulfamerazine and sulfamethazine undergo a reaction of partial neutralization as the below:
In the above reaction, H2L+(H2O)4 is the sulfamerazine and sulfamethazine cations solvated with four water molecules and HL(H2O)4 represents neutral sulfamerazine and sulfamethazine solvated with four water molecules. The described reaction in Eq. 3 is characterized by another equilibrium constant, KC1, which was also theoretically determined.
All aqueous solutions contain hydrogen (H+) and hydroxyl (OH-) ions. In pure water, these ions are entirely derived from the ionization of the water molecules. The autoprotolysis reaction of two molecules water is better represented by the following reaction:
At T = 298.15K, Kw =1.008×10-14 and it shows that only a few of the water molecules are ionized [18].
The reaction 5 is characterized by equilibrium constant, Kal. This reaction was theoretically obtained by incorporating of Eqs.3 and 4. The equation 5 defines the first ionization constant of sulfamerazine and sulfamethazine:
It is obvious that:
The equation 6 was used to theoretically determine the value of the first ionization constant of sulfamerazine and sulfamethazine in water. The values of structural properties for different species of sulfamerazine including, cationH2L+(H2O)4 (Fig. 4A), anion L-(H2O)3 (Fig. 4B), and neutral HL(H2O)4 (Fig. 5A) have been summarized in Table 4. The formation of neutral sulfamerazine and sulfamethazine implies that the electronic density of the N1 atom, in absolute value, significantly decreases with respect to the N1 atom of the sulfamerazine and sulfamethazine cations. It can show the first deprotonation of sulfamerazine and sulfamethazine.
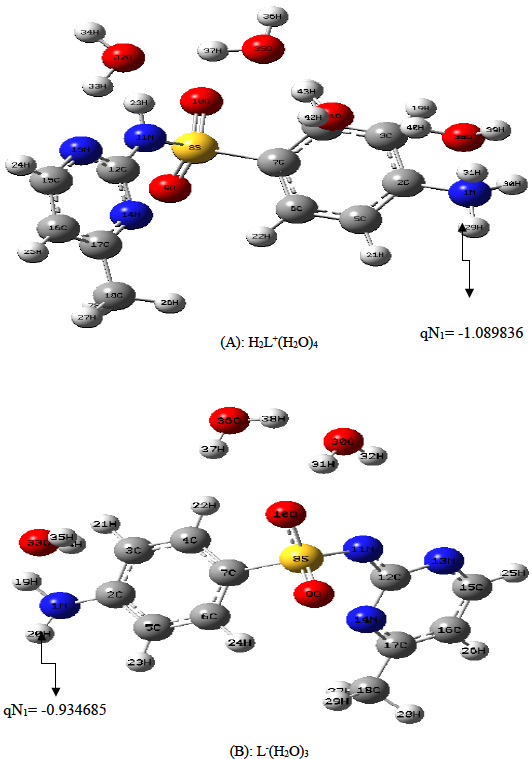
Fig. 4 Calculated structure for the sulfamerazine cation (A) and anion (B) at the B3LYP/6-31+G(d) level of theory and using Tomasi’s method in water at 298.15 K.
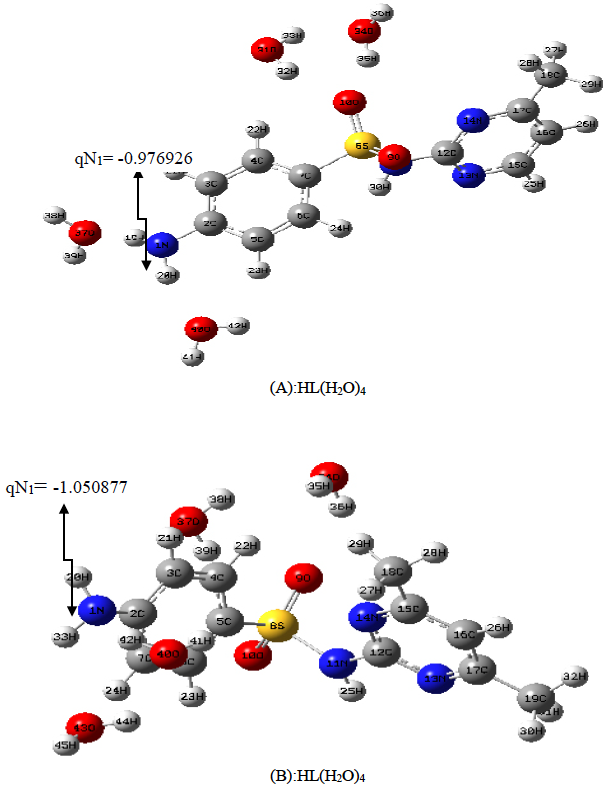
Fig. 5 Calculated structure for the neutral sulfamerazine(A) and sulfamethazine (B) at the B3LYP/6-31+G(d) level of theory and using Tomasi’s method in water at 298.15 K.
As it can be observed in Table 1, the theoretically calculated values of pKa1 for sulfamerazine and sulfamethazine (2.13 and 2.31) are relatively comparable with the experimentally ones (2.17 and 2.37), respectively [10].
KC1 and KC2, equilibrium constants of equations; Ka1 and Ka2, first and second acidic dissociation constants of species in water; D, dihedral angle between the indicated atoms (°); a0, bohr radius (Å); q, total atomic charge (Mulliken) (au); d, bond lengths between the indicated atoms; A, angles (°).
Second ionization constants of sulfamerazine and sulfamethazine
It is selected that the sulfamerazine and sulfamethazine suffer a total neutralization process as the below:
In reaction 7, L-(H2O)3 shows the sulfamerazine and sulfamethazine anion solvated with three water molecules and HL(H2O)4 represents the neutral sulfamerazine and sulfamethazine solvated with four water molecules.
The described reaction in Eq. 7 is characterized by another equilibrium constant, KC2, which was also theoretically determined. By combining Eqs.7 and 4, the second ionization reaction of sulfamerazine and sulfamethazine was obtained as the below:
The second ionization constant (Ka2) that characterizes the above reaction is associated with the constants KC2 and Kw:
The above equation was used to theoretically determine the value of the second ionization constants of sulfamerazine and sulfamethazine in water. The values of structural properties for different species of sulfamethazine including, neutral HL(H2O)4 (Fig. 5B), cation H2L+(H2O)4 (Fig. 6A), and anion L-(H2O)3 (Fig. 6B) have been listed in Table 5. It is clear that the formation of the sulfamerazine and sulfamethazine anion implies that the electronic density of the N11 atom increases notably (in absolute value) with respect to the N11 atom of the neutral sulfamerazine and sulfamethazine. It can show the second deprotonation of sulfamerazine and sulfamethazine.
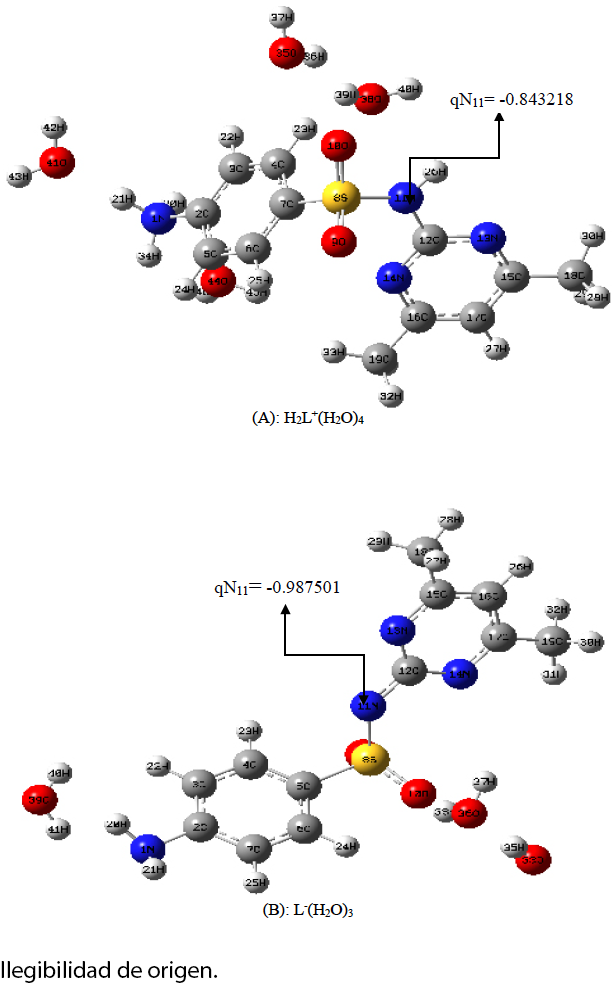
Fig. 6 Calculated structure for the sulfamethazine cation (A) and anion (B) at the B3LYP/6-31+G(d) level of theory and using Tomasi’s method in water at 298.15 K.
Table 1 shows that the theoretically calculated pKa2 values for sulfamerazine and sulfamethazine (6.70 and 7.58) are relatively comparable with the experimentally ones (6.77 and 7.49), respectively [10].
KC1 and KC2, equilibrium constants of equations; Ka1 and Ka2, first and second acidic dissociation constants of species in water; D, dihedral angle between the indicated atoms (°); a0, bohr radius (Å); q, total atomic charge (Mulliken) (au); d, bond lengths between the indicated atoms; A, angles (°).
The molecule of water originated from the acid-base reaction, together with the hydration water molecule of the sulfamerazine and sulfamethazine, and these are the molecules of water that interact with the sulfamerazine and sulfamethazine molecules by means of IHBs. According to Ref 19, the properties of the weak, moderate and strong hydrogen bonds have classified. For species of this study, the distances and angles of intermolecular hydrogen bounds (IHBs) are shown in Tables 4 and 5. These values show that the all species of sulfamerazine and sulfamethazine have moderate IHBs. Data of IHBs is very important because it can be used in the design of benefit and economical nanodrugs that are very useful in the treatment of disease.
Conclusion
In this paper, we theoretically determined the pKa of sulfamerazine and sulfamethazine. We showed the feasibility of a theoretical method that uses to determine the ionization constants of these molecules. The calculations performed at the B3LYP/6-31+G(d) levels of theory using Tomasiʼs method allowed us to prove that cation, anions and neutral molecules from IHBS with sum molecules of water. The theoretical ionization constants show relatively suitable agreement with the acidity constants experimentally determined. This agreement along with the other data (the electronic density, q, structural properties, and IHBs) help us to design nano drug modeling of sulfamerazine and sulfamethazine.











 text new page (beta)
text new page (beta)