Servicios Personalizados
Revista
Articulo
Indicadores
-
 Citado por SciELO
Citado por SciELO -
 Accesos
Accesos
Links relacionados
-
 Similares en
SciELO
Similares en
SciELO
Compartir
Revista mexicana de ciencias forestales
versión impresa ISSN 2007-1132
Rev. mex. de cienc. forestales vol.7 no.37 México sep./oct. 2016
Articles
Rainfall interception in mountain forests in the Texcoco river basin, Mexico
1Campo Experimental Valle de México. INIFAP. CIR-Centro. INIFAP. México. Correo-e: flores.eulogio@inifap.gob.mx
2Sitio Experimental Tlaxcala, CIR-Centro. INIFAP. México
3Académico de la Universidad Autónoma Chapingo. México.
The process of interception of rainfall by tree mass, as a component of the hydrological cycle in forest areas, is determined, among other causes, by the climatic conditions, orographic and by the characteristics of the vegetation. Here are presented the results of a research program that aims to measure the amount of rainfall that three forest communities intercept in the forest -hydrological basin of Texcoco river. Three research modules with an area of 1.0 ha were established in high mountain forests located in an altitudinal range of 2 850 to 3 650 m. In each one, dendrometric measurements were made: normal diameter, total height and clean stem of each tree from 7.5 cm of normal diameter. During the rainy periods of 2009, 2010 and 2011, precipitation measurements were made, and the precipitation amounts and their distribution were determined by rainy season. For the measurements of total precipitation, two rain pluviometers located outside the modules were used. The interception was measured with 75 direct reading pluviometers distributed at random under the canopy. Precipitation-interception were recorded by rainfall event. The amounts intercepted by tree crowns were expressed as percentages for each of the studied groups: fir (26.1 %), oak (21.7 %) and pine (19.2 %). The statistical models of the relationship were generated: total precipitation (mm) - intercepted rain (%), for each group.
Key words: Hydrologic cyicle; forest -hydrological basin; rainfall interception; models; Pinus; Quercus; Abies
El proceso de intercepción de lluvia por la masa arbórea, como componente del ciclo hidrológico en áreas forestales, está determinado, entre otras causas, por las condiciones climáticas, orográficas y por las características de la vegetación. Se presentan resultados de un programa de investigación que tiene como objetivo medir las cantidades de lluvia que interceptan tres comunidades forestales, en la cuenca hidrológico-forestal Río Texcoco. Se establecieron tres módulos de investigación con una superficie de 1.0 ha, en bosques de alta montaña ubicados en un intervalo altitudinal de 2 850 a 3 650 msnm. En cada una, se realizaron mediciones dendrométricas: como diámetro normal, altura total y de fuste limpio, de cada árbol a partir de 7.5 cm de diámetro normal. Durante los periodos de lluvia de los años 2009, 2010 y 201, se realizaron mediciones de precipitación, y se determinaron las cantidades de precipitación y su distribución por periodo de lluvias. Para las mediciones de precipitación total, se emplearon dos pluviómetros ubicados fuera los módulos. La intercepción se midió con 75 pluviómetros de lectura directa distribuidos aleatoriamente bajo el dosel. La precipitación-intercepción se registraron por evento de lluvia. Las cantidades interceptadas por las copas de los árboles se expresaron como porcentajes, para cada uno de los grupos estudiados: oyamel (26.1 %), encino (21.7 %) y pino (19.2 %). Se generaron los modelos estadísticos de la relación: precipitación total (mm) - lluvia interceptada (%), para cada grupo.
Palabras clave: Ciclo hidrológico; cuenca hidrológica-forestal; intercepción de lluvia; modelos; Pinus; Quercus; Abies
Introduction
Hydrology in forest ecosystems is made up of complex processes in which biotic and abiotic factors interact. Although the interception of rainfall by vegetation is not the main factor in hydrological estimates, it does allow identifying that changes in tree cover affect the hydrological balance of a tree area, since it alters the soil moisture content and surface runoff (Sadeghi et al., 2015).
The canopy regulates the flow following precipitation before reaching the forest floor, because it modifies the trajectory of the fall of the drops dividing it into: direct rain, interfoliar rain and rain of fustal runoff. In the interception process, it is recognized that forest vegetation participates in the spatial distribution of rainfall. Includes its different strata, and once it reaches the forest floor has patterns of temporality; in such a way that it constitutes a process of great complexity, by the structural elements of the forest and its interaction with the environment (Kittredge, 1948).
Eventhough forests have no major influence on precipitation, their participation can not be considered insignificant, particularly when accounting for the amount and distribution of rainfall on the forest floor (Kittredge, 1948). This is one of the main reasons why several studies of forest ecology have been aimed at generating relationships between the composition and spatial variability of the forest structure with the interception of rainfall (Flores et al., 2011).
Precipitation in forest ecosystems is divided into direct precipitation (PD), fustal runoff (F) and loss of interception (I), classification that is important in studies of forest hydrology (Marín et al., 2000; Lida et al., 2005).
Net precipitation (PN) is the amount of rain that reaches the forest floor through direct precipitation (PD) and fustal runoff (EF) (Manfroi et al. 2004; Levia and Herwitz 2005; André et al., 2008). PD refers to the rain that reaches the forest floor, passing between treetops or dripping. EF corresponds to the fraction of the rain that reaches the forest floor, sliding through the branches and branches of the trees, is caused by the precipitation intercepted by the canopy components (Staelens et al., 2008). Finally, rain interception (I) is the part of the precipitation retained by the canopy of canopies that does not reach the forest floor by evaporation (Taghi et al., 2013). It is calculated indirectly by the difference of the gross precipitation (PB) measured over the canopy or in a nearby area, and the sum of PD and EF (Aussenac, 1981):
According to Sadeghi et al. (2015), rainfall interception can account for 10-25 % of total rainfall (PB) in deciduous forests, and up to 40 % in perennial forests. Aussenac (1981) records a relation of intercept quantities for different species of Abies, Picea, Pinus, Pseudotsuga, Fagus and Quercus. From the above, it is inferred that the density or levels of forest cover will have an impact on the interception process, as they affect the soil moisture content and surface runoff of the land.
The interception of rainfall and its subsequent evaporation affects the water yield of watersheds, their study is complex and can be very variable. On a large scale, weather factors such as wind speed and exposure, rainfall intensity, and fog incidence determine the loss of interception. At a small scale, an important control of the interception loss is the density and composition of the vegetation that define the capacity of the crown to temporarily store water. Other factors are also involved, such as crown exposure, canopy roughness, and rainwater penetration (Fleischbein, 2005; Siegert, 2014).
The various components of the rainfall interception process have been measured and modeled for many types of vegetation, with particular emphasis on forest stands. However, it is important to recognize the importance of rainfall interception models to predict the effects of changes in plant cover on water resources (Muzylo, 2009), in addition to allow the extrapolation of results of measurements, both in space and time, providing information that refers to the mechanisms involved in the interception process by the tree vegetation and of the attributes that control such processes (David et al., 2005; Návar et al., 2008).
In this context, it is proposed to determine the amount of rainwater retained by the canopy through the rain interception process, and to generate the respective models in three types of high mountain forest in the Texcoco river basin, Mexico.
Materials and Methods
In 2009, a research line was initiated in the Campo Experimental Valle de México de INIFAP (Valle de México Experimental Station from INIFAP) to study the interception of rainwater by arboreal vegetation in the area of forest hydrology in the Texcoco river basin. Coatepec, Santa Mónica, San Bernardino, Chapingo, Coxcacoaco, Xalapango and Papalotla rivers are part of the Texcoco hydrological basin in the State of Mexico (Semarnat, 2015).
Three types of high mountain forest were selected, which were evaluated in three different years: Abies religiosa (Kunth) Schltdl. et Cham. (2009), located at 3 000 masl; Quercus spp. (2010) at 2 900 masl., and Pinus hartwegii Lindl. (2011) at 3 650 masl (Figure 1). The vegetation in each type of forest, arboreal stratum and undergrowth, was identified by botanical collections. The design of the modules consisted of 1.0 ha (100 x 100 m) plots where only dominant tree species were considered. The variables included were: diameter at breast height measured with diametric tape (Ben Meadows), total height and clean stem height measured with Suunto clinometer; location, within each plot, of trees and direct reading pluviometers (Tru-Chek®), using Cartesian coordinates. Additionally, the unevenness of the terrain was measured with a 20 m hose level to generate its physiography.

Figure 1 Location of the research modules within the San Pablo Ixayoc forest ejido, Texcoco municipality, State of Mexico.
The amount of total annual precipitation and its distribution for each forest condition, was determined from measurements of daily precipitation under open conditions. For the measurement of the amount of intercepted rainfall per module in each module, 75 Tru-Check® (direct reading) rain gauges under the canopy, with a precision of 0.1 mm, were placed at random at 0.9 m above the floor level to avoid the splashed effect (Prasad, 2016).
The procedure to express the amount of rainfall intercepted was by the difference between the total precipitation measured under open conditions and the overall average of the water volume of the collectors under the vegetation canopy, for each rainfall event (Xiao et al., 2000 Sadeghi et al., 2015).
The study began in 2009, in the fir plot, continued in 2010, with oak and concluded in 201 with pine. This allowed to generate values of interception by species, precipitation event and per year. The fustal runoff was not quantified.
Based on the dispersion of points, the ratio of total precipitation against the percentage of interception was analyzed. In order to statistically correlate the precipitation and intercept values for each module, logarithmic and exponential non-linear models were fitted by regression analysis (Hosseini et al., 2012; Sadeghi et al., 2015).
The selection criteria of the best adjusted models included the coefficient of determination and significance of the regression parameters. Statistical analysis was performed in the SAS program (SAS, 2007).
Results
Annual Average Precipitation
The general characteristics regarding the location of the three study sites are summarized in Table 1. In terms of altitude, the pine forest is the one located in the highest part of the basin, followed by the fir forest and in the lower part, the oak forest.
Table 2 shows some of the population and dasometric characteristics of the dominant vegetation in each research module.
Table 2 Dominant vegetation of each research module.
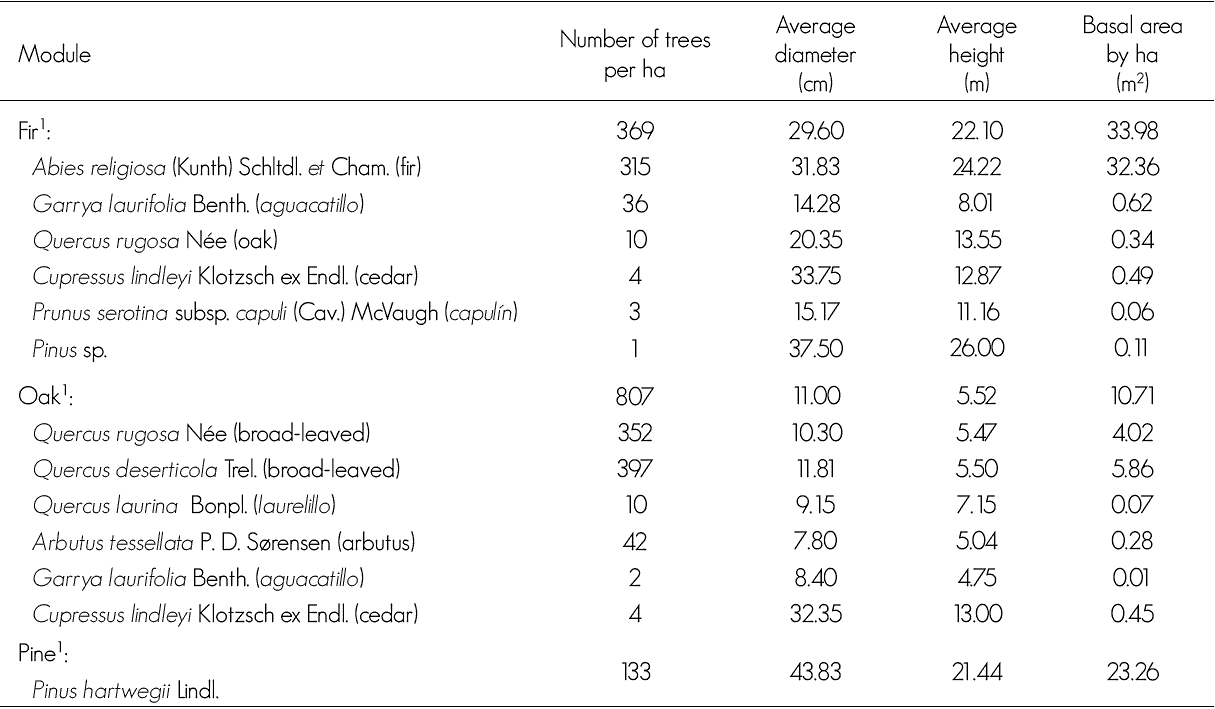
1 = Taxonomic identification performed by Dr. Enrique Guizar Nolazco from the. Herbario of the División de Ciencias Forestales of the Universidad Autónoma Chapingo.
For Pinus, Abies and Quercus, 72, 74 and 63 precipitation events were measured, respectively. Results indicate that for the Pinus hartwegii forest, a cumulative annual rainfall of 680.20 mm, in the Abies religiosa forest, 707.70 mm and for the Quercus spp. Forest, 503.00 mm (Table 3). The intervals of variation per event were 0.2-23.5 mm for Pinus, 0.2-33 mm for Abies and 0.2-38 mm for Quercus. Pinus and Abies recorded atypical data, the first of 82 mm and the second of 62 mm.
Measures of rainfall interception
The measurement of rainfall amounts during the interception process, the number of records of rainfall events varied in quantity, since only the significant ones were included for the evaluation years in the study area.
The calculation procedure consisted in measuring the amount of rainfall intercepted from the difference between the total precipitation measured under open conditions and the general average obtained from the volume of the water collected by the collectors under the canopy for each rain event, expressed as a percentage of total precipitation.
Where:
I = Amount of intercepte rainfall
PT = Amount of total rainfal measured in neighboring areas to vegetation
PBD = Amount of rainfal measured under the canopy
Results are summarized in Table 3
The percentage of intercepted rain refer to the amount of rain that is “lost” from evaporation, since it is captured by the tree cover.
Generation of Interception Models
According to the point dispersal from the relation of total precipitation against interception percentage, and in order to statistically, correlate them, a non-linear regression was made using the PROC GLM of SAS procedure. Exponential and logarithmic models were tested (Scatena, 1990; Hosseini et al., 2012; Sadeghi et al., 2015) (Table 4):
Where:
y = Rain interception under the canopy in percentage
X = Total precipitation (under open sky), measured in mm
Ln = Natural logarithm
a, b = Coefficients of regression
The behavior of the three generated models is graphially shown in Figure 2.
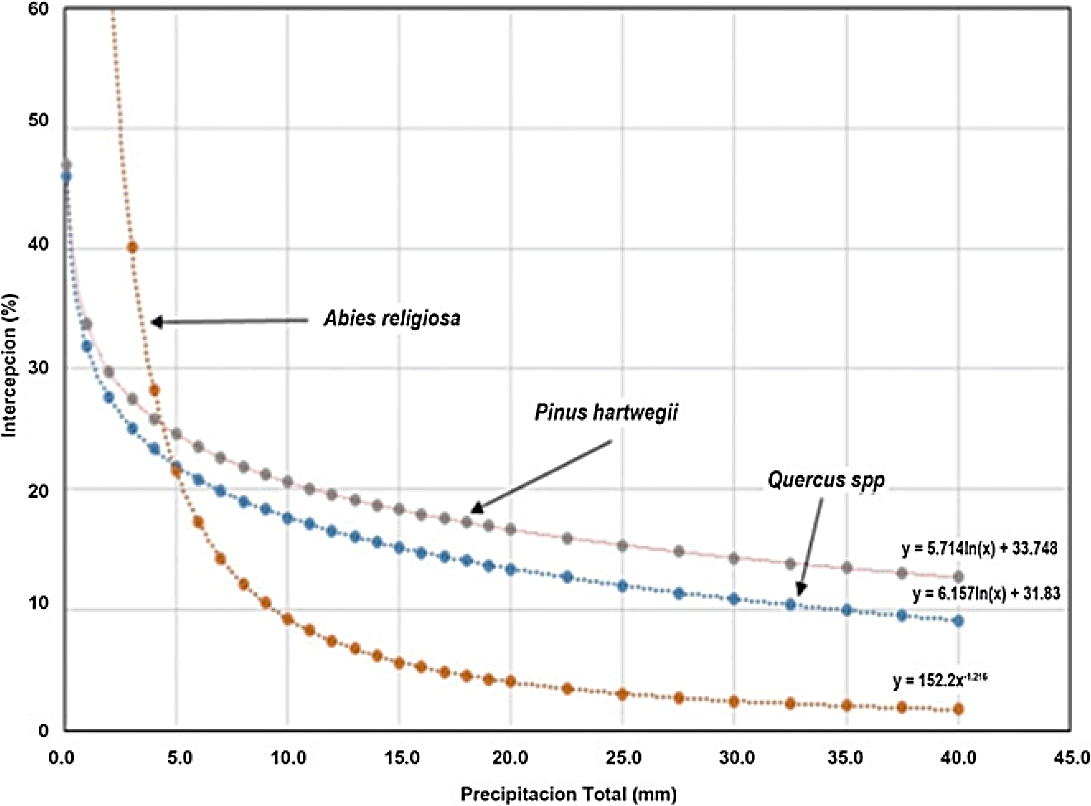
Negative exponential for Abies religiosa (Kunth) Schltdl. et Cham.; negative logarithmic for Pinus hartwegii Lindl. and Quercus spp., from the interception percentage ratio as a function of total precipitation.
Figure 2 Graphic behavior of the regression models from species under study.
The graphic shows the behavior of rainfall interception which reflects a part of the condition of the forest in terms of the mensuration characteristics of the species under study. Abies tends to form more dense masses than Pinus and Quercus and from their habit of keeping a great numberof leaves along the stem, a a result, the amounts of rain water retention are greater than those from pine and oaks. The former explains the reason why interception in this species is higher, even in low values of total precipitation.
Even though the behavior of interception is similar in the oak and pine forests, it is evident that the interception curve in the pine forest is greater, which can be attributed to a larger density of the basal area, as well as height and average diameter. This statement is reinforced with the oberved behavior of the Abies forest in regard to the mensuration variables (Table 2).
When analyzing the rainfall interception percentages en the three cases, it is worth noting that they are consistent with the value obtained in different temperate forests (Aussenac, 1981). Interception values of 21.5 % have been documented for Pinus halepensis Miller and of 20.4 and 24.9 % for Quercus sessiliflora Salisb. (Aussenac, 1981), which are consistent with those recorded in the actual study, except for Abies. Cantú and González (2001) quote 13.5 % for Quercus in forests of Linares, Nuevo León State, Mexico, which differ from those indicated in this study, which is understood from the differnt climatic conditions.
According to more recent research studies, the results are consistent in terms of the behavior of the interception models (Hosseini et al., 2012; Yáñez-Díaz et al. 2014; Sadegi et al., 2015). It is important to highlight that the percentages here obtained suggest that other variables (Torres-Rojo, 2014), such as density, crown architecture and leaf morphology, among other things that were not analyzed, also participate in the rain interception process, and thus, need to be assessed.
Conclusions
Three models were obtained that adequately describe the values and behavior of rain interception for each dominant species considered. The evaluated characteristics allow, through the proposed models, the prognosis of the effects of the vegetal cover on the input of rainwater in the study area.
The three modules show different characteristics, in terms of species, altitude, exposure and micro-environmental conditions. Based on the differences in interception models, it is possible that other climatic, site or vegetation variables (allometric) need to be incorporated into the modeling of the interception process of rainfall by temperate forests.
Conflict of interests
The authors declare no conflict of interests
Contributions by author
Eulogio Flores Ayala: design and planning of the research, supervision of field work, data taking at the field, analysis of the application of results and writing of the document; Vidal Guerra De la Cruz: research support, field data analysis, structuring, presentation and writing of results in the document; Gerardo H. Terrazas González: statistical analysis and fitting of the generated models; Fernando Carrillo Anzures: field data taking, assessment of the aplicability of the information taken in the field and forestry interpretation; Fabián Islas Gutiérrez: assessment of the aplicability of the information taken in the field and review of the fit of the equations; Miguel Acosta Mireles: data taking at the field, research support, field data analysis, interpretation of the fit to the generated models, structuring, presentation and writing of results in the document; Enrique Buendía Rodríguez: field data review and depuration and presentation of results in the document.
Acknowledgements
The authors would like to express their gratitude to the ejidal authorities of the community of San Pablo Ixayoc, Texcoco municipality, State of Mexico, for their support and access facilities to their forests to carry out the present research work.
REFERENCES
André F., J Mathieu. and Q. Ponette. 2008. Effects of biological and meteorological factors on stemflow chemistry within a temperate mixed Oak-beech stand. Science of the Total Environment 393(1):72-83. [ Links ]
Aussenac, G. 1981. L’interception des précipitations par les peuplements forestiers. La Houille Blanche (7-8):531-536. [ Links ]
Cantú S., I. and H. González R. 2001. Interception loss, throughfall and stemflow chemistry in pine and oak forests in northeastern Mexico. Tree Physiology 21:1009-1013. [ Links ]
David, J., F. Valente and J. Gash. 2005. Evaporation of intercepted rainfall. In: Anderson, M. (ed.). Encyclopedia of Hydrological Sciences. John Wiley and Sons. West Sussex, England. pp. 627-634. [ Links ]
Fleischbein, K., W. Wilcke, R. Goller, J. Boy, C. Valarezo, W. Zech and K. Knoblich. 2005. Rainfall interception in a lower montane forest in Ecuador: effects of canopy properties. Hydrological Processes 19(7):1355-1371. [ Links ]
Flores, A. E., F. Becerra, L., E. Buendía, R., F. Carrillo A. y M. Acosta M. 2011. Intercepción de lluvia por vegetación forestal. Estado actual de la investigación en México e Hidalgo. Aportes para el desarrollo del estado de Hidalgo. In: Colegio del Estado de Hidalgo (comp.). Vol.1 Agua y Medio Ambiente. Ed. Miguel Ángel Porrúa. México, D.F., México. pp. 151-166. [ Links ]
Hosseini, G. B. , S. M., P. Attarod, V. Bayramzadeh, M. T. Ahmadi and A. Radmehr. 2012. Throughfall, stemflow, and rainfall interception in a natural pure forest of chestnut-leaved Oak (Quercus castaneifolia C.A. Mey.) in the Caspian Forest of Iran. Annals of Forest Research 55(2):197-206. [ Links ]
Kittredge, J. 1948. Forest influences. The effects of woody vegetation on climate, water, and soil, with applications to the conservation of water and the control of floods and erosion. Dover Publications, Inc. New York, NY, USA. 349 p. [ Links ]
Levia, D. F. and S. R. Herwitz. 2005. Interspecific variation of bark water storage capacity of three deciduous tree species in relation to stemflow yield and solute flux to forest soils. Catena 64: 117-137. [ Links ]
Lida, S., T. Tanaka and M. Sugita. 2005. Change of interception process due to the succession from Japanese red pine to evergreen oak. Journal of Hydrology 315: 154-166. [ Links ]
Manfroi O, K. Koichiro, T. Nobuaki, S. Masakazu, M. Nakagawa, T. Nakashizuka and L. Chong. 2004. The stemflow of trees in a Bornean lowland tropical forest. Hydrological Processes 18 (13): 2455-2474. [ Links ]
Marin, T. C., W. Bouten and J. Sevink 2000. Gross rainfall and its partitioning into throughfall, stemflow and evaporation of intercepted water in four forest ecosystems in western Amazonia. Journal of Hydrology 237:40-57. [ Links ]
Návar Ch., J. J., J. Méndez G. y H. González R. 2008. Intercepción de la lluvia en especies de leguminosas del nordeste de México. Terra Latinoamericana 26 (1):61-68. [ Links ]
Prasad G., C., L. Adrian Bruijnzeel, M. W. Lubczynski, M. Ravelona, B. W. Zwartendijk and H. J. (Ilja) van Meerveld. 2016. Measurement and modelling of rainfall interception by two differently aged secondary forests in upland Eastern Madagascar. Journal of Hydrology . doi: http://dx.doi.org/10.1016/j.jhydrol.2016.10.032. [ Links ]
Sadeghi S. M. M., P. Attarod and T. G. Pypker. 2015. Differences in rainfall interception during the growing and non-growing seasons in a Fraxinus rotundifolia Mill. plantation located in a semiarid climate. Journal of Agricultural Science and Technology 17 (1):145-156. [ Links ]
Statistical Analysis System Institute (SAS). 2007. The SAS System for Windows ver. 9.1.3. SAS Institute Inc. Cary, NC, USA. [ Links ]
Scatena, F. N. 1990. Watershed scale rainfall interception on two forested watersheds in the Luquillo mountains of Puerto Rico. Journal of Hydrology 13 (1990): 89-102. [ Links ]
Siegert, C. M. and D. F. Levia 2014. Seasonal and meteorological effects on differential stemflow funneling ratios for two deciduous tree species. Journal of Hydrology 519: 446-454. [ Links ]
Staelens J., A. D. Schrijver, K. Verheyen and N. E. C. Verhoest. 2008. Rainfall partitioning into throughfall, stemflow, and interception within a single beech (Fagus sylvatica L.) canopy: influence of foliation, rain event characteristics, and meteorology. Hydrological Processes 22 (1): 33-45. [ Links ]
Taghi A. M., P. Attarod andV. Bayramzadeh . 2013. The role of rainfall size in canopy interception loss: An observational study in a typical beech forest. Middle-East Journal of Scientific Research 3(7): 876-882. [ Links ]
Torres-Rojo, J. M. 2014. Exploring volume growth-density of mixed multiaged stands in northern Mexico. Agrociencia 48 (4): 447-461. [ Links ]
Yáñez-Díaz, M. I., I. Cantú-Silva, H. González-Rodríguez y J. I. Uvalle-Sauceda. 2014. Redistribución de la precipitación en tres especies arbustivas nativas y una plantación de eucalipto del noreste de México. Tecnología y Ciencias del Agua 5 (2):71-84. [ Links ]
Xiao, Q., E. G. McPherson, S. L. Ustin, M. E. Grismer and J. R. Simpson. 2000. Winter rainfall interception by two mature open-grown trees in Davis, California. Hydrological Processes 14(4): 763-784. [ Links ]
Received: February 02, 2016; Accepted: July 08, 2016











 texto en
texto en 





