1. Introduction

The elucidation of the origin of the spectra coming from magnetic white dwarfs and neutron stars is only possible if we understand the behavior of simple atoms and molecules in the presence of strong magnetic fields. Chemistry of molecules in strong magnetic fields is quite different from traditional chemistry. For example, in strong magnetic fields, the presence of exotic species of atoms and molecules is possible (the case of one-two electron systems made out of protons and/or alpha particles is reviewed in ¹ and ² and references therein). Exotic molecules in strong magnetic fields were predicted by Ruderman ^3,4 when he suggested that in strong magnetic fields linear chains of Hydrogen are formed along the magnetic field orientation. These predictions were motivated by the presumable existence of strong magnetic fields B ∼ 10¹² G in neutron stars. Currently, such magnetic fields in neutron stars are confirmed by observations, and there is evidence ⁵ that even stronger magnetic fields can appear in magnetars (B ∼ 10¹⁴⁻¹⁵ G). Since then, the study of molecules and atoms in strong magnetic fields has gained relevance. In particular, from the observation by the Hubble Space Telescope of Helium atmospheres in magnetic white dwarfs ⁶ and more recently, from observations by Chandra X-Ray Observatory in 2002 when they detected a considerable amount of data of thermal emission showing clear signals of absorption features from the surface layer of the isolated neutron star 1E1207.4-5209 ⁷, which is characterized by an enormous magnetic field B ≳ 10¹² G. This conclusion was supported in 2003 by observations of XMMNewton X-ray Observatory ⁸ (see also ⁹). The origin of these absorption lines in spectra is not fully understood yet. Simple spectral models demonstrate that the atmosphere of the 1E1207 object cannot be Hydrogen or ionized Helium in ultra-strong magnetic fields, but leading to the extreme conclusion that the presence of oxygen or neon can possibly explain the absorption features (see for example ¹⁰). Other possibilities led to the proposal of models based on the existence of simple exotic compounds like H3++ (see ¹¹ for example).

The simplest molecule H2+ has been widely and extensively studied by many authors with and without the presence of a magnetic field. In the absence of a magnetic field, the ground state (1g) is absolutely stable while the first excited state (1u), usually considered as unbound and purely repulsive, is characterized by a shallow Van der Waals minimum with a large inter-proton equilibrium distance (∼ 12 − 13 a.u.)ⁱ. Such minimum even supports the first vibrational level which remains stable towards dissociation ¹². This Van der Waals minimum remains stable even in the case of nuclei with finite masses (See ¹³).

In the presence of a uniform magnetic field the ground state remains stable for any orientation of the magnetic field, and the well becomes deeper as the magnetic field increases. The parallel configuration realizes the optimal configuration of minimal total energy. For larger magnetic fields, the domain of inclinations where the H2+-ion exists (where the minimal total energy lies below the energy at the dissociation limit), reduces as the magnetic field increases and finally becomes 0-25° at B = 4.414×10¹³ G (see ¹ and references therein).

The case of the 1u state in a magnetic field is by far, much less studied. The majority of studies were performed for the parallel configuration. Previous studies of this state in an arbitrarily oriented magnetic field only found stability when the molecular axis is oriented along the magnetic field lines and for relatively small strengths. In some cases, the accuracy of calculations has not allowed to make a definitive conclusion about the stability with respect dissociation and nuclear motion effects (see ¹).

The present study is focused to the 1u state of H2+ in presence of a uniform magnetic field in the range of strengths (B = 0 − 10a.u.). Our goal is to confirm the stability of this excited state when the system is interacting with an arbitrarily oriented constant magnetic field. We are particularly interested in knowing the optimal configuration of minimal total energy. We developed a variational study with a physics recipe for choosing variational trial functions (described in full generality in ¹⁴).

2. Hamitonian

The Hamiltonian which describes a system of two infinitely massive protons and one electron (ppe) (or H2+) placed in a uniform magnetic field B, is given (in atomic units) by

where p^=-i∇ is the momentum of the electron, 𝒜 is a vector potential corresponding to the magnetic field B = ∇ × 𝒜, r₁,r₂ are the distances between the electron and each proton, and R is the internuclear distance. The total energy of this system is defined as the total electronic energy plus the classical Coulomb repulsion energy between the protons. We will consider the general situation where the molecular axis is oriented along the z-axis interacting with a uniform magnetic field arbitrarily oriented forming an angle θ with respect to the molecular axis

A 2-parameter vector potential corresponding to the magnetic field (2) is given by

where λ₁ and λ₂ are two arbitrary parameters. Gauge (3) satisfies the Coulomb gauge (∇ · 𝒜(r) = 0). The symmetric gauge (𝒜 = (1/2)B × r) corresponds to the case λ₁ = −(1/2)cosθ and λ₂ = −(1/2)sinθ. For a magnetic field oriented along the z-axis (θ = 0°), the Landau gauge corresponds to λ₁ = −1 and λ₂ = 0. Gauge (3) includes a) the linear gauge 𝒜 = ((1 + λ₁)y, λ₁x, 0) if λ₂ = 0 for a magnetic field oriented parallel to the z-axis, and b) the linear gauge 𝒜 = (0, λ₂z,(1+λ₂)y) if λ₁ = 0 for a magnetic field oriented parallel to the x-axis. The parameters λ₁ and λ₂ will be treated a priori as independent variational parameters in the present study.

Using the gauge (3) defined by 2 parameters, the Hamiltonian takes the form

In the parallel configuration, the problem is characterized by the quantum numbers corresponding to: (i) spatial parity p and (ii) the angular momentum projection m on the magnetic field direction (z-direction). In cases of arbitrary inclination of the magnetic field, axial symmetry around z-axis does not exist, therefore only spatial parity p characterizes the system. The notation we use for the different states is based on the following convention: the first number corresponds to the number of excitation - “principal quantum number”, e.g. the number 1 is assigned to the ground state, the number 2 to the first excited state, and then a subscript g/u (gerade/ungerade) corresponding to positive/negative eigenvalues of the parity operator.

3. Method

Since we used an approximate method for solving the Schrodinger equation with the Hamiltonian (1), our approx-¨ imate energies can well be gauge-dependent (only the exact ones are gauge-independent). The present study is based on the variational method with the form of the vector potential chosen in a certain optimal way. The choice of trial functions is made according to the following recipe: As a first step, we construct an adequate variational real trial function Ψ₀^14,15,16,17, for which the associated potential V₀ ≡ (∆Ψ₀ /Ψ₀) reproduces the original potential near Coulomb singularities and the harmonic oscillator behavior (transverse to the magnetic field direction) at large distances. The trial function should support the symmetries of the original problem. We then performed a minimization of the energy functional by treating the free parameters of the trial function and the parameters λ₁ and λ₂ defining the vector potential on the same footing. Such an approach enables us to find the optimal form of the Hamiltonian as a function of λ₁ and λ₂. It is worth to emphasize one important assumption in the present study. Namely, that for a fixed value of B and a given inclination, we can find a gauge for which the eigenfunction is realⁱⁱ. This gauge optimization is nothing but a mechanism to justify the use of real trial functions. Therefore one can discard any imaginary term in the Hamiltonian. This prescription was used in ¹ and led to adequate trial functions to study the two lowest states of H2+ in strong magnetic fields B > 10⁹ G.

A key point in the present study is based on the observation that in the Landau problem (one electron in a constant magnetic field) with the magnetic field given by (2) and the corresponding vector potential (3), the exact (real) eigenfunction for the ground state is given by

where |ρ→'|| is the transverse distance between the electron position and the magnetic field line (see Fig. 1). For the parallel case (θ = 0°) the standard form, in the symmetric gauge, i.e. Ψ0Landau=e-(B/4)ρ2, where ρ is the radial cylindrical coordinate, is recovered.

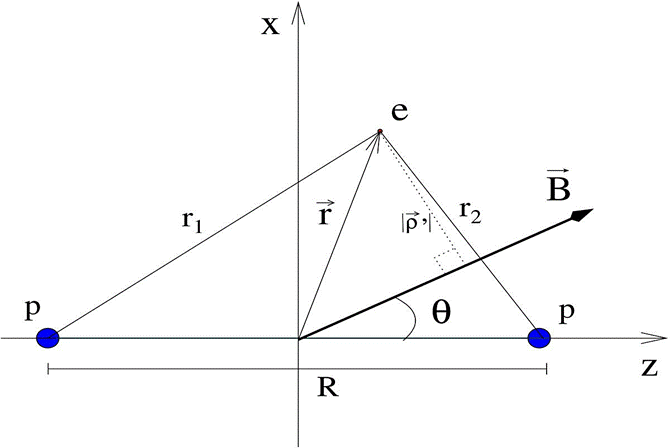
Figure 1 Geometrical setting for the H2+ molecular ion in presence of a magnetic field forming an angle θ with respect to the molecular axis and parallel to the x-z plane. The protons are situated along the z-axis separated by a distance R. The origin of coordinates coincides with the midpoint along the molecular axis, and it is chosen as the gauge origin. 

Motivated by the exact solution (5) for an electron in the presence of a constant magnetic field given by (2) we propose a trial function of the form

which is a (symmetric (gerade)/antisymmetric (ungerade)) function with respect to the interchange of the nuclei, of the Guillemin-Zener type multiplied by the Landau factor in the form (5). In (6) α₃, α₄, β_3x, β_3y, β_3z and β_c are variational parameters. So, this function is characterized by 6 variational parameters. The function (6) is a modification of the Guillemin-Zenner type function used in ¹. Due to the symmetrization the trial function (9) can be separated in two terms ψ_GZ−a,b. Following a criterion of physical adequacy described in ¹⁴, the associated potential (V = ∆Ψ/Ψ) corresponding to the first term ψ_GZ−a of the trial function (6) is

The dominant terms in the associated potential V_GZ−a reproduce the terms of the original potential in (4), for the symmetric gauge, if α₃ = α₄ = 1, β_3x = cos²θ, β_3y = 1, β_3z = sin²θ, β_c = 2cosθ sinθ. The associated potential contains additional terms which are sub-dominant near Coulomb singularities and at large distances. It is important to mention that if the parameters β_c = β₃ = 0, then the associated potential reproduces the potential and, correspondingly, the trial function used in ¹. This situation occurs in the configurations of maximal symmetry 0° and 90°.

The Guillemin Zener type function (6) reduces to the particular cases of Heitler-London type, and Hund-Mulliken type functions, when α₃ = α₄ and α₃ = 0, respectively.

4. Results

We carried out a variational study of the 1u state of the system (ppe) with infinitely heavy nuclei, interacting with a magnetic field 0 < B ≤ 10 a.u. in inclined configuration 0° ≤ θ ≤ 90° with respect to the molecular axis. In this range of magnetic fields the total energy of the 1u state presents a pronounced minimum. Our variational results indicate that there exist some critical fields and critical inclinations where the 1u becomes unstable towards dissociation H2+→H+p. This is described in more details below.

The numerical calculation was developed using subroutines D01FCF of NAG-LIB ¹⁹ for the 2(3) dimensional integrations (depending to the symmetry of the configuration) and the package MINUIT of CERN-LIB ²⁰ to minimize the energy functional. In order to reach a higher (and stable) accuracy, the (finite) domain of integration was manually and conveniently subdivided following the profile of the integrand. Usually, the domain of integration along each coordinate was subdivided into 3 or more subregions. The variational results are presented with an estimated relative accuracy of 10⁻⁶.

4.1. Configurations of maximal symmetry

The results of our variational calculations with the trial function (9) for the 1u state of H2+ in the presence of a uni form magnetic field, in maximal symmetry configurations θ = 0°; 90°, are presented in Table I and Table II, respectively.

Table I Total, E_T, binding, E_b = E_e −E_T, energies and equilibrium distance R_eq for the state 1u as a function of magnetic field B in the parallel configuration θ = 0° which becomes 1σ_u. This state represents the first excited state or the ground of state of negative parity. The optimal gauge is found to correspond to the symmetric gauge (λ₁ = −1/2,λ₂ = 0) for all magnetic fields. The ground state of energy of the Hydrogen atom 1s₀²⁷ is presented in the last column for comparison. The horizontal line divides the fields where the 1u is stable/unstable. 

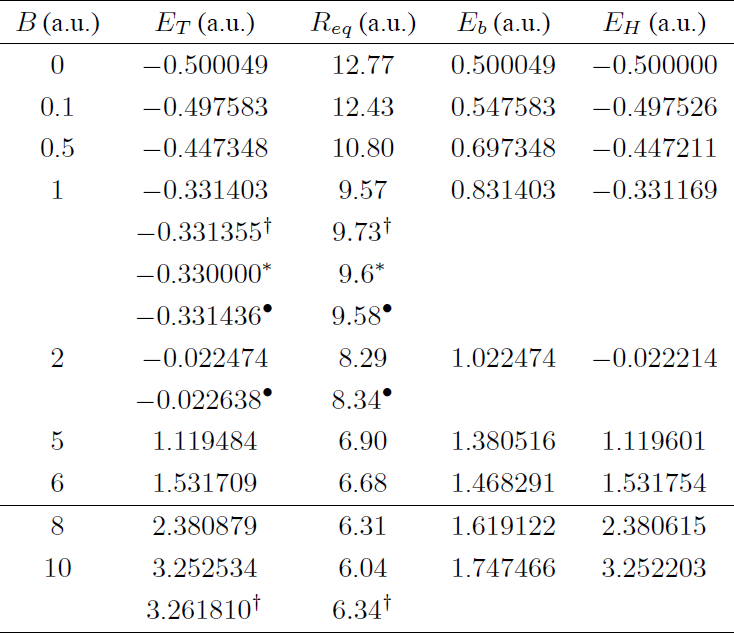
^† results from ¹.

^∗ results from ²⁴.

• results from ²⁵.

Table II Total, E_T, binding, E_b, energies and equilibrium distance R_eq for the state 1u as a function of magnetic field B in the perpendicular configuration θ = 90°. This state represents the first excited state or the ground of state of negative parity. The optimal gauge is found to correspond to ∼ Landau gauge (λ₁ = 0,λ₂ = −0.08) for all magnetic fields. The ground state of energy of the Hydrogen atom 1s₀²⁷ is presented in the last column for comparison. The horizontal line divides the fields where the 1u is stable/unstable. 

† Results from ¹.

In the parallel configuration our results for the total energy of the 1u state are in excellent agreement with the best results ^{21,22,23,24,25}. For example, for B = 1 a.u. the difference with respect to the best known results existing in the literature ²⁵ is beyond 10⁻⁵ a.u. Qualitatively, we observed a monotonous increase in the total E _T and bindinⁱⁱⁱ energy E _B , accompanied by a monotonous decrease in the equilibrium internuclear distance R_eq as a function of magnetic field (0 ≤ B ≤ 10 a.u.) It is worth emphasizing that in this particular configuration, our results improve slightly the results from ¹ with a simple addition of one Guillemin-Zener type function (the improvement was ∼ 10⁻⁵ for B = 1 a.u.). However, such a small difference may be enough to confirm or discard stability in some cases. The fact that we obtained a small improvement in the total energy, even adding one more Guillemin-Zener term to the trial function (i.e. 5 more variational parameters), can be interpreted as a manifestation of the convergence of the trial function. However, the obtained equilibrium distance R_eq = 9.57 a.u. at B = 1a.u. shows a better agreement with results in ^24,25 (see Table I).

The 1u state in the perpendicular configuration has received less attention in the literature. The only exceptions are references ^{24,25,26 iv}. In the perpendicular configuration, the system also exhibits an increasing behavior of the total and binding energies as a function of the magnetic field, accompanied by a monotonous decrease in the equilibrium internuclear distance (see Table II). For all the magnetic fields considered, the total energy for the 1u state in the perpendicular configuration is always larger compared with the energy in the parallel configuration. Also, for all magnetic fields studied, we observe that in perpendicular configuration the system is more compact (the internuclear equilibrium distance is smaller) in comparison to the parallel configuration (e.g. by ∼ 2.3a.u. at B = 1a.u.)

One of the main goals of the present study is to find the optimal configuration (of lowest total energy) for this state. From our variational results, we confirm the conjecture that the parallel configuration realizes the configuration of minimal total energy of the H2+ molecular ion in the 1u state for all magnetic fields considered in the present study (see Tables I and II). In particular, for B = 1 a.u. the minimal total energy in the parallel configuration is in agreement with adiabatic potential energy calculations carried out in ²⁴ where a basis set of ∼ 360 generalized optimized atomic orbitals was used. It is worth to notice that for the optimal (parallel) configuration our 20-parameter trial function (9) provides a better (lower) total energy (by ∼ 1.4 × 10⁻³ a.u.) in comparison to ²⁴.

The difference between the energies of maximal symmetry configurations increases as a function of the magnetic field (∆E = E_T (90°) − E_T (0°) = 0.8 a.u for B = 1 a.u.), such behavior is interpreted as the 1u state becomes more rigid towards rotations as the magnetic field increases.

4.2. Stability of 1u state: Critical Fields

One can observe from Table I and Table II that the 1u state is not stable for all the magnetic fields. First of all, it is important to emphasize that a high accuracy was required in order to analyze the stability the 1u state, since it is a weakly bound state and the potential well is very shallow. The present variational results show that the energy of the 1s ground state of Hydrogen atom and the total energy of the 1u state of H2+ in the parallel configuration are very close. Therefore, it is necessary to analyze the dissociation energy (see Fig. 2).

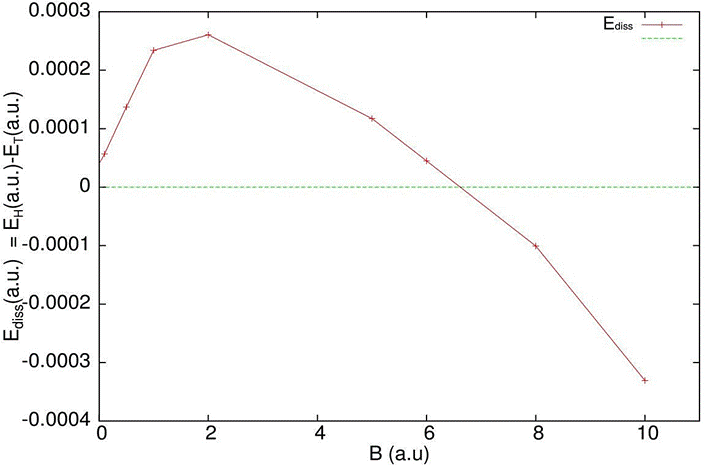
Figure 2 Dissociation energy E_diss = E_H −E_T of the 1u state of H2+ at equilibrium as a function of the magnetic field B in the parallel configuration (θ = 0°). The curve E_diss vs B exhibits a domain of magnetic fields where the 1u state of H2+ is stable with respect to dissociation to Hydrogen atom plus proton (positive dissociation energy). The range of this domain goes from B = 0 a.u to B_cr ∼ 6.6 a.u. 

Our variational results show that in the parallel configuration the 1u state is stable towards dissociation H2+→H+p for B < 8a.u. In Fig. 2 the dissociation energy is plotted as a function of the magnetic field. In such plot we can see that there is a critical value of the magnetic field where the 1u state becomes unstable towards dissociation (the dissociation energy turns negative), such critical field is estimated at ∼ 6.6 a.u. by a simple interpolation. This critical field defines a domain of magnetic fields where the 1u state remains stable towards dissociation.

As for the parallel configuration we can do a similar analysis for the perpendicular case. In this configuration the 1u state is only stable for magnetic fields B < 0.1 a.u. Thus, the domain of stability in the perpendicular configuration is quite smaller compared with that of the parallel configuration. The critical magnetic field is estimated at B = 2 × 10⁻³ a.u.

In both configurations, for all the magnetic fields considered, the 1u state exhibits a pronounced minimum in the potential energy curve, but only in those magnetic fields for which the global minimum of energy at finite R_eq is below the total energy of the Hydrogen atom in the ground state (see Fig. 3 for example for B = 1a.u) the system is considered to be stable. The deepest well in the parallel configuration occurs for a uniform magnetic field of ∼ 2 a.u. that represents the maximal stability of the system (maximum of dissociation energy as a function of the magnetic field) (see Fig. 2).

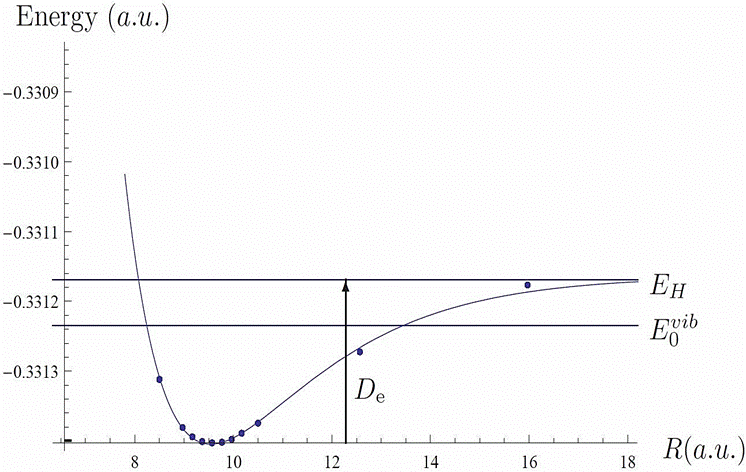
Figure 3 Total energy (dots) of the 1u state of H2+ as a function of the internuclear distance R at the magnetic field B = 1 a.u. in the parallel configuration θ = 0°. The curve represents the Morse fit to the variational results. The upper horizontal line represents the asymptotic energy i.e. the total energy of the ground state 1s₀ of Hydrogen atom E_H = −0.331169a.u. at B = 1a.u. (see ²⁷). The horizontal line below the asymptotic energy represents the first vibrational state (E0vib = 0.00014a.u. with respect to the equilibrium energy). The total energy at equilibrium is E_T = −0.331403a.u. at R_eq = 9.57a.u. 

5. Inclined Configurations

5.1. Stability of 1u state: Critical Angles

In the present study, we also considered the analysis of inclined configurations 0° < θ < 90° using the trial function (9). It is important to mention that for arbitrary inclinations of the magnetic field there are almost no references in the literature. The only exception is ²⁶ where the qualitative description is very different from the behavior obtained in the present study. We consider that such differences do not allow for a direct comparison with the present results^v and that independent calculations are needed in order to resolve this disagreement.

Our variational results are presented in Tables III and IV for the magnetic fields B = 0.1 a.u. and B = 1 a.u. respectively.

Table III Total energy E_T, and equilibrium distance R_eq for the state 1u at different orientations θ of the magnetic field with respect to the molecular axis for the magnetic field B = 0.1a.u. We estimate that the uncertainty in R_eq is ∆ ≃ 0.05a.u. due to the fact that the potential well is too flat. The total energy of the ground state of Hydrogen atom at B = 0.1 a.u. is −0.497527 a.u. 

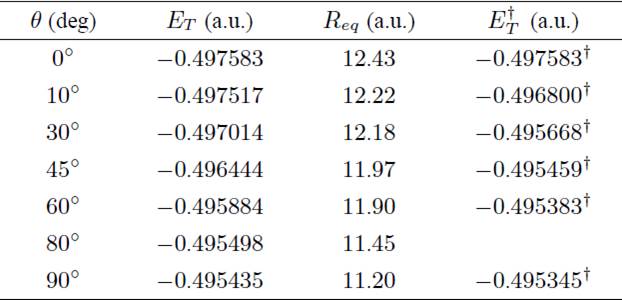
† results from ¹.

Table IV Total energy E_T, and equilibrium distance R_eq for the state 1u at different orientations θ of the magnetic field with respect to the molecular axis for the magnetic field B = 1a.u. We estimate that the uncertainty in R_eq is ∆ ≃ 0.05a.u. due to the fact that the potential well is too flat. The total energy of the ground state of Hydrogen atom at B = 1a.u. is −0.331169a.u. 

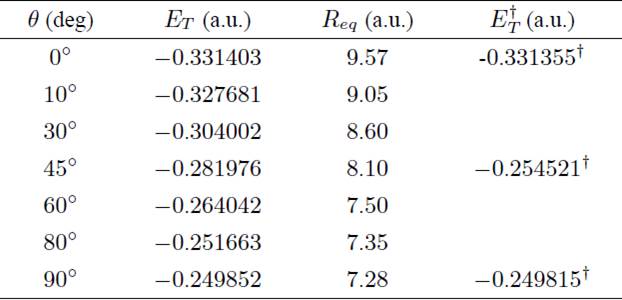
† results from ¹.

As we expected the maximal symmetry configurations are found to correspond to the extremals of energy: the parallel configuration is the optimal configuration of total energy, while the perpendicular configuration corresponds to the maximum of total energy (though it also corresponds to the most compact configuration).

The behavior of the total energy of 1u state of (ppe)-system for both representative magnetic fields is monotonously increasing as a function of the inclination angle θ of the magnetic field, while the equilibrium distance R_eq decreases monotonically (see Tables III, IV and Figs. 4, 5).

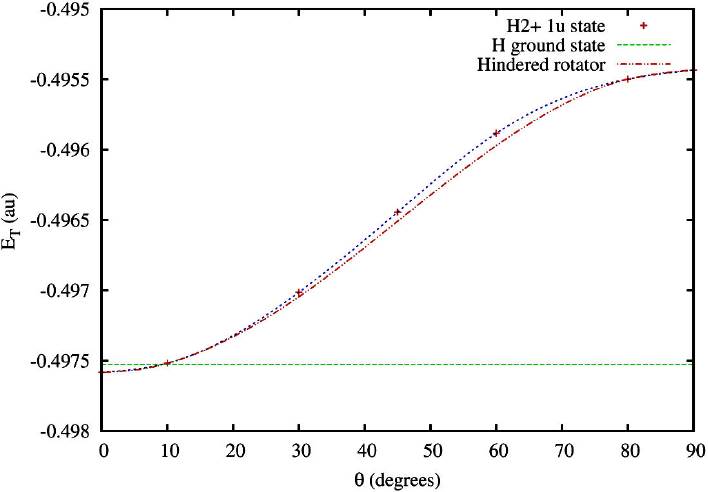
Figure 4 Total energy E_T of the 1u state of H2+ at equilibrium as a function of orientation θ at a magnetic field B = 0.1 a.u. The horizontal line represents total energy of the separate system H+p (i.e. the energy of the Hydrogen atom at B = 0.1 a.u.). The orange curve represents the Hindered Rotor Model. The curve exhibits a domain of orientations where the 1u state of H2+ system is stable with respect to dissociation. The critical angle for which the system becomes unstable is estimated at θ_cr ∼ 9°. 

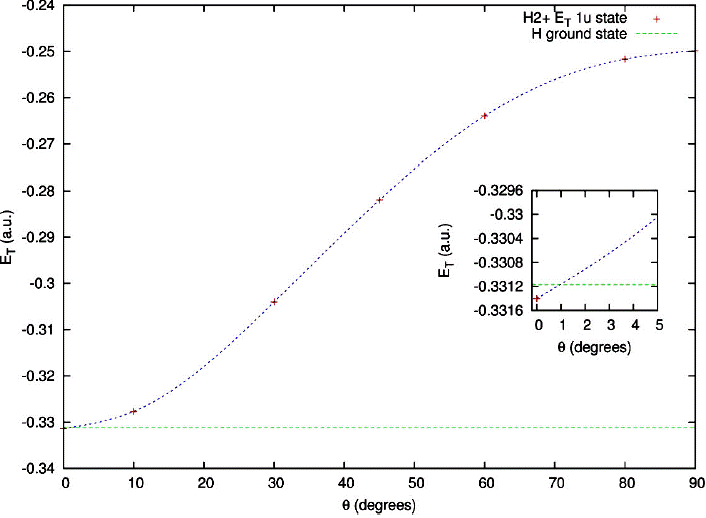
Figure 5 Total energy E_T of the 1u state of H2+ as a function of orientation θ with respect to the molecular axis for the magnetic field B = 1a.u. The horizontal line represents total energy of the separate system H + p (i.e. the energy of the Hydrogen atom at B = 1a.u.). The curve exhibits a domain of orientations where the 1u state of H2+ system is stable with respect to dissociation. The critical angle for which the system becomes unstable is estimated at θ_cr ∼ 1°. 

For both magnetic fields, the 1u state of the (ppe)-system becomes unstable towards dissociation H2+→H+p from a certain critical orientation (see Figs. 4 and 5). Critical angles were found at θ_cr ∼ 9 for B = 0.1 a.u and θ_cr ∼ 1 for B = 1 a.u. This non-negligible decreasing of θ_cr as a function of the magnetic field corresponds to the increase in the total energy difference between the maximal symmetry configurations. Though the improvement to the total energy using the trial function (9) is relatively small in comparison to the total energy found in ¹, the change in the critical angle is quite significant. This qualitatively different result implies that the 1u state is more stable towards rotations.

It is worth to observe from Fig. 4 that our variational results as a function of the inclination angle θ fit well at a hindered rotator model

V(R,θ)=V(R,0)+ΔV(R)sin2θ , (14)

where ∆V (R) = V (R,90°)−V (R,0°) is the barrier height for a given value of R. Such behavior would allow us to make a similar rotational analysis as it was done in ²⁸ for the ground state 1g (this will be done elsewhere).

Using our variational results, it is possible to construct a surface of energy as a function of the molecular distance R and the magnetic field orientation θ (see Fig. 6 for B = 1a.u). The potential energy surface appears smooth and without indications to any other local minimum.

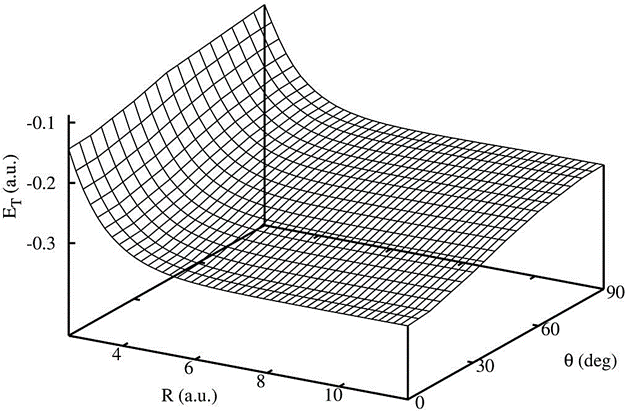
Figure 6 Total energy E_T (in a.u.) of the 1u state of H2+ as a function of the internuclear distance R (in a.u.) and the orientation θ (in degrees) with respect to the molecular axis at the magnetic field B = 1a.u. The potential surface exhibits a global minimum at R = 9.56a.u. and θ = 0°, and shows that the configurations of maximal symmetry correspond to the extremals of energy. 

Additionally, we made an analysis of the behavior of the expectation values 〈x²〉, 〈y²〉, 〈xz〉 as well the gauge parameters λ₁,2, with respect to the orientation angle θ for a magnetic field B = 1a.u. (see Fig. 7 and Table V) as obtained with the trial function (9). From these values, we can compute the variation of the total energy of the 1u state through Eq. (10). Both expectation values hx²i and hy²i increase/decrease monotonously as a function of the inclination angle. On the other side, the expectation value hxzi vanishes in the configurations θ = 90° due to the symmetry z → −z. This corroborates the result predicted by the Hellman-Feynman theorem, i.e. the variation of the total energy becomes zero only in the configurations of maximal symmetry. The analysis also confirms that the optimal gauge for the parallel configuration θ = 0° corresponds to the symmetric gauge, while for the perpendicular configuration θ = 90°, the Landau Gauge seems to be more adequate.

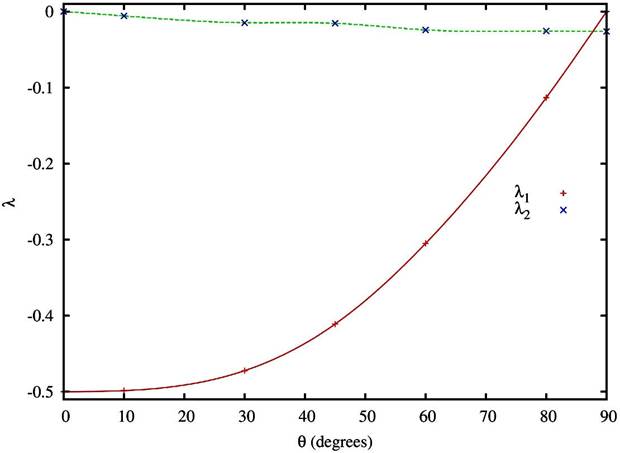
Figure 7 Optimal gauge variational parameters λ as a function of the orientation θ for the magnetic field B = 1 a.u. In the parallel configuration (θ = 0°) the optimal gauge corresponds to the symmetric gauge (λ₁ = −1/2, λ₂ = 0). In the perpendicular configuration (θ = 90°) the optimal gauge is very close to the Landau gauge (λ₁ = 0, λ₂ = −0.08).  

Table V Expectation values, optimal gauge parameters λ_1,2, and the variation of the total energy (10) as a function of the orientation θ corresponding to the trial function (9) for the magnetic field B = 1a.u. 

6. Conclusions

We found a range of magnetic fields where the 1u state of H2+ with infinitely massive protons is stable in both (θ = 0° and 90°) configurations of maximal symmetry. With the improved trial function (6) proposed in this study we found in the parallel configuration a domain of stability for magnetic fields 0 ≤ B ≲ 6.6a.u. This range of magnetic fields overlaps with the range of magnetic fields typically present in magnetic white dwarfs. The maximal stability for this state is realized at B ∼ 2a.u. Up to magnetic fields B = 1a.u. the first vibrational state remains stable towards dissociation. Additionally, the trial function (6) describes a larger range of stable orientations θ for two representative magnetic fields: θ_cr ≃ 9° for B = 0.1a.u. and θ_cr ≃1° for B = 1a.u. From the optimization of the vector potential, we confirm that both gauge parameters λ₁,2 are relevant to obtain the minimal energy in configurations of arbitrary orientation of the magnetic field, despite the fact that λ₂ is relatively small. We confirmed the theoretical predictions by Hellman-Feynman theorem that the energy extrema correspond to the configuration of maximal symmetry: the minimum corresponds to 0° and the maximum corresponds to 90°.

From an astrophysics point of view, the stability of the 1u state can be of relevance for the analysis of the spectra of white dwarfs. For example, in the magnetic field B = 1a.u. the energy corresponding to the transition between the two lowest states of H2+ is 3.9 eV.

7. Note added in proof

While this manuscript was under consideration the authors performed an analysis for the ground state 1g in magnetic fields 0.1a.u. ≤ B ≤ 1 a.u., using a linear superposition of three functions of the type (6) and found an improvement in the variational energy (for intermediate angles 0° < θ < 90°) which is in excellent agreement with the hindered rotor model approximation (14) used in the reference ²⁸. It is worth to mention that the agreement between the hindered rotor model and the variational results using the functions of the type (6) is much better in the case of the ground state 1g. This results will be reported elsewhere.