Introduction

The family of ordered-vacancy compounds (OVC’s) with the general formula Mn◻B2IIIX4VI, with B: Al, Ga or In; and X: S, Se, or Te, where ⃞ symbolizes a cation vacancy, or simply MnB2IIIX4VI, has received considerable interest in the last three decades [¹-¹²]. These magnetic compounds, which have three metal cations (one Mn and two B’s) around each anion site (X) while the fourth empty site forms an ordered array of vacancies, could exist in different crystal structures and have shown several notable physical and chemical properties associated with the crystallographic ordered array of these vacancies. In addition, the presence in these OVC’s of magnetic Mn⁺² ions make them of great interest from the point of view of their magneto-optical properties. Thus, magnetic properties of MnIn₂Te₄, MnGa₂Se₄, MnIn₂Se₄; which crystallize in tetragonal defect-chalcopyrite (space group I4-2m) [²,³], defect-thiogallate (s. g. I4-) [⁷], and cubic spinel (s. g. Fd3-m) [¹²] structures at ambient conditions, respectively, and its alloys, have been studied in detail for several authors [²-⁸,¹³-¹⁵]. MnIn₂Se₄, a member of this family which crystallizes in a layered rhombohedral structure (s. g. R3-m) [¹⁶,¹⁷], has also been studied with considerable interest. Measurements of magnetic susceptibility as a function of temperature show antiferromagnetic behavior with paramagnetic Curie temperature of about −96 K and the formation of spin-glass-like phase below about 3.6 K [⁶,¹⁴,¹⁵]. The occurrence of this spin-glass transition is confirmed by ac in-phase magnetic susceptibility measurements close to the spin freezing transition [¹⁴]. Here, the typical downward shift in the freezing temperature T _f , when the frequency is decreased, was observed. The zero-frequency transition temperature is T _f = (3.58 ± 0.02) K. On the other hand, analysis of its optical absorption coefficient spectra [¹,¹⁸] reveals that the lowest-energy-gap of this compound is indirect between parabolic bands that vary from 1.55 to 1.43 eV in the temperature range from 10 K to 295 K [¹⁸]. This indirect band-to-band transition arises from the uppermost Γ₄(z) valence band at Γ point, to the conduction band minimum located at a lower-symmetry point different from Γ [12]. Additionally [¹⁸], two direct band-to-band transitions, beginning at 1.72 and 1.85 eV, at 295K, and at 1.82 and 1.96 eV, at 10 K, from the uppermost Γ₄(z) heavy-hole and middle Γ₅(z) light-hole bands, to the Γ₁(s) conduction band, were observed in the high energy region. At energies around 2.2 eV, an internal transitions from ⁶A₁ ground state to the ⁴ T ₁ d-excited level of Mn⁺² ion, was also observed from photoluminescence spectra.

A semiconductor property which is affected by the presence of Mn⁺², especially at low temperatures, is the band gap E _G . Theoretical studies have shown [¹⁹,²⁰] that nearby a critical point, as for example the spin glass transition temperature T _f , a magnetic contribution to the variation E _G with temperature, due to the exchange interaction between the d electrons of Mn⁺² ions and the electrons in the valence and conduction bands, should occur. For antiferromagnetic exchange interaction, as is the case of MnIn₂Se₄, an increase in E _G around T _f is expected [¹⁹]. In spite of these advances, to the best of our knowledge, a detailed study of the temperature dependence of E _G for semiconductors where a paramagnetic to spin-glass transition occurs, has not been reported. This is of interest in relation to whether it is a true phase transition from a thermodynamic point of view or a gradual freezing of the magnetic moments. For this reason, in the present work we present a study of the temperature dependence of the fundamental band gaps in MnIn₂Se₄. This dependence is discussed in terms of the theoretical models reported in the literature that analyze the magnetic contribution to the shift of E _G with T.

2. Crystal growth and experimental details

Bulk single-crystals of MnIn₂Se₄ were grown by chemical vapor transport technique from polycrystalline MnIn₂Se₄ which was synthesized from stoichiometric amounts of 5 N pure Mn, In, and Se, in an evacuated and closed ampoule. This was carried out by gradual heating of the mixture, subsequent melting, and gradual cooling through the freezing point. For the crystal growth, a 20 cm long and 1.5 cm inner diameter quartz stationary ampoule loaded with 1.5 g charge of the pre-synthesized material was used. I ₂, with a concentration of 4 mg/cm³ of ampoule volume, was employed as transport agent in the reaction. The transport reaction was carried out in a two-temperature zone furnace during seven days between 800^oC, in the highest-temperature region of the ampoule where the source pre-reacted material is initially placed, and 750^oC, in the lowest-temperature region where the obtained bulk crystals are deposited. As-grown bulk crystals have typical dimensions of about 20-30 mm² and show a dark color.

A SIEMENS D-5005 diffractometer, operated at 30 kV and 15 mA, with a Cu-target (λ−Cu Kα = 0.15418 nm) tube, a graphite monochromator and a scintillation detector, was used to register the powder X-ray diffraction pattern at room temperature. The intensity data were recorded in a θ/θ reflection mode from 10 to 100^o with a step size of 0.02^o and a scan speed of 25 s/step. Quartz was used as an external standard.

The absorption coefficient spectra α was obtained from the optical transmittance T _r spectra through the relation [²¹] T _r = [(1 − R)² exp(−αx)]/[1 − R ² exp(−2αx)], where x is the thickness sample and R ≈ 0.223 [¹⁸], is the reflectivity. T _r spectra were measured by using an Acton Research Spectra P-500 spectrometer in the energy range from 1.2 to 2.4 eV. For the measurements of these spectra at various temperatures from 10 to 295 K, the samples were mounted on a cold finger of a Janis closed cycle refrigerator.

3. Powder X-ray diffraction analysis

To determine the crystal structure and unit cell parameters of MnIn₂Se₄, powder X-ray diffraction analysis was carried out. The structure was refined by means of the Rietveld method using the Fullprof program. For the refinement, atomic coordinates of MnIn₂Se₄ single-crystal reported by Range et al. [¹⁷], where used in the analysis. The Rietveld plot is shown in Fig. 1. The final refinement converged to the profile agreement factors: R _p = 9.9 %, R _wp = 10.5 %, R _exp = 9.1%, S = 1.2. MnIn₂Se₄ crystallizes in the centrosymmetric space group R3-mD3d5, Z = 3, with unit cell parameters: α = 0.4055(1), c = 3.9467(2) nm, c/α = 9.733, and V = 0.5620(2) nm³. These values are in good agreement with those reported in [¹⁶,¹⁷] and also shown in Table I.

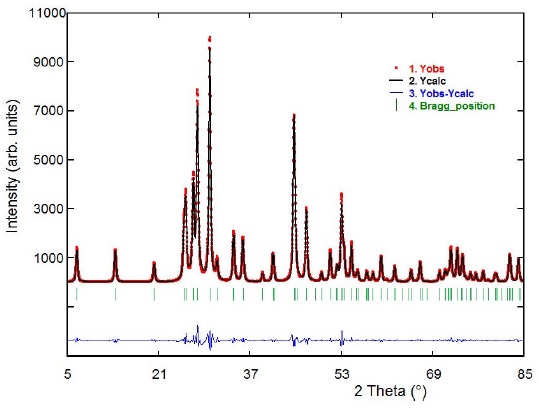
FIGURE 1 Observed (circles), calculated (solid line), and difference plot of the final Rietveld refinement of MnIn₂Se₄. 

The crystallographic analysis confirms that MnIn₂Se₄ belongs to a rhombohedral defect structure with tetrahedral and octahedral cation-to-anion coordination, which can be expressed by the chemical formula [M]_oct([M]_tetr)₂Se₄, where [M] means either Mn and In cations distributed in a disordered arrangement. [M]_oct being the disordered cations occupying the octahedral sites, six-fold coordinated by Se anions, while [M]_tetr being these cations occupying the tetrahedral sites, four-fold coordinated by Se anions.

The unit cell diagram of MnIn₂Se₄ is shown in Fig. 2. A close-packed Se layer, with Mn and In cations at random in octahedral and tetrahedral voids, leaving the interlayers between h-stacked layers vacant, can be observed in this figure.

FIGURE 2 The rhombohedral structure of MnIn₂Se₄ with octahedral (purple) and tetrahedral (green) polyhedra. Mn, In, Se atoms and cation vacancies, are represented by green, orange, yellow and white spheres, respectively. 

4. Temperature dependence of the band gap energy

The nature of the fundamental absorption edge in MnIn₂Se₄ was examined by us in a previous article [¹⁸] by studying the optical absorption coefficient spectra α _c of this compound at several temperatures between 10 and 295 K. It was found, at values of α _c lower than about 2 × 10³ cm⁻¹, that the lowest energy gap is indirect. On the other hand, for values of α _c greater than 2 × 10³ cm⁻¹, the absorption coefficient spectra show the presence of two direct band-to-band transitions at higher energies. These two processes, with characteristic energies EGDI and EGDII, correspond [18] to direct transitions from the uppermost Γ₄(z) and the middle Γ₅(z) valence bands, to the Γ₁(s) conduction band of MnIn₂Se₄, respectively.

The shape of E _G vs. T curve in non-magnetic semiconductors is approximately flat at low temperatures, but smoothly changes to a linear decrease in the high temperature region. However, as can be seen in Fig. 3, where the temperature variation of E _GI , EGDI and EGDII energy gaps of MnIn₂Se₄ in the form of solid circles are plotted, this is not observed in the present case where going to low temperatures, the curves also shown a nearly linear variation with T.

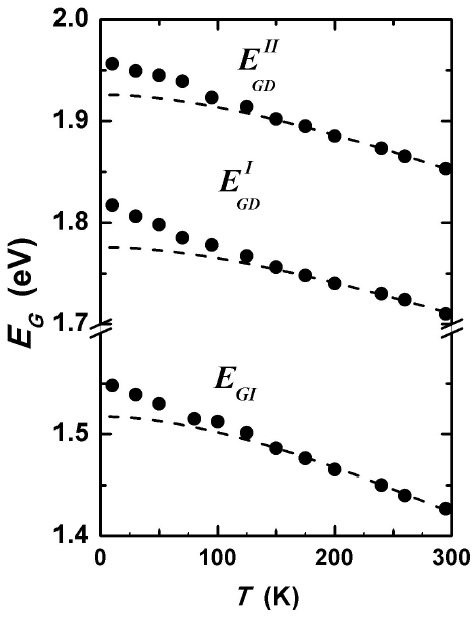
FIGURE 3 Temperature variation of E _GI , EGDI and EGDII energy gaps of MnIn₂Se₄. Solid circles (•) and broken lines represent the experimental points and the fit to the experimental curves in the high-temperature region (T > 150 K) by using Eq. (5), respectively. 

Such a behavior at low T, which has also been observed in other materials having antiferromagnetic exchange [²²,²⁴], is also consistent with the theoretical study [¹⁹] about the effects of spin fluctuations on the shift of the E _G with temperature around a magnetic critical temperature T _C . According to this study, for an antiferromagnetic exchange interaction between spins, a magnetic contribution is expected that should cause an increase ∆E _GM in the band gap of the semiconductor in the vicinity of the spin-glass transition temperature T _f .

To describe the behavior of E _G near the critical freezing temperature T _f in terms of a power law, the reduced temperature difference τ = |T − T _f |/T _f , which is zero at the phase transition, is introduced in the analysis of the data [¹⁹]. For a semiconductor with antiferromagnetic exchange interaction, the theory indicates that ∆E _GM satisfies a relation of the form

where λ is the critical exponent of the band-gap variation ∆E _GM and A is a parameter that measures the strength of the total spin interaction on this variation.

Two different temperature regions should be taken into account in this analysis. The critical region, near the spinglass freezing temperature T _f , where the main contribution to ∆E _GM vs. T arises from short-range spin correlations, and the non-critical one, far from T _f , (T > Tf) where longer-range effects turn out to be important. Kasuya and Kondo [²⁵], when studying the anomalies of the electrical resistivity in antiferromagnetic materials that exhibit orderdisorder second-order phase transitions, have found that in the critical region λ is equal to the critical index of the specific heat magnetization α, which has a value close to zero. On the other hand, for τ outside the critical region (at T > T _f ), Alexander et al. [¹⁹] predict that the value of exponent λ should be 1/2. Analyses of experimental E _G vs. T data for various diluted magnetic semiconductors showing antiferromagnetic behaviour [²²-²⁴] confirm the predictions of both these models.

In order to obtain a theoretical expression for ∆E _GM that can be compared with the data, Eq. (1) should be integrated.

then, if the value of ∆E _GM at T _f is expressed as ∆E _f , the constant of integration C in Eq. (2) can be removed and this equation can be written as:

Thus, values of the magnetic shift ∆E _GM determined from the experimental data can be analyzed in terms of Eq. (4). To calculate ∆E _GM it is necessary to know the values of E _G in the absence of any magnetic effects. These can be determined by extrapolating to low temperatures the E _G (T) curves at high temperatures where the magnetic effects are unimportant. This may be done by using the expression for the E _G vs T variation based on Bose-Einstein phonon model, given by [²⁶]

where E _B −α _b = E _G (0) is the value of the energy gap at 0 K, and Φ an average phonon related to the Debye temperature.

The difference between the measured curves and those extrapolated by using Eq. (5) is thus attributed to the magnetic contribution∆E _GM . Hence, the data shown in Fig. 3 have to be fitted to Eq. (5) in the high-temperature region (T ≥ 150 K). Thus, in order to reduce the number of the free parameters to be determined from the data, i.e. E _B , α _B , and Φ, to only two, we have taken Φ = 200 K, which is the Debye temperature for MnIn₂Se₄ estimated from its melting point (T _M ≈ 1178 K) by using the classical Lindemann expression, which relates Φ to the melting point T _M through the expression Φ ∼ TM ^1/2 [²⁷]. Hence, using Φ ≈ 200 K as a fixed parameter, Eq. (5) was fitted to the experimental E _GI (T), EGDI (T), and EGDII (T) data of Fig. 3, in the temperature range from 150 to 300 K. Values of E _G (0) and a _B , obtained from this fit are also given in Table II. Theoretical extrapolated E _GIE (T), EGDEI (T), and EGDEII (T) curves, obtained from Eq. (6), are also shown in Fig. 3 by broken lines. The magnetic shift can thus be obtained as ∆E _GM (T) = E _G (T)−E _GE (T), for these three curves. The value of ∆E _G at T _f ≈ 3.6 K for each of the curves in Fig. 3, as obtained directly from these curves, was 34, 39 and 33 meV, for E _GI (T), EGDI(T), and EGDII(T), respectively.

The temperature variation of ΔE_GM for the three band-to-band transitions in the form of a plot of ln(ΔE_GM-ΔE_G) vs. ln τ, in the temperature range where the magnetic contribution to E_G vs. T is appreciable, is shown a broken line, in Figs. 4, 5a, and 5b, for E_GI, EGDI, and EGDII, respectively. It can be seen that in the low τ region all the curves show a linear behaviour, in good agreement with Eq. (4). The slope (1-λ) in this temperature region is found to be 0.98, 0.92, and 0.91 (±0.02), for E _GI , EGDI, and EGDII curves. These give λ _c ≈ 0.02, 0.08 and 0.09 for these curves, respectively. This is also shown in Table II. These values are in good agreement with theoretical analysis that predicts λ ≈ 0 in the critical region [²⁵]. In addition, in the high-τ region, the slope of the line is found to be 0.54, 0.51, and 0.48 (±0.02). This gives λ _nc ≈ 0.46, 0.49, and 0.52 (±0.02) for these curves, respectively, which is also in agreement with the theory that predicts λ _nc ≈ 0.5 in this region [¹⁹].

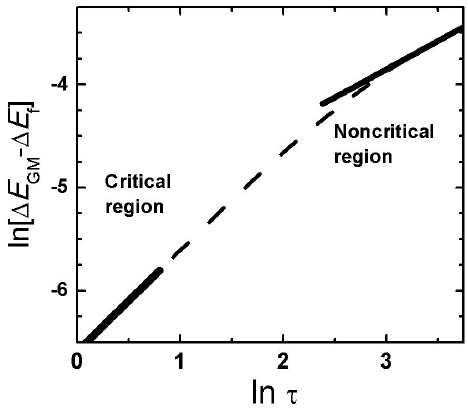
FIGURE 4 Temperature variation of ∆E _GM for the indirect bandto-band transition E _GI observed in MnIn₂Se₄ in the form of a plot of ln(∆E _GM − ∆E _f ) vs. lnτ, in the temperature range where the magnetic contribution to E _G vs. T is appreciable (T < 150 K) (broken line). The slopes in both low and high-τ regions are indicated by bold continuous straight lines. 

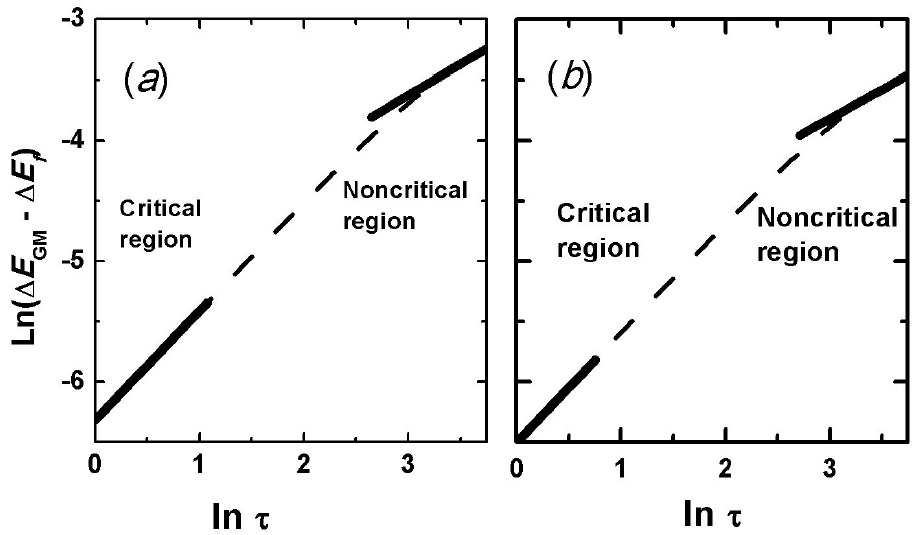
FIGURE 5 Temperature variation of ∆E _GM for the indirect band-to-band transition GGDI and GGDII observed in MnIn₂Se₄ in the form of a plot of ln(∆E _GM − ∆E _f ) vs. lnτ, in the temperature range where the magnetic contribution to E _G vs. T is appreciable (T < 150 K) (broken line). The slopes in both low and high-t regions are indicated by bold continuous straight lines  

However, it is also observed that an intermediate temperature region exists where λ which was obtained from the slope of the broken lines at each temperature in Fig. 5, gradually increases between these two values. This is shown in Fig. 6 where the variation of λ with lnτ is plotted for the EGDI(T) optical transition. Values of λ for two alloys of Cd _x Zn _y Mn _z Te pseudo-ternary system, a material for which a transition from antiferromagnetic-to-spin glass phase has been observed [34], are also shown in this figure for comparison.

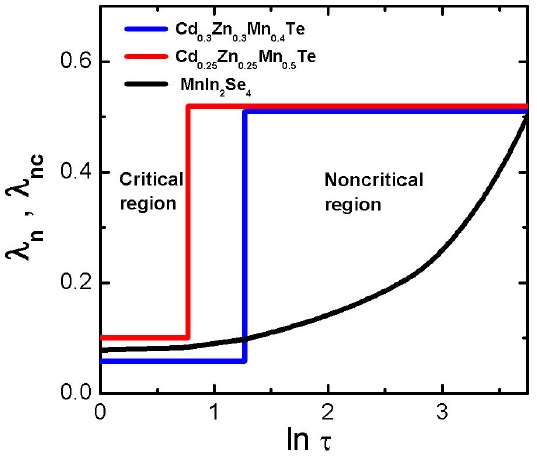
FIGURE 6 Variation of λ¸ obtained from the slope of broken lines in Fig. 5, with ln τ in the critical and noncritical regions for the direct transition EGDI in MnIn₂Se₄ (black line). Values of λ¸ for the lowest band gap of Cd_0:3Zn_0:3Mn_0:4Te (blue line) and Cd_0:25Zn_0:25Mn_0:5Te (red line) Cd_xZn_yMn_zTe pseudo-ternary alloys, taken from [22] are also shown. 

The existence of this intermediate region, which does not appear in ideal antiferromagnetic compounds where an abrupt change when passing from critical to noncritical regions is observed [²²-²⁴], indicates that in the present case a gradual change is occurs when MnIn₂Se₄ pass from the spinglass phase to the paramagnetic one. This fact reveals that it is not a true phase transition in the thermodynamic sense but a gradual freezing of the magnetic moments.

Thus, it is found that in MnIn₂Se₄ below about 20 K, short-range spin correlations give the main contribution to ΔE_GM vs. T, while longer-range effects becomes dominant at temperatures between about 70 to 150 K. In the intermediate temperature region, where λ¸ varies from about 0.02 to 0.40, both these two spin correlation effects should be taken into account in the analysis of magneto-optical properties of this compound.

Values of the parameters A in the critical and non-critical regions, obtained from the fit are also shown in Table II. These values of A _c and A _nc , are in good agreement with those reported for antiferromagnetic semiconductors [22,24] that vary from 0.2 to 28.0 and 4.8 to 18.0 meV, respectively.

5. Conclusion

The temperature dependence of the indirect and direct fundamental band gaps of single crystals on the layered compound MnIn₂Se₄, that crystallize in the rhombohedral defect structure with space group R3-mD3d5, were studied by optical absorption spectra. The data were analyzed in terms of the theoretical models by Alexander et al. [¹⁹] and Kasuya and Kondo [²⁵] that takes into account the magnetic contribution to the shift of E _G with T. It is suggested that short-range effect spin correlations dominate the contribution to the shift below about 20 K, in the critical region of the paramagnetic to spin-glass phase transition, whereas longer-range effect spin correlations control the contribution to this shift in the temperature range from about 70 to 150 K, in the noncritical region. An intermediate temperature region, where a gradual freezing of the magnetic moments occurs, was also observed. This probably indicates that, from a thermodynamic point of view, the spin-glass phenomenon is a gradual freezing of the magnetic moments instead of a true phase second order transition.