Introduction
Wood normally shrinks as it dries and swells as it absorbs moisture. These changes in their dimensions are of importance to determine the service life condition of wooden materials. Even if sometimes the swelling and shrinking of wood can be used as an advantage (swelling is employed to close seams in barrels, tubs, tanks, and boats), they result in drying deformations, dimensional variations in wooden structures and ageing of coatings (Perré, 2007). These particularities of wood are due to its hygroscopic behavior which is linked with some important wood properties such as density and mechanical strength which changes significantly as a response to moisture variations. Timber twist is caused when wood does not shrink uniformly in all directions when dried. In general, cells get slimmer, but not much shorter, when they lose moisture. Therefore, timber shrinks least in the grain direction and more in radial and tangential directions. Rays act as restraining rods to reduce radial shrinkage, so most shrinkage is developed along tangential direction (Ramage et al. 2017).
Several previous studies have tried to understand relationships among wood shrinkage values, wood species, tree growing conditions, wood density, material direction, position within the tree, and position within the growth rings. Because of the lack of knowledge and published data for several years, explaining shrinkage in relation to its anatomical features is still a challenge (Redman, Bailleres, & Perré, 2011; Leonardon, Altaner, Vihermaa, & Jarvis, 2010; Kelsey, 1963; Mariaux & Narboni, 1978). In past studies, wood shrinkage has been explained by various parameters such as density, humidity (Kelsey, 1963; Kollmann & Côté, 1968), ring width, tree age (Polge, 1964), microfibril angle (Barber & Meylan, 1964), wood ray proportion (Keller & Thiercelin, 1975) and radial parenchyma cells (uniseriate and multiseriate rays) (De la Paz, Dávalos-Sotelo and Quintanar-Isaias, 2005). Kollman and Côté (1968) demonstrated that shrinkage differs in three different directions due to the influence of wood rays as well as due to different arrangements of fibrils on the cell wall. In the case of transverse anisotropy, for example, the presence of rays is often evoked as a predictive parameter for Transverse Anisotropy Ratio for Shrinkage [TARS] (Barkas, 1941; Kawamura, 1984, Gu, Zink-Sharp, & Sel, 2001). According to previous studies (Lindsay and Chalk, 1954), wood rays have an important influence on radial shrinkage. It has been shown that radial shrinkage of hardwood species with no large woody rays is higher than that of hardwoods with large rays (Schniewind & Kersavage, 1962; Wijesinghe, 1959). These past studies also highlighted that radial shrinkage of isolated wood rays is lower than that of adjacent tissues composed only of longitudinal elements. They supply further evidence for Bosshard’s view (1956) that anisotropic shrinkage of wood is due to at least two separate factors and demonstrates that, although rays do have a restricting effect on radial shrinkage, there is also some inherent cause of anisotropic shrinkage in the fibers. The relative importance of these factors appears to vary considerably in different species, particularly through differences in the rate of radial shrinkage of the rays. Shrinkage varies widely in wood material cut from the same species, and even in material cut from the same tree. Sapwood generally shrinks less than heartwood. The springwood of an annual ring shrinks less transversely and more, longitudinally than the summerwood of the same ring (Peck, 1957). According to Schniewind (1959), the difference in shrinkage values between the early wood and latewood would contribute to accentuate the wood TARS in the transversal plane. A new cambium layer marks a new growth season. In Quercus wood species, this growth stage is rapid and large cells with thin walls are formed and, in latewood (the cells that grow later in the season, normally during summer) cells have thicker walls, are tightly packed and smaller than the cells that grow during spring. The parallel arrangement of the springwood (early wood) to the summerwood (late wood) tangentially in the annual rings may account for some of the difference between tangential and radial shrinkages (Ritter & Mitchell, 1952). Summerwood shrinks more tangentially than springwood, because it is denser and stronger than springwood, the summerwood also forces the springwood to shrink more than it would if it were detached from the two adjoining bands of summerwood. The relative position of the springwood and summerwood, however, does not affect radial shrinkage.
Table 1 shows the impact of the type and size of rays on radial and tangential wood shrinkage in the initial and final wood of Quercus kelloggii, according to Schniewind (1959) and Botosso (1997).
Table 1 Radial and tangential shrinkage of Quercus kelloggii wood, according to wood position within the tree (initial and final wood) and their wood ray composition (according to Schniewind, 1959 and Botosso, 1997).
| Quercus kelloggii wood specimen characteristics | Shrinkage values (%) | |
| Radial | Tangential | |
| Isolated wood rays | 2.1 | 6.6 |
| Initial wood | ||
| Without large woody rays | 3.2 | 5.2 |
| With large woody rays | 3.1 | 7.8 |
| Final wood | ||
| Without large woody rays | 4.5 | 7.9 |
| With large woody rays | 3.3 | 9.2 |
However, some researchers disagree with the influence of these rays on wood shrinkage anisotropy, especially on softwood species (Bosshard, 1956; Boutelje, 1962).
Both type and size of wood rays are also important parameters in wood shrinkage (De la Paz, Dávalos-Sotelo and Quintanar-Isaias, 2005). Kawamura (1979) showed that large-sized woody rays increase the shrinkage anisotropy of oak wood, while smaller (uniseriate) woody rays have a smaller impact on their TARS. This phenomenon could be explained by the alignment of microfibrils (Skaar, 1988) and by presence of the wood ray’s parenchyma cells which have a high microfibril inclination relative to their principal axis. Keller and Thiercellin (1975) found that the higher the mean unit size of large rays, the smaller the wood radial shrinkage. According to Guitard and El Amri (1987), this correlation agrees with the fact that a larger volume fraction of wood rays decreases radial shrinkage to the detriment of an increase in tangential shrinkage. These results reinforce the hypothesis that wood rays are at least partially responsible for the difference between tangential and radial hardwood shrinkage (Skaar, 1988). This phenomenon was explained by the lower hygroscopic behavior of woody ray tissues (lower Fiber Saturation Point) than that of the longitudinal tissues of the wood and by the chemical composition of wood rays (Morschauser & Preston, 1954), more particularly by the lignin and extractive contents (Boyd, 1974). All of this points out that predicting shrinkage requires additional knowledge of the relationships between wood anatomical structure and shrinkage properties. Generally, properties and morphology need to be defined at a given scale to predict shrinkage at the upper scale.
Objectives
The objectives of this present study were (i) to determine the physical properties of various ring porous wood species (ii) and to evaluate the influence of wood rays (according to their proportions and volume measured by image analysis) on physical properties of wood and more particularly on the longitudinal, radial shrinkage and TARS. This study focuses on four hardwood species differing by the presence and abundance of their multiseriate wood rays: Castanea sativa Mill, Quercus canariensis Willd, Quercus petraea (Matt.) Liebl. And Quercus robur L.
Materials and methods
Tree selection
The work focused on four hardwood ring-porous species with variable wood-ray characteristics. Castanea sativa Mill, Quercus canariensis Willd, Quercus petraea (Matt.) Liebl. And Quercus robur L. were chosen for their similar anatomical structure apart from their wood rays characteristics and proportions. Q. canariensis belongs to the Section Mesobalanus and Q. petraea and Q. robur to the Section Leucobalanus. In addition, these wood species have a very important economic aspect in France and Tunisia.
Table 2 and figure 1 show the anatomical structure characteristics and provenances of the studied woods.
Table 2 Hardwood ring-porous species studied according to their anatomical characteristics and provenances.
| Scientific names | Common names | Anatomical properties | Provenances |
| Castanea sativa Mill. | Sweet chestnut | Contains only uniseriate and rarely multiseriate wood rays. | Private forest of « Basses Cevennes à Chataigners » Southern France 44°45’0” N, 1°25’59.99” E |
| Quercus petraea (Matt.) Liebl. | Sessile oak | Contains uniseriate and multiseriate wood rays. Multiseriate wood rays are mostly abundant, up to 1 mm wide (up to 30 cells) and up to 5 cm high, frequently absent in young shoots. | Forest of Brin-sur-Seille (Northeastern France) 48°46’59” N, 6°21’0” E |
| Quercus robur L. | Pedunculate oak | Contains uniseriate and multiseriate wood rays. Multiseriate wood rays are abundant, up to 1mm wide (up to 30 cells) and up to 5 cm high, frequently absent in young shoots. | Forest of Brin-sur-Seille (Northeastern France) 48°46’59” N, 6°21’0” E |
| Quercus canariensis Willd. | Zeen oak | Contains uniseriate and multiseriate wood rays. Multiseriate wood rays are very abundant and have large sizes in width. | Aïn Draham area (Northern Tunisia) 36°46′34″ N, 8°41′05″ E |
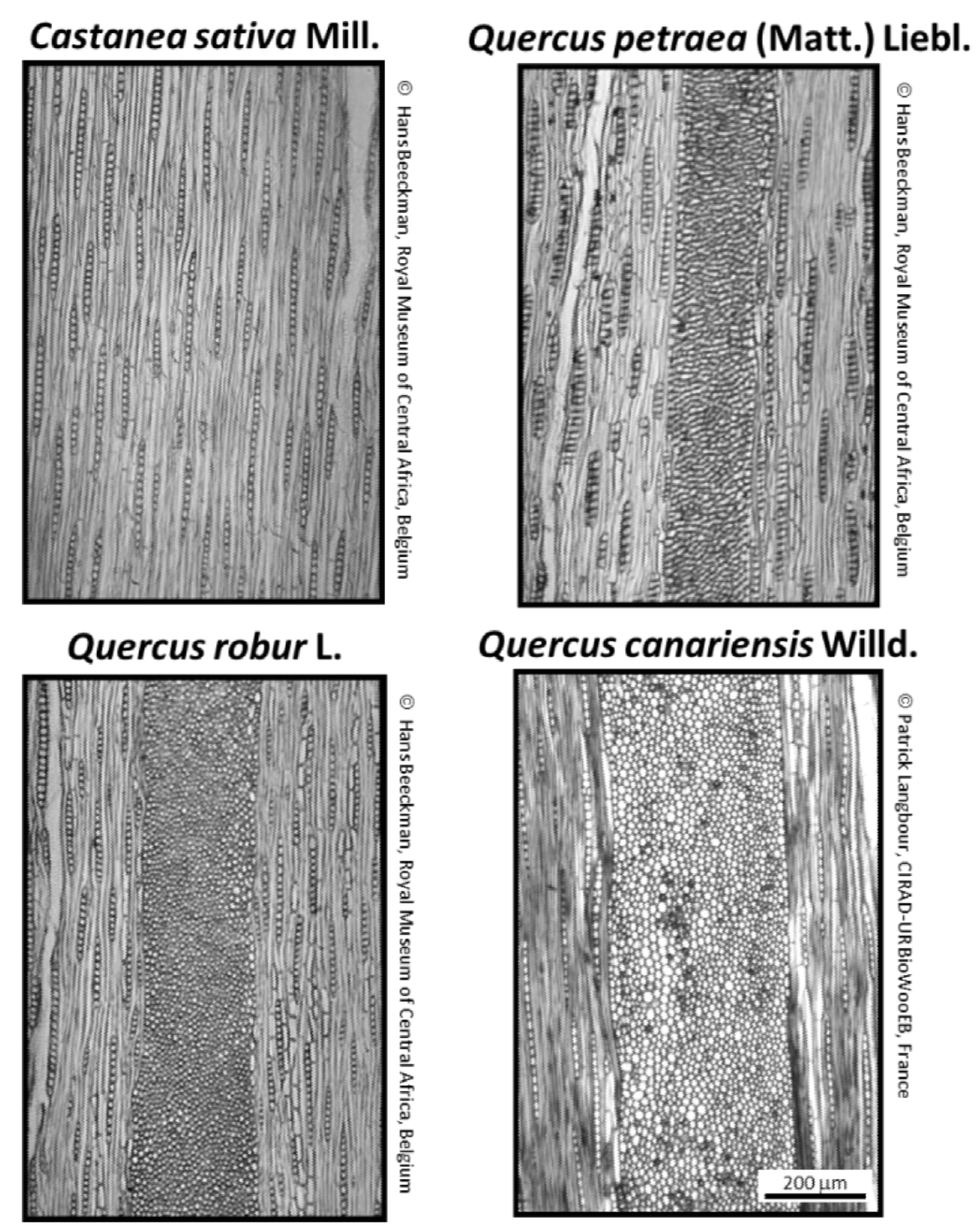
Figure 1 Microscopic views of the tangential sections of the four hardwood ring-porous species studied [the scale used is the same for the 4 photos].
For each wood species, six mature trees (Table 3) were selected from their respective forest areas and cut to specimen in order to characterize wood rays (number, width and proportions), basic density and shrinkage.
Table 3 Average values of annual rings width and tree age of the hardwood ring-porous selected trees.
| Wood species | Average value of annual rings width (mm) | Average value of tree age (years) |
| Castanea sativa Mill. | 3.03 ± 0.70 | 63 ± 12 |
| Quercus petraea (Matt.) Liebl. | 1.70 ± 0.42 | 80 ± 18 |
| Quercus robur L. | 2.05 ± 0.33 | 82 ± 16 |
| Quercus canariensis Willd. | 1.81 ± 0.30 | 85 ± 22 |
Wood sampling
To perform physical tests, one wooden disk 20 cm in thickness was cut at 1.30 m from the ground for each selected tree. The following parameters were measured for each wooden disk (six disks per wood species): ring width, sapwood width, eccentricity of the heartwood, tension-wood proportion, knots (size, shape and orientation) and other flaws in the wood (sap stain, resin pockets, wounds, insect attack, decay, crowning (upside warping), etc.).
As shown in figure 2, 12 wooden sticks (20 mm × 20 mm × 200 mm, right to left - RTL) were collected diametrically opposed from each wooden disk [four specimens from sapwood and eight specimens from heartwood]. Then, twin specimens (20 mm × 20 mm × 20 mm and 20 mm × 20 mm × 5 mm, RTL) were cut out from these sticks to determine radial and tangential shrinkage and basic density, respectively.
Wood ray characterization
For each wood specimen from C. sativa, Q. canariensis, Q. petraea and Q. robur, the proportion of multiseriate wood rays was determined as a percentage. To do so, an experimental device developed in the laboratory was used, composed of a digital camera coupled with a magnifying glass and connected to a computer with image analysis software (Fig. 3). Wood specimens were attached to a holder with their Tangential-Radial (LR) side facing the digital camera.
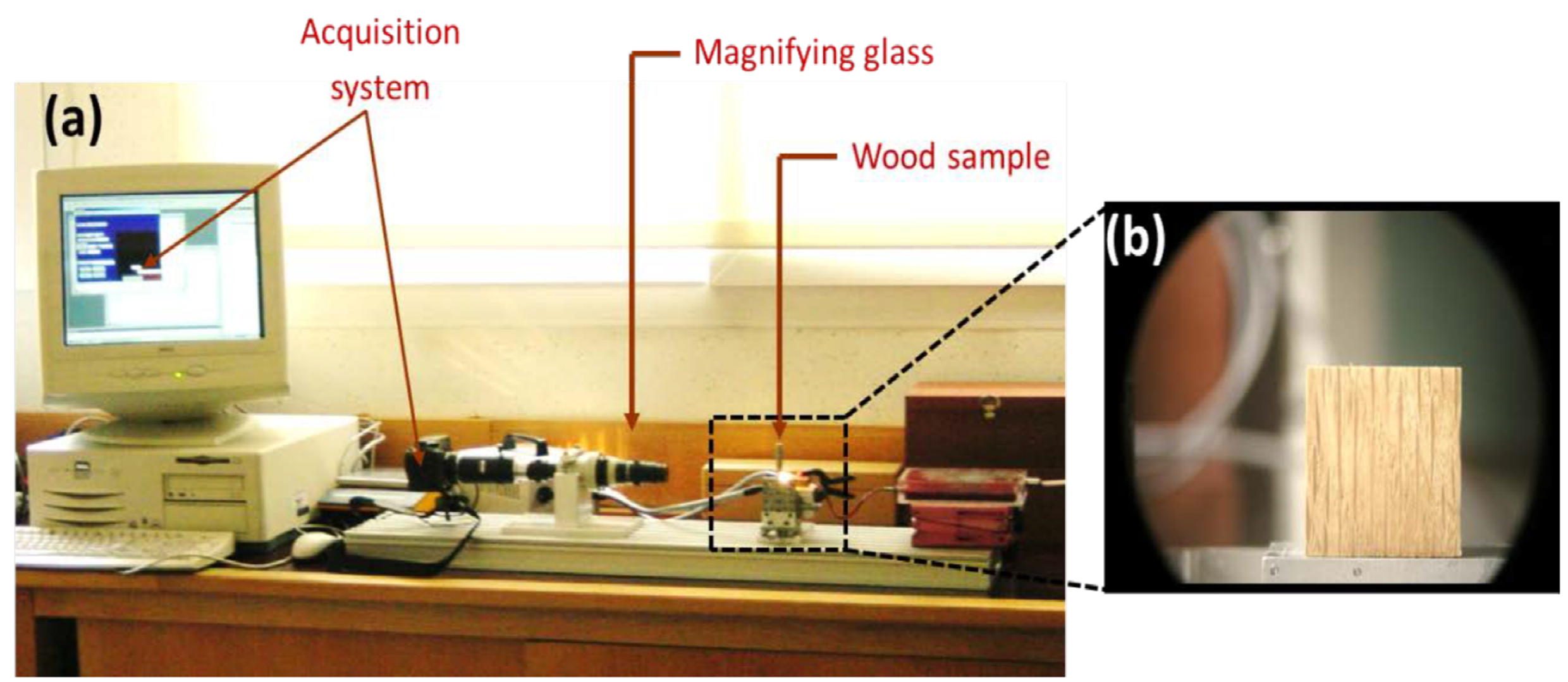
Figure 3 (a) Image analysis system (Microvideomat de Zeiss) for characterizing multiseriate wood rays, (b) LR face of wood specimen during the analysis.
Once the specimen image was taken, Photoshop® software was used to transform this image into a gray level (Fig. 4) in order to calculate the woody rays’ area in relation to the total surface area of the LR face of the wood specimen analyzed (Eq. 1).
where:
WR |
= Wood rays |
WRSA |
= Wood ray surface area (mm2, black) |
WSLRSA |
= Wood specimen LR surface area (mm2, grey) |
This process was repeated on 72 specimens from heartwood (36 for heartwood 1 and 36 for heartwood 2, Fig. 2) and 72 specimens from sapwood from each wood species studied in this work, in order to observe the variation of wood ray proportions evolution from pith to bark of the tree.
Basic density
The basic density (Db) of each wood specimen (20 mm × 20 mm × 5 mm, RTL) was determined by the gravimetric method described by Haygreen and Bowyer (1996), given by the following equation (Eq.2):
Where
Db |
= basic density of wood |
m0 |
= the oven-dried mass of the wood specimen (g) |
Vh |
= saturated volume of the specimen (cm3). |
For each wood species, basic density was measured on 72 specimens from heartwood (36 for heartwood 1 and 36 for heartwood 2, Fig. 2) and 72 specimens from sapwood, in order to observe the wood basic density variation from pith to bark of the tree.
Shrinkage
o avoid errors during sampling, extreme cases such as excessively knotty trees or the presence of reaction wood or slope grain were excluded from the sample (ISO 4471, 1982). Tangential (βt) and radial (βr) shrinkage was determined for each wood specimen (20 mm × 20 mm × 20 mm, RTL) according to the ISO 4469 (1981) standard. The TARS was then determined by the equation 3. TARS is used to express wood shrinkage anisotropy; the closer the TARS is to 1, the more the wood deformations due to drying are close to isotropic.
For each wood species, all shrinkage measurements were performed on 72 specimens from heartwood (36 for heartwood 1 and36 for heartwood 2, Fig. 2) and 72 specimens from sapwood, (36 for heartwood 1 and 36 for heartwood 2, Fig. 2), in order to study the wood shrinkage properties variation from pith to bark of the tree.
Results
Basic densities
Basic Density (Db) was estimated for the four hardwood species. The average values are presented in figure 5, for each species. The results show the significant relation of ring-porous hardwood species with their respective basic densities.
The average values of basic density were 0.460, 0.550, 0.580 and 0.690 for Castanea sativa, Quercus petraea, Quercus robur and Quercus canariensis, respectively. According to the anatomical properties, presented in figure 1 and Table 2, basic density seems to be correlated with wood ray proportions. These results are consistent with a previous study conducted by Boyces, Kaeiser, Bey (1970), who also indicated that ray proportion of black walnut wood (hardwood) was positively correlated with its specific gravity. Taylor (1969) found a similar tendency for Quercus rubra and Quercus alba woods highlighting that the increment in ray proportion contributes to the increase in wood basic density.
Figure 6 shows the average values of wood basic density according to position within the tree, for the four wood species studied.
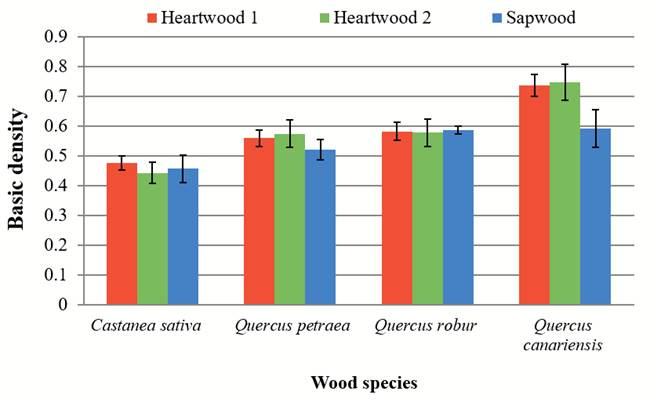
Figure 6 Average values of basic densities (Db) of each hardwood ring-porous species, according to the wood specimen position within the tree.
In addition, no significant difference between the basic density of heartwood 1 and heartwood 2 was observed. However, from heartwood to sapwood specimens, basic density seems to decrease for each of the ring-porous hardwood species studied, except for Quercus robur which present a similar density for its heartwood and its sapwood. However, this tendency is not really significant, and more data could be required to confirm this. Nevertheless, these results are similar to those obtained from other studies conducted on oak wood. For example, Cinotti (1991) studied the wood basic density repartitions in Quercus petraea and found average values of 0.589 and 0.570 and 0.526 for pith, heartwood and sapwood, respectively. Studying the wood from various Quercus species in France, Courtoisier (1976) found basic density values varying from 0.414 to 0.515 for sapwood specimens, and from 0.439 to 0.600 for heartwood specimens. The variability of density values from heartwood to sapwood may be explained by (i) the increase in wood rays as the tree grows and (ii) a higher content of tannins and many other extractives in heartwood than those contained in the of sapwood (Ayobi, Kiaei, & Bakhshi, 2011).
Shrinkage
According to the shrinkage analyses, the results illustrated in figure 7 show that the tangential (βt) and radial (βr) shrinkage and (TARS) values for the four wood species vary widely in material cut from the same wood species, and even in material cut from the same tree. In addition, shrinkage values, obtained in this study, for sapwood were lower than those obtained for heartwood, confirming previous statements from Peck (1957). It appears clear that TARS values are characteristic for each wood species. Moreover, and according to figure 7(c), TARS seems to be correlated with the wood anatomical properties and more particularly to ray size, as it was well stated by McIntosh (1955), who found that the presence of large woody rays limits radial shrinkage in Quercus rubra wood. Bosshard (1974) also highlighted the influence of wood rays in wood shrinkage anisotropy. Why wood shrinks more tangentially than radially has never been explained satisfactorily. It was thought that wood-ray cells restricted radial shrinkage because the length of the wood ray cells lies in a radial direction. It has been found, however, that the structure of wood ray cells permits large lengthwise shrinkage (Ritter & Mitchell, 1952). Although wood-ray cells shrink more lengthwise than the adjacent cells, this shrinkage is less than the radial shrinkage of the other cells (McIntosh, 1955; Morschauser & Preston, 1954). Consequently, the wood-ray cells exert a restraining effect on radial shrinkage and then TARS increased according to the wood ray proportion within the wood. The lower density of wood rays than those of other wood fibers could explain this tendency of the wood-ray cells in restraining radial shrinkage. The difference between tangential and radial shrinkage may be caused by the inflection of crystallites near the pits, since they occur on the radial faces of the wood fibers (Gross, Clark, & Ritter, 1939). However, other explanations have been put forth, such as the difference between fibril angles in radial and tangential walls (Yang, Ilic, Evans, & Fife, 2003), differences between the thickness of the primary walls in the radial and tangential directions, and the difference among the number of cross walls along the radial and tangential axes (Peck, 1957). Gu et al. (2001) state that the fibril arrangement (i.e. their packing density) on cross sections of the cell walls, especially of the thick S2 layer, contributes considerably to the different hygroexpansion/shrinkage of wood in the radial and tangential direction. For the radial variation of ray height, Lev-Yadun (1998) found that wood rays height was less than four cells near the pith, increasing with age, and had no general direct relationship with growth-ring width. Giraud (1977) mentioned that wood ray height increases with distance from the pith, in a study of Entandrophragma utile, a tropical Meliaceae. The ray area level of wood tends to increase according to its position in the tree, from the heart to the outer part of the heartwood. This rate of ray then remains approximately constant in the outer part of the tree. This stated variation of wood ray proportion along the distance of wood from the tree pith, could explain the fact that the results obtained show a decrease of wood radial shrinkage from heartwood to sapwood excepted with Castenae, the only species with uniseriate rays (Fig.7b).
Discussion
Influence of basic density (Db) on wood shrinkage intra wood species
For all wood species studied, basic density revealed a small but not significant wood variability within the tree, more particularly between sapwood and heartwood. More data could be required to confirm this. Similar results were observed on the four studied wood species. In addition, the density of ring porous woods is mainly dependent on growth ring structure and its variability and less on structural and chemical changes as a result of heartwood formation processes (Tsoumis, 1991).
Only results obtained from Q. canariensis are detailed below in order to facilitate the visibility of the results. In Q. canariensis wood, some extreme values of basic densities were found from 0.813 near the pith to 0.530 in specimens from wood near to the bark. In addition, each trunk had a similar density profile. Significant difference was observed between heartwood and sapwood specimens where sapwood specimens had low tangential and radial shrinkage values whereas heartwood specimens were characterized by higher shrinkage values in both directions (Fig. 8 a, b).
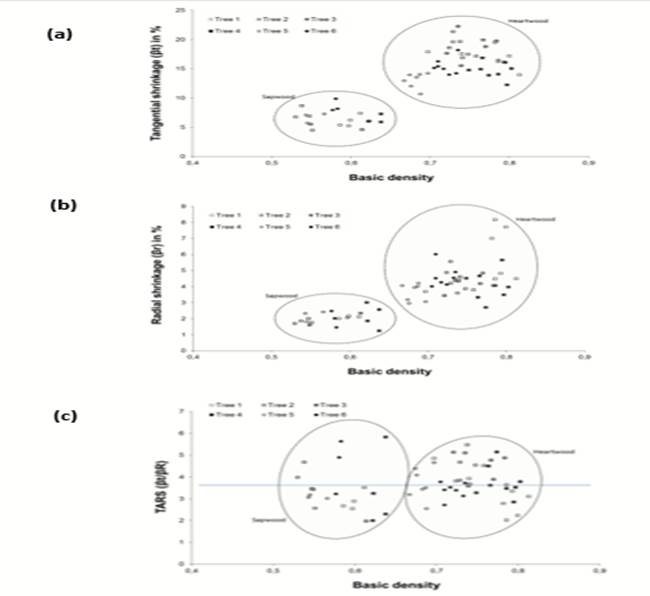
Figure 8 Relationships between basic density (Db) and (a) wood tangential shrinkage [βt], (b) radial shrinkage [βr] and (c) the TARS of Q. canariensis wood, according to the various selected trees.
Strong positive correlations were found previously, between densities, radial and tangential shrinkage and percentage of cell wall (Sadegh, Kiaei, & Samariha, 2012). These results could be explained by the fact that wood shrinkage and swelling are affected by several wood factors, such as the heartwood to sapwood ratio or the fibrillary angle on the S2 layer (Zheng, Pan, Zhang, Jenkins, & Blunk, 2006). Wood density is an important property for both solid wood and fiber products from conifers and hardwoods, and is affected by cell wall thickness, wood ray proportion, cell diameter, earlywood to latewood ratio and chemical content of the wood (Orwa, Mutua, Kindt, Jamnadass, & Anthony, 2009).
Finally, shrinkage anisotropy, which is characterized by the TARS (Fig. 8 c), was found to be homogeneous within the trees and high with a mean ratio of 3.520 between tangential and radial shrinkage. This ratio differs only slightly from sapwood to heartwood whereas the βt - βr difference appears significantly higher in heartwood. High difference between βt and βr shrinkage values indicates that this wood will be more prone to distortion and cracks during drying.
Influence of basic density (Db) on wood shrinkage inter wood species
Figure 9 highlights the correlations between wood basic density and (a) tangential shrinkage, (b) radial shrinkage, and (c) the TARS, for the four hardwood species. It appears that radial and tangential shrinkage is correlated with wood basic density according to three density classes as shown in figure 9 (a, b) : [Z1] Db < 0.550; [Z2] 0.550 < Db < 0.650; [Z3] Db > 0.650. Z1 was mainly composed of C. sativa wood specimens and no correlation between shrinkage and density was observed for this species. Z2, however, was made up of all wood species but mainly Q. robur and Q. petraea woods and a slight positive relation was observed between the density of the wood and its respective shrinkage properties. Finally, Q. canariensis wood specimens were in Z3 with a high positive correlation between density and shrinkage values. These results from Q. canariensis are consistent with others previous studies highlighting that wood density are related to tangential and radial shrinkage, according to the wood species (Harris & Meylan, 1965; Pentoney, 1953).
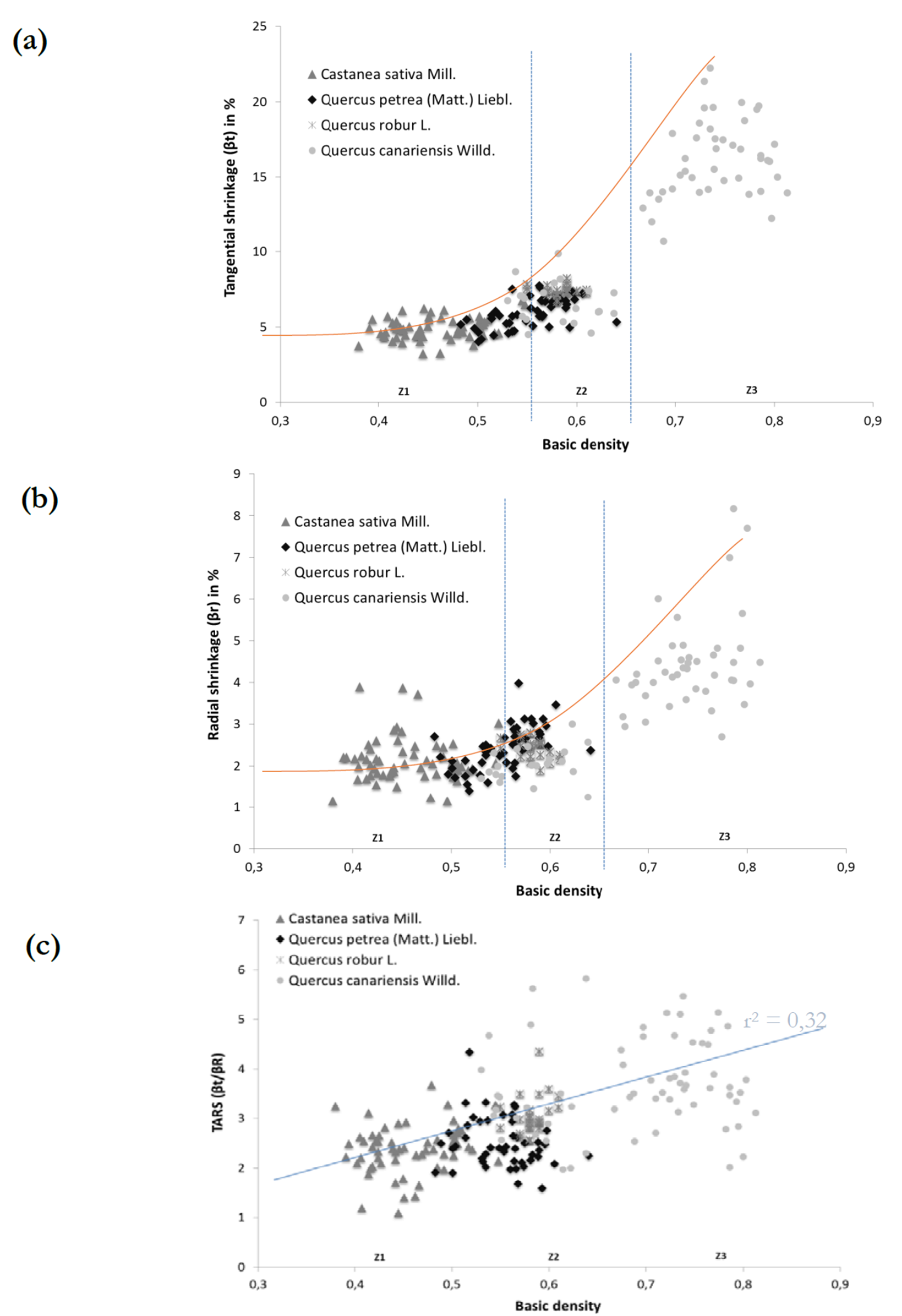
Figure 9 Relationships between heartwood and sapwood basic densities (Db) and (a) wood tangential shrinkage [βt], (b) radial shrinkage [βr] and (c) the TARS, for the four ring-porous hardwood species.
Contrary to the previous no-variation TARS values recorded according to the evolution of wood density for the same species (Fig. 8c), Figure 9c shows that TARS value increased according to wood basic density depending on the wood species (r2= 0.320). This last observation is especially due to the different anatomical structure (Fig. 1) from the various wood species and not due to their respective basic densities.
These statements confirm the effect of anatomical structure, and more particularly the influence of wood-ray proportions and characteristics, on wood density and its shrinkage properties, as it was established earlier for Mothe, Chanson, Thibaut, Martin, & Mourgues (1990) who confirmed that taking simple anatomical data into account, such as ring properties or the number of wood-rays, in addition to density, generally improves wood shrinkage predictions. Similar results were found by De la Paz, et al (2005).
Influence of multiseriate wood ray proportion on wood shrinkage
In recent years, the influence of rays on the wood properties has not been studied too much, although it is an important component of the anatomical structure of wood, and it is difficult to find scientific information on this subject after the 1990’s. This lack of interest is surprising since one-fourth to one-third of the total xylem of some species is composed of these tissues (Taylor, 1969). The results obtained on wood ray proportion are consistent with those studied in the past. Microvideomat de Zeiss analyses carried out in this work showed that Q. canariensis and Q. petraea wood specimens contain multiseriate-ray proportions from 4.430% to 38.45% [Average value = 18.41%], and from 1.590% to 15.19% [Average value = 6.56%], respectively. As shown on figure 1, C. sativa wood contains no multiseriate wood rays and that the multiseriate wood ray proportion of Q. robur wood was very similar to that of Q. petraea wood. For this reason, the discussion will focus only on Q. canariensis and Q. petraea wood properties. Figure 10 shows correlations between Q. canariensis, and Q. petraea showing large ray proportion of the wood and their respective tangential shrinkage, radial shrinkage and TARS values. It can be observed a tendency showing that wood ray proportion is related to wood shrinkage properties. These results are similar to previous findings by Mattheck and Kubler (1997) and Kawamura (1979) who determined relationships between wood-ray proportion, strength properties and shrinkage values. These correlations could be explained by the wood anatomical structure and its chemical composition. Variations of shrinkage in different directions are due to several factors such as the cellular structure and physical organization of cellulose chain molecules within the cell walls (Ilic, Boland, McDonald, Downes, & Blakemore,, 2000).The microfibril angle of the S2 layer is also an important factor (Ilic et al., 2000), and the presence of a gelatinous layer in the fibers results in large shrinkage (Malan & Arbuthnot, 1995). Finally, they may be due to ray proportion as well, which are significant anatomical elements of wood, since their volume can represent from 3% to 30 % of the total volume of the wood (Barbe & Keller, 1996). In fact, the number of rays per centimeter (in the tangential plane) is a good indicator of the tangential and radial shrinkage of wood. In addition, Kawamura (1982) highlighted that the volume fraction and the type of woody rays contain in the wood are correlated to the wood mechanical properties. Similar results are shown by De la Paz et al. (2005). In particularly, the volume fraction of woody rays would be a great parameter allowing a better estimation of the mechanical Moduli of elasticity of wood (Guitard & El Amri, 1987).
Conclusions
This work focused on some physical properties (basic density, shrinkage) and wood-ray characteristics of four ring-porous hardwoods with some anatomical features, according to their wood ray composition: Castanea sativa, Quercus petraea, Quercus robur and Quercus canariensis. The four wood species showed different proportions of large and multiseriate wood-rays. According to their wood anatomical properties, wood-ray proportion seems to be positively correlated with wood basic density, radial shrinkage, tangential shrinkage and Transverse Anisotropy Ratio for Shrinkage (TARS). The results show that the latter wood properties vary according to the wood position within the tree. A significant difference was observed between heartwood and sapwood specimens. Sapwood specimens have low tangential and radial shrinkage values whereas heartwood specimens are characterized by higher deformations in both directions.
To conclude, tangential ray proportion is a good indicator of tangential and radial shrinkage of wood. Considering the volume fraction of woody rays contained within the wood would be a suitable factor to improve predictions of its physical properties in order to choose the best species adapted for the intended use.











 nueva página del texto (beta)
nueva página del texto (beta)







