von Kármán’s mean velocity distribution model

Different models to represent velocity distribution in an open channel flow are found in the literature (^{Spalding, 1961}); however, in the practice of hydraulic engineering, the most widely used model is that of von Kármán, which has three conditions. The first is to represent the viscous or laminar sublayer where the turbulent stresses have no influence and the viscous forces (v) dominate (Equation 3).

The second represents the transition zone, also called the buffer zone, where the turbulent and viscous stresses are of the same order (^{Shih, Povinelli, Liu, Potapczuk, & Lumley, 1999}) (Equation 4).

The third considers the outer zone or turbulent region where the viscous stresses have no influence (Equation 5). This condition is known as the log-law (^{George, 2007}).

The log-law has two empirical variables: the von Kármán constant (κ) and the additive constant (A). According to ^{Zanoun et al. (2003)}, the values of 0.4 for κ and 5.5 for A, parameters used in practice for an open channel flow, are considered adequate (Equation 6).

Each of the equations comprising the von Kármán model has a validity range as a function of the parameter yu _* /v and indicates the evaluation zone of the three flow regions: viscous, transition and outer sublayer.

Shear velocity estimation

For the experimental study it is important to know the boundaries of the outer region (Equation 6). According to the experimental results of ^{Tominaga and Nezu (1992)}, the point closest to the bottom in mean velocity flows in the vertical u-m less than 40 cm·s^-1 must be deeper than 3 mm (y > 3 mm) and in the case of velocity flows u-m greater than 40 cm·s^-1 it is permissible to consider measuring points 1 mm from the bottom (y ≥ 1 mm).

Equation 6 uses the estimate of the averaged flow velocity u-; however, in this case, the estimation of the instantaneous shear velocity (u) was done with separation of velocity scales, one is the averaged and the other the fluctuating u=u-+u'. The measuring equipment used for this case was an acoustic Doppler velocity meter (ADVM) with a maximum sampling frequency of 100 Hz.

In order to process the experimental data, the velocity measurement pertaining to the outer zone was assessed to discard the points outside the range of analysis. Subsequently, the shear value was estimated with the instantaneous velocity (ui,j) using Equation (7).

The subscript i indicates the analysis point in the velocity profile and j the temporal sample number. To be able to include the high frequency, the mean velocity u- was replaced by the instantaneous velocity ui,j.

Equation 7 is not explicit since the shear velocity is found on both sides of the expression; therefore, for its solution, the fixed-point algorithm described by ^{Burden and Faires (2011)} was applied.

According to ^{Schmid and Lazos-Martínez (2000)}, in the measurement of a random variable, the results generally follow, in good approximation, a normal distribution. In the case of flow velocity, there are results that validate this hypothesis to a certain degree. This subject is widely discussed by ^{Frisch (1995)} and ^{Davidson (2004)}; however, in this case, the probability density function (PDF) of the data obtained by evaluating Equation 7 is unknown. Therefore, the final value representative of the shear velocity u _* was calculated with the median of the u _*i,j values obtained.

Anderson-Darling test of the shear model

As part of the data analysis, the Anderson-Darling normality test was performed to determine if the data follow a normal distribution, this by calculating the statistical value of the test (Equation 8).

According to ^{Stephens (1974)}, the critical value is 0.754 for a significance level of 5 % and when the number of data (n) is greater than 100. Therefore, if the statistical value A ² , of the test for the sample, is less than 0.754, it is accepted that the data follow a normal distribution; otherwise, the possibility that the data follow a central tendency distribution is rejected.

Due to the fact the PDF was unknown, and in order to ensure 50 % coverage of the data around the representative value of u _* , the location of the first (Q ₁ ) and third (Q ₃ ) quartile of the data ordered in increasing form was calculated (Equations 9 and 10, respectively).

From the location of the first and third quartiles the u _*i,j values were located and the interquartile range was obtained.

Experimental station

The tests were carried out in the variable slope experimental channel at the Hydraulic Laboratory of the Mexican Institute of Water Technology (IMTA, for its Spanish acronym); the channel has a smooth, metallic, rectangular-shaped bottom with a 0.245-m-wide base and 5 m long (Figure 1). The experimental station has a 10-hp pump that supplies the flow, a measuring weir calibrated with ISO standard 1438 (^{International Organization for Standardization, 2008}), and a valve to regulate the flow, among other components that allow for stable experimental conditions.

Figure 1 Experimental station. 

For measuring the instantaneous velocities, the Nortek^® Vectrino Profiler™ ADV device was used; it allows sampling the three velocity components in a profile of up to 30 mm, with a separation of 1 mm between cells and with a sampling frequency of 1-100 Hz. The device was positioned 3.5 m away from the flow inlet to avoid defects in the velocity profile caused by the inflow of the flow or by its outflow in free fall to the recirculation tank.

The channel slope was slightly modified with a mechanical jack, taking care that the experimental conditions complied with the criteria of repeatability and reproducibility. The Froude (F _r ) and Reynolds (R _e ) numbers were calculated with Equations 11 and 12, respectively.

All the tests were done in a subcritical regime, as indicated by the Froude number values in Table 1 (F _r < 1). In addition, the flow was considered fully developed turbulent, with the Reynolds number values being sufficiently high and far from the transition range (R _e > 1.2 x 10⁴).

For all tests, the measurement of the instantaneous velocities was carried out in a 12-mm profile, as close as possible to the wall, with a frequency of 100 Hz and a sampling time of 30 s.

Tests

Figure 2 shows the results of a sampling of the instantaneous velocity with F _r = 0.67 and R _e = 4.22 x 10⁴ for cell five at 8.6 mm depth.

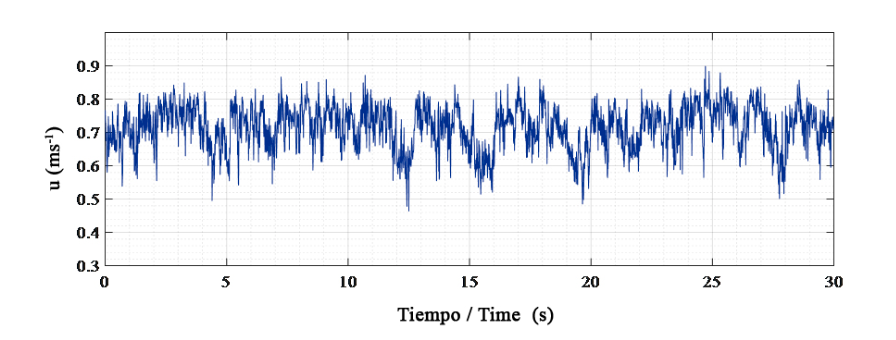
Figure 2 Record of instantaneous velocity (u) (main direction, x) taken at the Hydraulic Laboratory of the Mexican Institute of Water Technology (IMTA). 

The tests were conducted under the experimental conditions shown in Table 1.

The results of the processing of the experimental data are presented in Table 2, where the statistical value obtained from the Anderson-Darling test with a 5 % degree of significance and the shear velocity obtained with the model of Equation 7 are indicated.

The statistical values of the Anderson-Darling test (Table 2) corroborate that the PDF of the data u _*i,j does not follow a central tendency, since in all cases A ² > 0.754; therefore, it is correct to represent the shear velocity value u* with the statistical median and make use of the interquartile range to obtain 50 % coverage around the representative value.

Figure 3 shows four dimensionless profiles drawn from the estimation of the shear velocity with a different bottom slope. It can be seen that the von Kármán model represents, with good approximation, the experimental values in their averaged condition.

Figure 3 Dimensionless profiles of mean velocities, the von Kármán model and experimental values. 

In the profiles of tests P-01, P-05 and P-09, a sampling point is observed in the transition zone; this case was discarded from the data analysis since the logarithmic flow model is only for the outer region.