1. Introduction

Similar to the electron, its anti-particle (the positron) also has important scientific and technological applications in a large variety of fields. A thorough presentation of the many applications of positrons can be found, for instance, in the book by Charlton and Humberston¹. These include astrophysics, solar physics, bio-medicine (both diagnostics and therapy) and materials science (defect studies and crystallography). From a more fundamental perspective, positrons are essential in the formation of antihydrogen, understanding elementary particle and positronium (Ps) physics, as well as in the investigation of positron binding to ordinary matter, i.e. neutral atoms and molecules. Resonances in electron-impact on atoms and molecules are well-known however, the situation with respect to positrons is not as clear¹. Positron binding energies have been measured for a large variety of small and large molecules², although only a few calculations are available. On the other hand, positron-atom binding has long been predicted for many atomic targets³, but it has not been observed yet. Recent advances both in theory and in experiment in positron physics had a great impact in several areas of research and knowledge (measurements of integral cross-sections are now being published by several groups⁴. These new scattering measurements are very important for comparison, setting new standards for both theoreticians and experimentalists. Indeed one rationale for the present investigation is to try and shed more light on this state of affairs. Our work represent a study preliminary on the simple scaling of plane wave Born cross section which was adapted for positron-impact excitations of atoms and molecules⁵. This study represent a investigation of the procedure which was proposed by Kim for electron scattering by atoms⁶. Here we have proposed a new model for positron-impact scattering which was developed by combining the original form of the electron scattering with the positron scattering theory.

Validating a scaling method for Born (first Born Approximation-FBA) cross sections of atoms (molecules) requires two initial ingredients: (i) the Born integral cross sections (ICS) themselves; (ii) reliable experimental or theoretical optical oscillator strengths. Although the model presented by Kim⁶ can generate quite accurate integral cross sections which compare well with experimental data the model has some inherent limitations owing to its relatively simple origin. The cross sections using FBA for simple atoms or molecules have been calculated since computers became powerful enough to perform such calculations, but it is now rare to find a paper on Born cross sections for electronic excitations of targets. This therefore represents a possible impediment in the implementation of the scaling Born approach?. Some studies using the scaling Born approach for electron scattering illustrates that the method could never hope to supplant ab initio scattering calculations, it simply cannot provide a mechanistic description of the scattering process. Nevertheless where such calculations are impractical or unavailable at this time, the scaling Born procedure does offer a very useful alternative. Cross sections for positron and electron impact are virtually identical at high energies and if the basic dynamical ingredients for this evidence is the FBA, then is possible extend the analysis developed by Kim⁶ to more complicated systems as positron-molecule (this is a important consideration and can be significant for studies using positron as incident particle). One of the complications created by the use of positron as incident particle is the existence of additional positronium channels which are not present in the case of electron scattering. Thus, we will present a study of the possibility of forming the positronium (Ps) compound, that adds a distinct ’reactive’ channel to the scaling Born approach. The goal of the present scaling method is to provide a simple theoretical method to calculate excitation cross sections comparable not only to reliable experimental data, but also to more sophisticated theories such, for example, Schwinger multichannel (SMC) method⁷.

In Sec. 2 we identify changes necessary to transform the model proposed by Kim⁶ and the present model for positron scattering. In Sec. 3 we discuss the application of the method for excitation of H 2 ( 𝑋 1 𝛴 𝑔 + [0cm][0cm]-> 𝐵 1 𝛴 𝑢 + , 𝐶 1 𝛱 𝑢 ) states. Conclusions are presented in Sec. 4.

2. Theory

The FBA is used as the starting point in the present work because (a) plane wave is the correct wave function at infinity for an positron (or electron) colliding with a neutral atom (or molecule), and (b) it is the simplest collision theory that uses target wave functions explicitly. The explicit use of target wave functions enables one to use relativistic wave functions for heavy atoms, and to distinguish the final state of the target. The scaling Born approximation described by Kim [6] for excitation of neutral atoms and molecules is applicable to dipole-allowed excitations, and use molecule properties as excitation energy, ionization energy, and the dipole 𝑓 value that can be obtained, in principle, from accurate wave functions. Since scaled cross sections are based on the plane wave Born approximation, they do not account for the resonances often found near the excitation thresholds. Qualitatively the FBA does not account for the electron scattering exchange effect with the target electrons, the distortion of plane waves in the vicinity of the target molecular, or the polarization of the target due to the presence of the incident particle. The scaling method developed by Kim⁶ combine these deficiencies into simple functional forms that depend on a few atomic or molecular properties. The scaling method proposed here for positron scattering is similar to the scaling method of Kim⁶ where the positronium channel now is introduced. We will see that our method not only reduces the cross sections magnitude at low energy, but also shifts the peak to a high energy than the peak of the unscaled FBA, while keeping the high energy validity of the Born approximation intact. In a generic form, first order Born cross sections 𝜎 Born (𝐸) for inelastic collisions are written as⁶

where 𝑎 0 is the Bohr radius, 𝑅 is the Rydberg energy, 𝐸 is the incident electron (positron) energy, and 𝐹 Born (𝐸) is the collision strength (multiplied by a constant to be consistent with the standard definition of the collision strength). The scaling method apply only to integrated excitation cross sections, not to angular distributions, because the scaling method do not alter the angular distribution shape described by the unscaled Born cross sections. The modified scaling here proposed, to be referred to as 𝐸 𝑝 𝐸𝑓-scaling (when use 𝑓) and 𝐸 𝑝 𝐸-scaling (without 𝑓) replaces the 𝐸 that appears in the denominator, i.e., 𝐸 by 𝐸+ 𝐸 ps + 𝐸 exc , where 𝐸 ps is the positronium energy, and 𝐸 exc is the excitation energy. The 𝐸 𝑝 𝐸-scaling initial is introduced as

with the argument that the effective kinetic energy of the incident positron seen by the target is 𝐸 plus the energy of the bound electron. The 𝑓-scaled Born cross sections,

A hint to the meaning of adding 𝐸 ps + 𝐸 exc to 𝐸 may be found in FBA cross section for the elastic scattering from the Yukawa potential. The potential is a screened Coulomb potential

where 𝑟 is the radial coordinate, 𝑍 is the atomic number, and 𝛽 is the “range” of interaction with a dimension of length. Using plane waves for the incident electron (or positron), the integrated cross section for elastic scattering is

where 𝑘 is the momentum of the incident electron (positron) in atomic units. After writing 𝛽=𝑏 and noting that𝑘=𝐸/𝑅 (Rydberg), Eq. (5) becomes

which has the 𝐸 in the denominator shifted by a constant with the dimension of energy. Although this analogy is not rigorous, the similarity between the Eq. (2) and Eq. (6) suggests that the constant ( ?? ps + 𝐸 exc ) can be seen as the scaling factor to represent the correlation between the positron and electron of the target (the combination 𝐸 ps + 𝐸 exc should not be taken literally as a rigid rule, but only as an indicator of the order of magnitude for the cross section). At present, the 𝐸 𝑝 𝐸𝑓-scaling cannot be “derived” from first principles (as cited by Kim for electron scattering⁶). As a test to study the scaling Born cross sections for positron scattering we have investigated some cases; electronic excitation of the H 2 ( 𝑋 1 𝛴 𝑔 + [0cm][0cm]-> 𝐵 1 𝛴 𝑢 + , 𝑋 1 𝛴 𝑔 + [0cm][0cm]-> 𝐶 1 𝛴 𝑢 + ) states.

The present study help verify also the influence of basis set used for the initial and final states of the H 2 molecule. The accuracy of the wavefunctions used in scattering theory is imperative and a study )using the Schwinger multichannel (SMC) method⁷ for H 2 showed that a serious difficulty with the trial scattering basis set, same for Born approximation its can be observed. Our idea was to study also how the basis set used in the Born approximation is significative and we report a technique where two different quadratures for 𝑘 𝑖 and 𝑘 𝑓 to avoid situations where | 𝑘 𝑓 − 𝑘 𝑖 | is too small. The evaluation for 𝑘 𝑖 (or 𝑘 𝑓 ) is generally associated with a double quadrature mesh ( 𝜃 𝑖 , 𝜙 𝑖 ) and ( 𝜃 𝑓 , 𝜙 𝑓 ) and with this process is expected to have a more significant convergence numerical. For the 𝐸 𝑝 𝐸𝑓-scaling presented we have used some considerations about the Born cross sections. To define a good Cartesian Gaussian basis set to produce a target molecule is not an easy task and the experience gained in ab initio calculations for electron-molecule collisions can be useful⁷. As pointed by Arretche and Lima⁷, no clear rules or procedures to construct trial basis sets for targets or scattering calculations. The ideia is to work with an initial set of functions { 𝐺 𝑘 } large enough such that the completeness of the basis set comes from “saturation”:

Scattering amplitudes obtained from Born method are valid for high-energy static calculations, i.e., in situations where the target wave functions can be considered frozen. As we will see our scaling Born for positron not only reduces the cross section magnitude at low energy, but also shifts the peak to a higher energy than the peak of unscaled (FBA), while keeping the high energy validity of the FBA approximation intact. Although computational tools are available to generate wave functions that will produce accurate electric dipole oscillator strengths, or the 𝑓 values, they are not always easy to use. Since 𝐸 𝑝 𝐸𝑓-scaling is just an adaptation of the FBA, the cross section obtained gives a direct and unequivocal measure of the quality of the present model.

3. Results

Considering the positron impact excitation-H 2 ( 𝑋 1 𝛴 𝑔 + [0cm][0cm]-> 𝐵 1 𝛴 𝑢 + ), we illustrate in Fig. 1 the integral cross sections (ICS) using our 𝐸 𝑝 𝐸𝑓-scaling compared with the SMC method⁷ and experimental data⁸. The values 0.3212 and 0.310 of 𝑓 ( 𝑓 Born and 𝑓 accur ) were used in the process for H 2 . The values 12.74 eV and 13.12 eV were used for 𝐵 1 𝛴 𝑢 + and 𝐶 1 𝜋, respectively, as energy excitation and 𝐸 ps =8.5 eV.

As observed in Fig. 1 the 𝐸 𝑝 𝐸𝑓-scaling is comparable in shape with the sophisticated Schwinger multichannel(SMC) method⁷. A cited by Arretche and Lima⁷ a serious difficulty with the trial scattering basis set was identified using

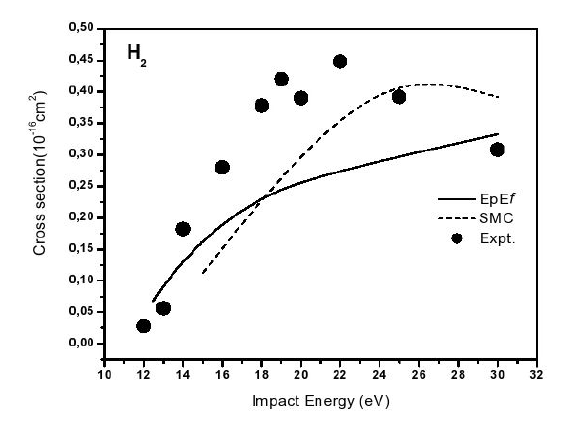
Figure 1 Electronic excitation cross sections for H2 (X1§+g-> B1§+u ) by positron impact in units of 10¡16 cm2. Solid line: EpEf-scaling; dashed line: SMC method [7]; black circle: experimental data⁸. 

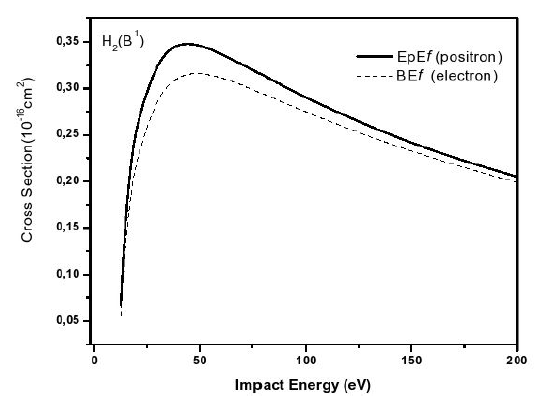
Figure 2 Electronic excitation cross sections for H2 (X1§+g -> B1§+u ) in units of 10¡16 cm2. Solid line: EpEf-scaling; dashed line: BEf-scaling for electron scattering. 

the SMC method and a systematic set of procedures to verify the quality of the basis set was considered⁷. This is important to point out that this level of similarity between our 𝐸 𝑝 𝐸𝑓-scaling and SMC method is of special relevance. The excitation electronic for 𝐵 1 𝛴 𝑢 + state using 𝐸 𝑝 𝐸𝑓-scaling and the scaling Born for electron scattering used by Kim⁶ is shown in Fig. 2. Analysis of the figure shows good agreement between the two methods at high energies. The opposite sign of the static interaction for positrons causes a kind of compensation between the two parts of potential (static + polarization): small adjustments of these parts cause big differences in cross sections. In the high energy region, the polarization, changing with the impact velocity, becomes relatively weaker. For high energy (same for inelastic process), Born’s approximation predicts equal cross sections for positrons and electrons and the convergence of the two cross sections for H 2 in the high energy can be observed (the convergence of the two cross sections for several atoms and

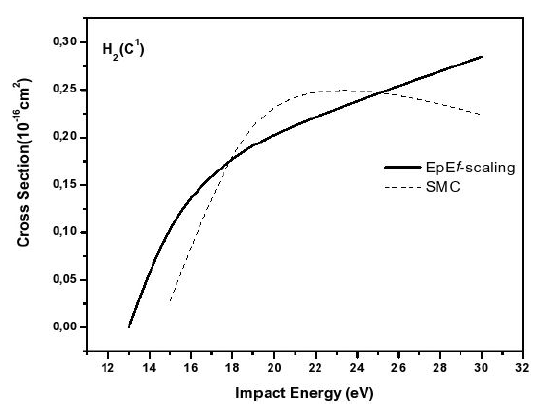
Figure 3 Electronic excitation cross sections for H2 (X1§+g-> B1§+u ) by positron impact (units of 10¡16 cm2). Solid line: EpEf-scaling; dashed line: SMC method⁷. 

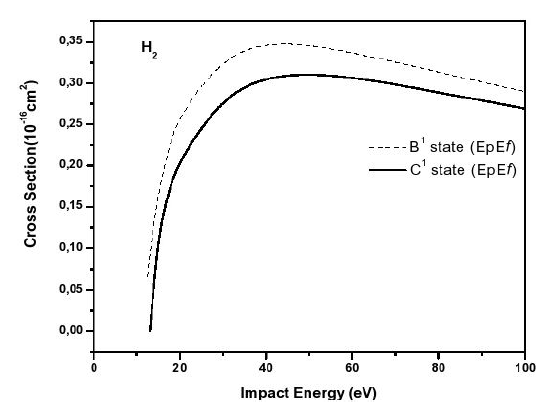
Figure 4 Electronic excitation cross sections for H2 (X1§+g -> B1§+u , X1§+g -> C1§+u ) by positron impact using EpEf- scaling only (units of 10¡16 cm2). Solid line: C1§+u ; dashed line: B1§+u. 

molecules in the high energy limit still represent a question open).

The results for the H 2 ( 𝑋 1 𝛴 𝑔 + [0cm][0cm]-> 𝐶 1 𝛴 𝑢 + ) transition are shown in Fig. 3. Again, we see reasonable agreement with the sophisticated SMC method⁷.

In Fig. 4 we shown electronic excitation cross section for H 2 ( 𝑋 1 𝛴 𝑔 + [0cm][0cm]-> 𝐵 1 𝛴 𝑢 + , 𝑋 1 𝛴 𝑔 + [0cm][0cm]-> 𝐶 1 𝛴 𝑢 + ) using 𝐸 𝑝 𝐸𝑓-scaling. This figure indicates that the cross section associated with the 𝑋 1 𝛴 𝑔 + [0cm][0cm]-> 𝐶 1 𝛴 𝑢 + transition is similar to the 𝑋 1 𝛴 𝑔 + [0cm][0cm]-> 𝐵 1 𝛴 𝑢 + one and this similarity indicates a nontrivial results (the present results also was observed by Arretche and Lima using the SMC method⁷ and this can motivate experiments). The present results indicate that the 𝐸 𝑝 𝐸𝑓-scaling can be extremely relevant to calculate electronic excitation cross sections for H₂⁹.

4. Conclusions

The Born-scaling for electron scattering by atoms and molecule have been extended to positron scattering. The results presented in this paper demonstrate the utility of the concept of adding a constant to the incident positron energy 𝐸 in the denominator of Eq. (1) as a simple way to obtain positron-impact cross sections for H 2 . The results of the 𝐸 𝑝 𝐸𝑓-scaling shown for H 2 should not diminish the value of more sophisticated methods that produce highly accurate results, though they require orders of magnitude more computational effort than FBA cross sections. First of all, new studies and reliable results are needed to confirm that 𝐸 𝑝 𝐸𝑓-scaling is reliable for positron scattering. Second, the 𝐸 𝑝 𝐸𝑓-scaling will not work well on angular distributions because the scaling only changes the “𝐸” dependence of FBA cross sections. It is well knows that FBA cross sections not reproduce large angle scattering well at any “𝐸”, and the 𝐸 𝑝 𝐸𝑓-scaling will not change the shape of FBA angular distributions. The scaling described here for positron scattering will facilite the calculation of integrated excitation cross sections for targets in general, which pose difficulties to more computer intensive theories.