2.1.Static potential

To evaluate the static potential, we use the ammonia molecular orbital functions determined by Moccia ³⁵. In this description, each molecular orbital was expressed in terms of Slater-type functions all centered at a common origin (the heaviest atom). Thus, the ammonia target can be described by means of Norb(Norb=5) molecular functions labeled 1A1, 2A1, 1A3, 1Ex and 1Ey, written as ³⁷

where N _j is the number of Slater functions used to construct the jth (with j varying from 1 to Norb) molecular orbital Ψj(r); r^ refers to the solid angle of the position vector r, and ajk the weight of each real atomic component. The function Rnjk,ljkξik(r) represents the radial part expressed by Slater-type functions (38) as

and the angular part Sljk,mjk(r^) are the real spherical harmonics linked to the complex form Yljk,mjk(r^) by ³⁹

For more details, we refer the reader to Refs. 35 and 37 where all the coefficients (ajk,ξjk) and all quantum numbers (njk,ljk,mjk) used are reported. Besides note that the static potential includes electronic and ionic contributions

The electronic contribution is given for each molecular orbital j by

and the ionic one by

The symbols r> and R> are respectively given by r>=max{r,r'}, and R>=max{r,RNH} where RNH=1.928 a.u. ³⁵ is the internuclear distance between the two atoms N and H, and Z = 10 for ammonia molecule.

2.2.Correlation-polarization potential

It is well established that NH₃ is polar covalent moleculedue to the electronegativity difference between the nitrogen (higher) and the hydrogen atoms. Hence, e-NH₃ interactions induce an additive potential due to the polarizability of the target induced by the incident electrical charge particularly at low velocities. The polarization potential Vp(r) used here refers to the well-known Buckingham type given by

where αd is the polarizability (αd=14.984 a.u. for NH₃⁴⁰) and rc is a cut-off-parameter expressed by Mittleman and Watson ⁴¹ as

with bpol an adjustable parameter. In our case, we found that (see Salvat et al. for more details ⁴²)

where E refers to the incident electron energy.

As proposed by Salvat et al. ⁴², we use here the polarization potential combined with the correlation one, called correlation-polarization potential. Different expressions of the correlation potential are found in the literature; the first expression chosen here is given by Padial and Norcross (1981) ⁴³

and the second one is given by Carr and Maradudin (1964) ⁴⁴

where rs=(3/4πρ(r))1/3) and β0=0.1423, β1=1.0529 and β2=0.3334. Following Salvat et al. ⁴² the correlation-polarization potential can be given by

where rcr is defined as the outer radius where the two potetials Vp(r) and Vc(r) cross.

2.3.Exchange potential

In addition to the polarization phenomena of the target in the electron-molecule interactions, the incident electron can be captured by the target - particularly at low incident energies - and then the latter releases one of their bound electrons. This phenomenon known as electron exchange process needs another potential called exchange potential. Among the various formulas available in the literature, we have selected two expressions; the first one Vex1(r), depending clearly on the static potential and the correlation-polarization one, was given by Furness and McCarty ⁴⁵

where ρ(r) is the spherical density of the electronic charge given by ⁴⁵

The second exchange potential Vex2(r), used here, was initially given by Mittleman and Watson ⁴⁶ and re-written by Riley and Truhlar ⁴⁷:

where kL is the local wave number of the electron projectile defined by kL2=k2+2I++kF2,I ⁺ being the first ionization potential of the molecule target, kF(r)=(3π2ρ(r))1/3 the Fermi wave number of the atomic electron cloud, and k2=2E. For illustration, we have reported in Fig. 1 the various potentials used in this work and described above. In Fig. 1(a), the static potential - calculated according to Eqs. (6), (7) and (8) - decreasing rapidly up to large distances present a discontinuity around =1.93 a.u due to the presence of the proton shell in the NH₃ molecule where the internuclear distance RNH=1.928 a.u. In panel (b), the two correlation-polarization potentials Vcp1(r) and Vcp2(r) are compared, we clearly observe for r<rcr a quasi-linear behavior with the radius whereas they both mimic the asymptotic Vp(r) potential and then depend on the projectile energy at large distances. The two exchange potentials Vex1(r) and Vex2(r) are reported in Fig. 1(c), the first one presents no minimum contrary to Vex2(r) which exhibits clearly a minimum for r = 0.07 a.u. However, they have nearly the same values at large distances r.

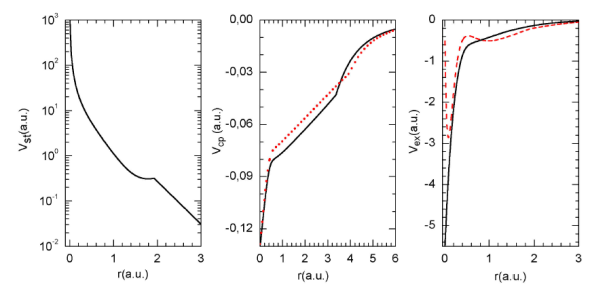
FIGURE 1 (Color online). Different potentials used in this work. (a) Static potentials used to describe the electron elastic scattering by ammonia molecules, (b) Correlation- polarization potentials V _cp1 ( black solid line) and V _cp2 (red dotted line), (c) Exchange potentials V _ex1 (black solid line) and V _ex2 (red dashed line). 

Finally, note that here we have studied studied two expressions for the correlation-polarization potential (Vcp1(r) and Vcp2(r)) and two others for the exchange potential (Vex1(r) and Vex2(r)). So, according to Eq. (2), we get four combinations for the potential V(r), namely Vst(r)+Vcp1(r)+Vex1(r), Vst(r)+Vcp1(r)+Vex2(r), Vst(r)+Vcp2(r)+Vex1(r), and Vst(r)+Vcp2(r)+Vex2(r) which are used in our calculation.

3.1.Differential cross sections

Differential cross sections for elastic scattering of electron by NH₃ molecule are calculated for scattering angles varying from 0 to 360^o and for numerous incident energies. First, these differential cross sections are reported in Fig. 3 (left panel) for 15 eV, 20 eV and 30 eV, where experimental data are available and only from 0 to 180^o since they are symmetrical with respect to the axis θ=180∘. The calculations are carried out for the four above-cited combinations of potentials investigated in this work. To the best of our knowledge, the only existing experimental DCSs related to the energies investigated here, were reported by Alle et al. ¹² and Shyn¹⁰ and we are not aware of the existence of other measurements.

The DCSs obtained (left panel in Fig. 3) are important in the forward directions and lesser in the backward directions, while they are even smaller exhibiting a valley around the perpendicular directions, as expected. In general, the different exchange and correlation-polarization potentials, investigated here, provide quasi-identical DCSs and a good agreement is observed throughout the angle range for all the energies investigated. However, at lower scattering angles, the calculated DCSs exhibit little discrepancies particularly at lower energies. In fact, these discrepancies related to the form of the correlation-polarization potential considered are directly linked to the polar nature of the target since there is a mutual influence between the electric dipole of the molecule and the charge of the projectile. It is then evident that this influence, responsible of the discrepancies, became relatively important at lower incident energies, since the projectile spends a relatively important time near the target molecule. In addition, the DCS curves show the existence of a profound minimum, in the exhibited valley, whose depth depends on the form of the tested correlation-polarization and exchange potentials. The minima are located around θ=109∘ at 15 eV which are shifted to lower scattering angles when the incident energy increases, namely around θ=105∘ at 20 eV and around θ=99∘ at 30 eV. Note that, outside the observed minima, our calculated DCSs are in excellent agreement with the experimental data reported by Alle et al. ¹² for vibrationally elastic scattering (black full squares in Fig. 3), using a crossed electron-molecular beam apparatus ¹². Furthermore, our results are also in good agreement with the relative measures of Shyn ¹⁰ (red circles in Fig. 3)) mentioned and normalized at 90^o by Pritchard et al. ⁹. We would note that the profound minima exhibited in these calculated DCSs for elastic scattering by NH₃ are also found in our precedent works on H₂S ⁴⁸ and HCl ⁴⁹ at lower energies using the same model. Otherwise, in Fig. 3 (right panel) the theoretical DCSs taken from ²⁹ (solid navy line), ³⁰ (dashed dark-yellow line), ²⁵ (dotted magenta line), ²³ (dashed-dotted blue line), ²⁴ (dashed dotted-dotted red line), ⁹ (short dashed black line) are also reported to compare with. Note that these DCSs do not show a profound minimum except that obtained by the Schwinger variational principle with plane waves ²⁹ (dashed dotted-dotted red line at 15 eV and 20 eV) based on fixed-nuclei approximation. As already pointed out by Brescansin et al⁵⁰, these deep minima are probably due to the fact that only the spherical part of the interaction potential is considered in the calculations. Indeed, the spherical potential used here for describing the electron elastic scattering by the NH₃ molecule is quite similar to that of the Neon atom for which the numerous DCSs reported in the literature ⁵¹ clearly point out an identical depth at lower energies. We would note here, that at intermediate and relatively high energies these very deep minima disappear completely, as we will see below. We can conclude that they are non-physical structures and probably underline the limitation of the current spherical approach for modeling the elastic scattering process at lower energies.

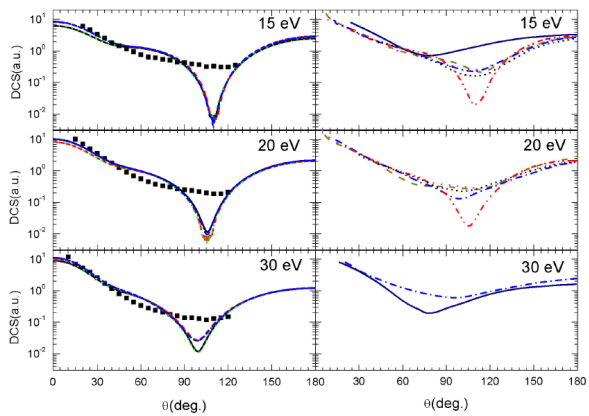
FIGURE 3 (Color online) In left panel: Comparison between calculated DCSs for electron elastic scattering by ammonia molecule at 15, 20, and 30 eV for various potentials: V _st (r) + V _cp1 (r) + V _ex1 (r) (solid black line), V _st (r) + V _cp1 (r) + V _ex2 (r) (dashed red line), V _st (r) + V _cp2 (r) + V _ex1 (r) (dotted green line) and V _st (r) + V _cp2 (r) + V _ex2 (r) (blue dash-dotted line). Experimental data are taken from ¹² (black solid squares) and ¹⁰ (red open circles). In the right panel: theoretical results are taken from ⁹ (short-dashed black line), ²³ (dash-dotted blue line), (24) (dash-dotted-dotted red line), ²⁵ (dotted magenta line), ²⁹ (solid navy line), and ³⁰ (dashed dark-yellow line). 

In Fig. 4, we report the DCSs for electron elastic scattering at intermediate incident energies (300 eV, 400 eV, 500 eV, and 1 keV) for the four combinations of correlation-polarization and exchange potentials investigated in this work. These latter provide quasi-identical curves, and an overall agreement is observed in the whole angle range for all the energies investigated. The effects of the fine contributions (correlation-polarization and exchange potentials) no longer appear even in the range of lower scattering angles. Besides, the observed minima at low incident energies disappeared from the value of 200 eV (not reported here). In addition, we have reported in Fig. 4 the experimental data provided by Harsbarger et al. ⁵ (black solid squares) and by Bromberg ⁶ (red open circles) for the scattering angles from 2 to 10^o. Our calculated DCSs appear slightly lesser than the experimental ones around 2^o and seem to be in good agreement beyond this scattering angle value. Finally note that, to the best of our knowledge, there are no other results neither experimental nor theoretical in larger angle range to compare with.

FIGURE 4 (Color on line) As in Fig. 3 at 300 eV, 400 eV, 500 eV and 1 keV. Experimental data are taken from ⁵ (black solid squares) and ⁶ (red open circles). 

3.2.Integral cross sections

Integral cross sections for electron elastic scattering from NH₃ molecules are also calculated using the four above-cited combinations including the static, correlation-polarization, and exchange potentials. The energy distribution for elastic scattering is obtained by integrating numerically the differential cross sections overall the scattering solid angle

The various obtained results are presented in Fig. 5a for the incident energies ranging from 10 eV to 20 keV, and for the four potential combinations investigated. As expected, the amplitude is important at lower incident energies and decreases monotonically with the increasing of the impact energy. In addition, the four calculated ICSs exhibit a good agreement at intermediate and high incident energies. In fact, the little discrepancies observed at low energies (≲30 eV) are undoubtedly due to the exchange and polarization effects since the ammonia molecule is a polar one with a dipole moment equal to 1.42 D. We can conclude here that the polarization-correlation and exchange phenomena have perceptible effects only at lower incident energies. We have also reported in Fig. 5a) the experimental data to compare with. Note that the available measurements in the literature concern essentially the total (elastic + inelastic) cross sections, see for example Refs. ^7,11,13,14. Other experimental results are available in the literature but not reported since they are out of the energy range investigated here. First, note that some disagreements are observed in the experimental results as already mentioned by different authors ^19,26. Besides, a simple comparison with our results shows that the predicted ICSs reproduce well the shape of the measures. Concerning the magnitude, the calculated ICSs with the potential combinations Vst(r)+Vcp1(r)+Vex2(r) (dashed red line), and Vst(r)+Vcp1(r)+Vex2(r) (dash-and-dotted blue line) are in better agreement with the experimental data of Alle et al. ¹² (black full squares) and those of Sueoka et al. ⁷ (magenta open circles). At high energies, all the calculated ICSs are in satisfactory agreement with the available measurements except that reported by Garcia and Manero ¹³ (black full circles). Besides, the calculated ICSs seem slightly lesser than the experimental ones in magnitude over all the energy range since the latter include the elastic and inelastic contribution (rotational and/or vibrational).

FIGURE 5 a) (Color online) Comparison between calculated ICSs for electron elastic scattering by ammonia for various potential combinations: V _st (r) + V _cp1 (r) + V _ex1 (r) (solid black line), V _st (r)+V _cp1 (r)+V _ex2 (r) (dashed red line), V _st (r)+V _cp2 (r)+ V _ex1 (r) (dotted green line) and V _st (r)+V _cp2 (r)+V _ex2 (r) (dashand-dotted blue line). Experimental data are taken from ¹² (black solid squares), ⁷ (magenta open circles), ¹¹ (blue up triangles), ¹⁴ (red stars), ¹³ (solid black circles), and ⁸ (olive diamonds). b) (Color online) As in Fig. 5a. Thin lines are theoretical results taken from ²¹ (red solid line and dark-yellow dashed dotted line), ²⁶ (dashed blue line), ²⁷ (dotted magenta line and short dashed purple line) and ³¹ (dashed-dotted-dotted pink line). 

The obtained cross sections (thick lines) using different potentials are also reported in Fig. 5b where they are compared to the theoretical results existing in the literature (thin lines) taken from ²¹ (solid red line and dashed dotted dark-yellow line), ²⁶ (dashed blue line), ²⁷ (dotted magenta line and short dashed purple line), and ³¹ (dashed dotted-dotted pink line). Note that the shapes of all the different cross sections (thin lines) are identical with that calculated in this work (thick lines). However, the magnitudes of our CSs are, in general, lesser than the former except that of Jain ²¹ (solid red line). In fact all the different previous CSs include the inelastic contribution except that of Jain ²¹ where the elastic CSs are considered in the static-exchange-polarization (SPE) model. Finally, it is worth noting that the CSs reported by Jain ²¹ including the absorption part (dashed-dotted dark yellow line) are higher in magnitude than that obtained in this work confirming in this way these arguments.