1.Introduction
The study of the fundamental or constituent blocks of matter has been for long time a fascinating field in physics. In the nineteenth century, the atom was considered to be the fundamental particle, the one from which all matter was composed. This idea was used to explain the basic structure of all elements 1.
The problem of what were considered to be fundamental particles was resolved by the quarks. Because of the heavy masses of the constituent quarks, a good description of many features of these systems can be obtained using non-relativistic models where the quark-antiquark strong interaction is described by a phenomenological potential 2. Heavy quarkonium systems have turned out to provide extremely useful probes for the deconfined state of matter because the force between a heavy quark and anti-quark is weakened due to the presence of gluons which lead to the dissociation of quarkonium bound states 3. The quarkonia with heavy quark and antiquark and their interaction are well described by the equation (SE) 4. The solution of the spectral problem for the SE with spherically symmetric potentials is of major concern in describing the spectra of quarkonia 5. Potential models offer a rather good description of the mass spectra of systems such as a bottomonium, and charmonium 6. In simulating the interaction potentials for these systems, confining-type potentials are generally used. The holding potential is the so-called Cornell potential with two terms, one of which is responsible for the Coulomb interaction of the quarks and the other corresponds to a confining term 7.
The solutions to the SE can be established only if we know the confining potential for a particular physical system. Till now, there are only a few confining potentials, like the harmonic oscillator and the hydrogen atom, for which solutions to the SE are found exactly 8.
The Hulthén potential takes the form 9
where α is the screening parameter and A0 is the potential strength which is sometimes identified with the atomic number when the potential is used for atomic phenomena (10). It is a short-range potential which is applied in many branches of physics, such as nuclear and particle physics, atomic physics, solid state physics, and chemical physics 11,12.
The Hellmann potential which is a superposition of an attraction Coulomb potential and a Yukawa potential can be expressed as 13.
where the parameters A1 and A2 denote the strength of Coulomb
and Yukawa potentials respectively, α denotes the screening parameter, and
r is the distance between two particles. These potentials have
been used to study bound state problems by many researchers 14-20. Recently, Inyang et al. 21 obtained the Klein-Gordon
equation solutions for the Yukawa potential using the Nikiforov-Uvarov (NU) method.
The energy eigenvalues were obtained both in a relativistic and non-relativistic
regime. They applied the results to calculate heavy-meson masses of charmonium
where A0, A1, and A2 are potential strength
parameters and α is the screening parameter. In other to make (3) temperature
dependent, the screening parameter is replaced with the Debye mass
m
D(T), which is temperature-dependent and vanishes at
We carry out a series expansion of the exponential terms in (4) up to order three in order to model the potential to interact in the quark-antiquark system and this yields,
We substitute (5) and (6) into (4) and obtain
where
The first term in (7) is the Coulomb potential that describes the short distance between quarks, while the second term is a linear term featuring confinement.
2.Approximate solutions of the equation with Hulthén plus Hellmann potential
The Schrödinger equation (SE) for two particles interacting via potential V(r) in three dimensional space, is given by 34
where l,
Let,
Substituting (11) into (10), we have
Transforming the coordinate of (12) we set
Differentiating (13) and simplifying we have
Substituting (13) and (14) into (12) we have
Next, we propose the following approximation scheme on the term
Let us assume that there is a characteristic radius r0 of the meson. Then
the scheme is based on the expansion of
Setting
which yields
Similarly,
By substituting (18) and (17) into (15) , we obtain
where
Comparing Eq. (19) and Eq.(A1) we obtain
We substitute (21) into (A9) and obtain
To determine k, we take the discriminant of the function under the square root, which yields
We substitute (23) into (22) and have
For a physically acceptable solution, we take the negative part of (24) which is required for bound state problems and differentiate; this yields
Substituting (21) and (25) into (A7) we have
Differentiating (26) we have
By using (A10), we obtain
and using (A11), we obtain
Equating Eqs. (28) and (29), the energy eigenvalues of (10) are given
2.1.Special cases
In this subsection, we obtain the special case by setting some parameters to zero.
1. When we set
2. When we set
3. When we set A0, we obtain the energy eigenvalue for Hellmann potential
4. When we set
The result of (34) is very consistent with the result obtained in (36) of .
To determine the wavefunction, we substitute (21) and (24) into (A4) and obtain
Integrating Eq. (35), we obtain
By substituting (21) and (24) into (A6) and integrating, we obtain
Substituting (21) and (37) into (A5) we have
The Rodrigues’ formula of the associated Laguerre polynomials is
where
Hence,
Substituting (36) and (41) into (A2) we obtain the wave function of (10) in terms of Laguerre polynomial as
where
3.Results
We calculate the mass spectra of the heavy quarkonium system such as charmonium and bottomonium that have the quark and antiquark flavor, and apply the following relation 36,37
where m is quarkonium bare mass and
3.1.Discussion of results
We calculate the mass spectra of charmonium and bottomonium for states from 1S to
1F, by using Eq. (45). The free parameters of Eq. (45) are fitted with
experimental data by solving two algebraic equations. Experimental data are
obtained from 38,39. For bottomonium
Table I Mass spectra of charmoniumin (GeV) for Hulthén plus Hellmann potential, (m c = 1:209 GeV, μ = 0:6045 GeV, A0 = -1:693 GeV, A1 = 20:654 GeV, A2 = 0:018 GeV, δ = 0:2 GeV, m D (T) = 1:52 GeV, ℏ = 1).
| State | Present work | [35] | [24] | Experiment [38,39] |
| 1S | 3.096 | 3.096 | 3.096 | 3.096 |
| 2S | 3.686 | 3.686 | 3.672 | 3.686 |
| 1P | 3.521 | 3.255 | 3.521 | 3.525 |
| 2P | 3.772 | 3.779 | 3.951 | 3.773 |
| 3S | 4.040 | 4.040 | 4.085 | 4.040 |
| 4S | 4.262 | 4.269 | 4.433 | 4.263 |
| 1D | 3.768 | 3.504 | 3.800 | 3.770 |
| 2D | 4.146 | - | - | 4.159 |
| 1F | 3.962 | - | - | - |
Table II Mass spectra of bottomonium in (GeV) for Hulthén plus Hellmann potential, (mb = 4:823 GeV, μ = 2:4115 GeV, A0 = -1:591 GeV, A1 = 9:649 GeV, A2 = 0:028 GeV, δ = 0:25 GeV, m D (T) = 1:52 GeV, ℏ = 1).
| State | Present work | [35] | [24] | Experiment [38,39] |
| 1S | 9.460 | 9.460 | 9.462 | 9.460 |
| 2S | 10.023 | 10.023 | 10.027 | 10.023 |
| 1P | 9.861 | 9.619 | 9.9630 | 9.899 |
| 2P | 10.238 | 10.114 | 10.299 | 10.260 |
| 3S | 10.355 | 10.355 | 10.361 | 10.355 |
| 4S | 10.579 | 10.567 | 10.624 | 10.580 |
| 1D | 10.143 | 9.864 | 10.209 | 10.164 |
| 2D | 10.306 | - | - | - |
| 1F | 10.209 | - | - | - |
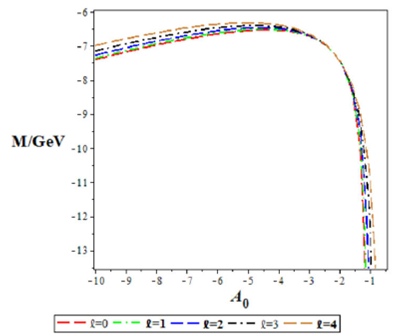
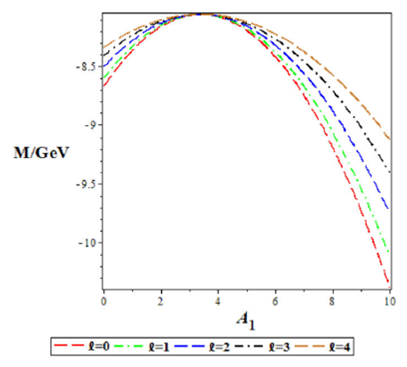
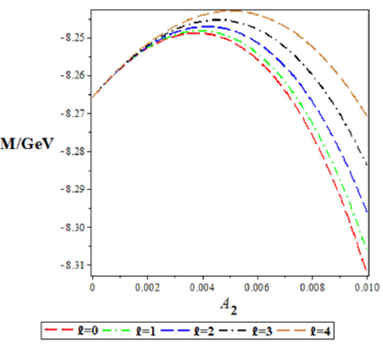
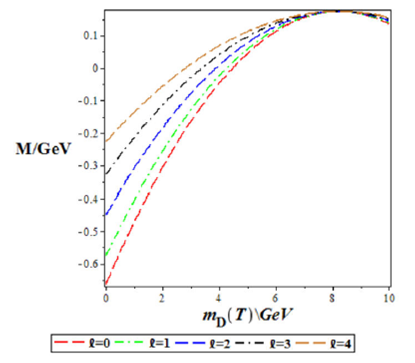
We also plotted the mass spectra energy as a function of potential parameters and Debye mass. In Figs. 1 and 2, the mass spectra energies increases to a peak and later decreases as potential parameters and increases, respectively. In Fig. 3 the mass spectra converges at the beginning, but spreads out and decrease monotonically with the increase in potential parameter A2. Figure 4 show the increase in mass spectra as the Debye mass increases, for various angular quantum numbers.
4.Conclusion
In this study, we adopted Hulthén plus Hellmann potential models for quark-antiquark
interaction. The potential was made to be temperature dependent by replacing the
screening parameter with Debye mass which vanishes at. The Schrödinger equation is
analytically solved using the Nikiforov-Uvarov method. We obtained approximate
solutions of the eigenvalues and eigenfunction in terms of Laguerre polynomials. We
applied the present results to calculate heavy-meson masses such as charmonium











 nueva página del texto (beta)
nueva página del texto (beta)